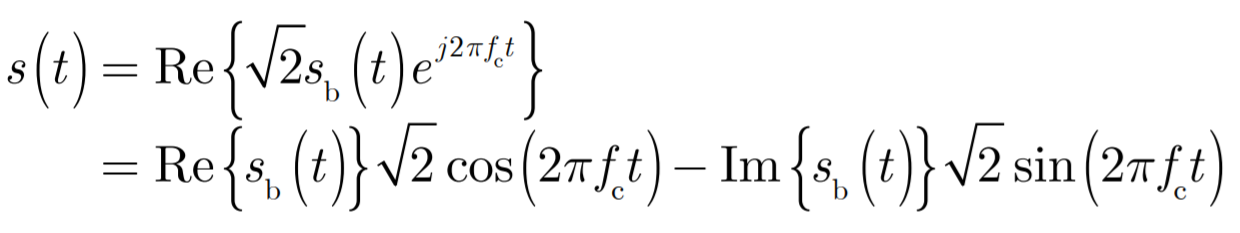Equivalent baseband communication channel

Question

I was reading on my lecture slides (I do not put them here since they are not in English) this statement about equivalent baseband channel models:

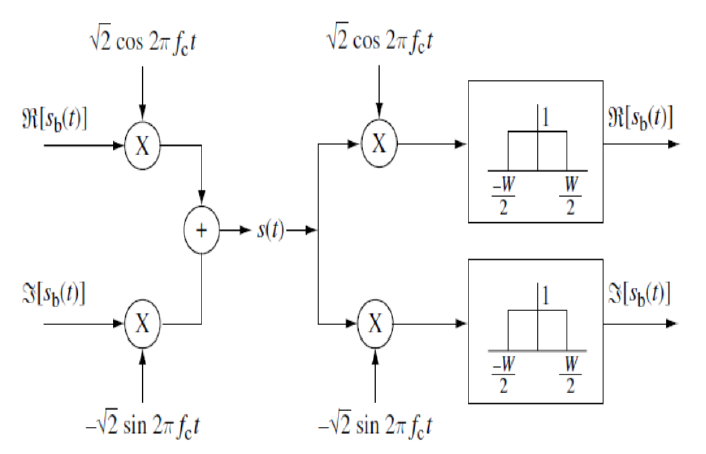
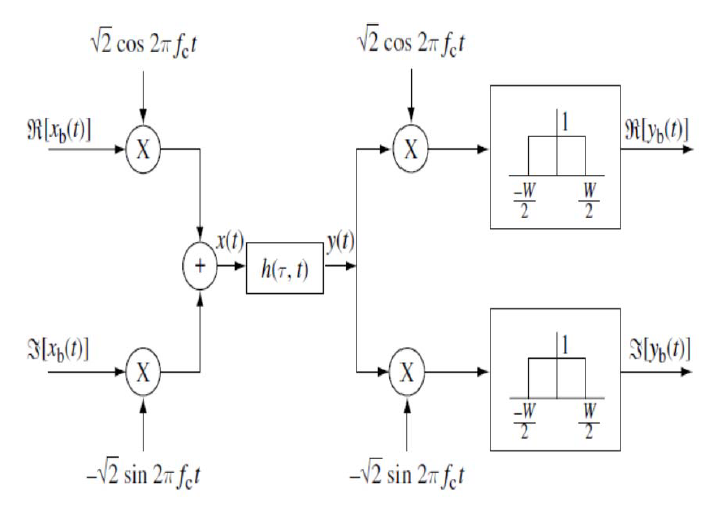
In typical wireless applications, communication occurs in a passband [fc W/2; fc +W/2] of bandwidth W around a center frequency fc. However, most of the processing, such as coding/decoding, modulation/demodulation, synchronization, etc., is actually done at the baseband. Therefore from a communication system design point of view, it is most useful to have a baseband equivalent representation of the system.

Since

it results:

and

and

I do not understand what it wants to get and why. Precisely:

• what does it mean with "equivalent channel model"? I'd say that it means a channel with same input, same output, and same transfer function

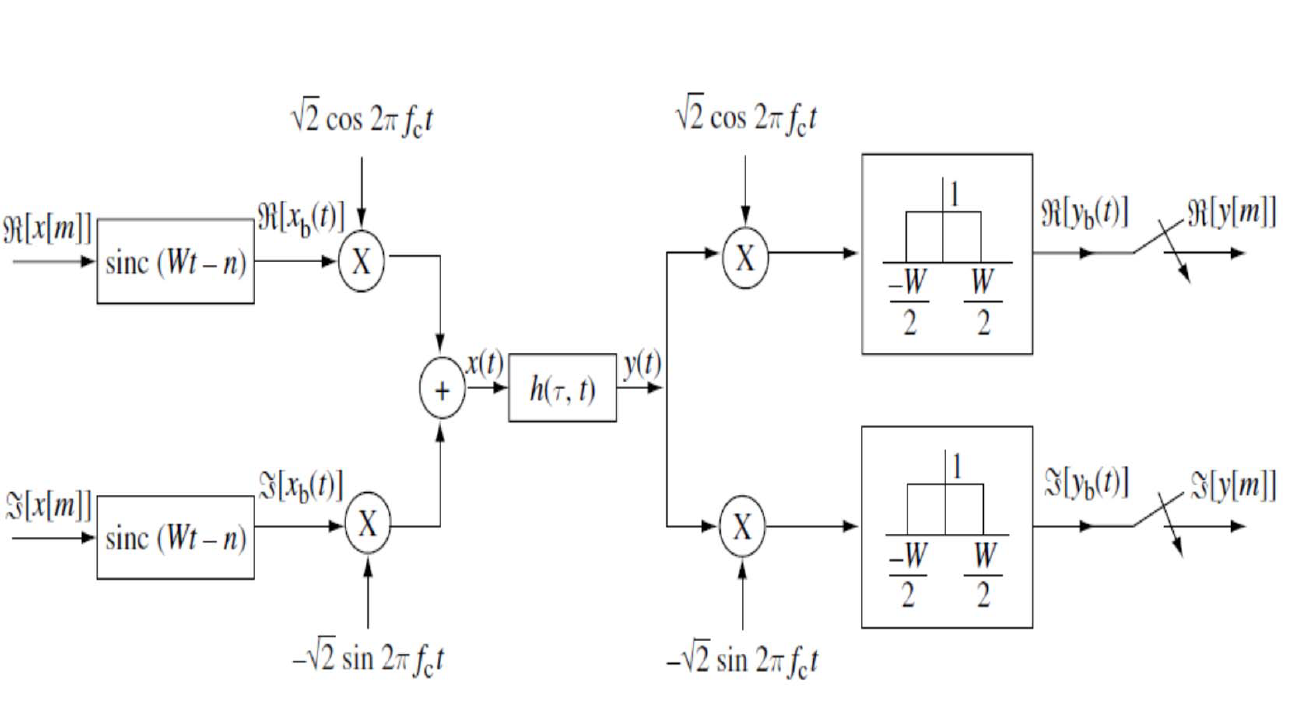
• which is the equivalent baseband channel model between the last two pictures?

• So are baseband signal really used inside a channel?

Answer 1

For many cases where you have a passband signal (meaning, a signal with some carrier frequency, \$f_c\$, and a signal with bandwidth \$W\$ around it, typical in communications systems), analysis is more convenient working with the so-called baseband equivalent version of that signal. It is called baseband equivalent, because it takes out the dependence on \$f_c\$, and can be viewed as a sort of baseband signal that is equivalent to the passband signal in a way. In your example, in the frequency domain, the passband is \$S(f)\$ and the baseband equivalent is \$S_b(f)\$.

Similarly, if you pass the passband signal through a channel, then you'd need a baseband equivalent channel model, that you can pass the baseband equivalent signal through. The whole system is known as the baseband equivalent representation of the system (that is referenced in the quotation you provided).

What is the baseband equivalent of the passband signal? Your example shows a baseband equivalent signal, \$s(t)\$ being derived. Notice that it is no longer real-valued, but complex valued in general. However, it is not a function of \$f_c\$ anymore, which helps with analysis. At the output of the system, you can always convert back to passband if desired.