1
\$\begingroup\$

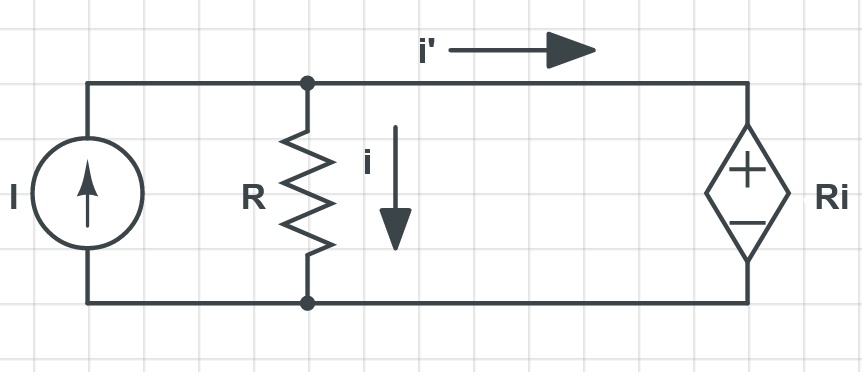
enter image description here

Can we find the value of \$i'\$ in this circuit, considering \$I\$ and \$R\$ are known?

Using Kirchhoff’s Current Law we have that \$I = i + i'\$.

If the dependent source voltage was \$ki \;, \; k \neq R \$ then from Kirchhoff’s Voltage Law we would have \$(k-R)i=0\$, and since \$ k \neq R \$ we have that \$i=0\$, so \$i'=I\$.

What happens when \$ k=R \$, as in the picture above? Kirchhoff's laws are theoretically satisfied for every value of \$i\$. Should we assume that \$i\$ is zero again? Or better, does \$i'\$ and by extension \$i\$ have a fixed value?

Thank you in advance

\$\endgroup\$

1 Answer 1

Reset to default
1
\$\begingroup\$

If \$k = R\$ then the voltage across the dependent source is \$Ri\$. The voltage across the resistor is equal to \$Ri\$. You are correct that KVL and KCL will be satisfied for all values of \$i\$, but that does not mean that you can assume \$i = 0\$.

I think you are being asked to think about the value of \$i'\$ as a function of \$i\$, not as an absolute value. You are almost there.

\$\endgroup\$
3
  • \$\begingroup\$ So, can \$i\$ and \$i'\$ in this circuit take an infinite set of values, with the restriction that they satisfy \$I = i+i'\$ ? Can't we circuitally conclude, that since the dependent source branch has no resistance, then only this branch draws current from the current source, so \$i=0\$ ? \$\endgroup\$
    – user245546
    Commented Mar 19, 2020 at 17:16
  • \$\begingroup\$ An ideal voltage source never has resistance, and it doesn't matter. The only thing we know about a voltage source is that it constrains the voltage between its terminals. The voltage source can have a voltage across it even if it has no resistance. Therefore, there can be a voltage across the resistor, and a current through the resistor, and a current through the dependent source. No, you cannot conclude that \$i=0\$. \$\endgroup\$
    – Elliot Alderson
    Commented Mar 20, 2020 at 0:41
  • \$\begingroup\$ Thank you very much!! \$\endgroup\$
    – user245546
    Commented Mar 20, 2020 at 21:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.