Get some clarification about bias stabilisation with Emitter Degeneration

Question

A textbook I use talks about the effects of adding \$R_{E}\$ in a common emitter amplifier. For stabilization of the circuit due to negative feedback

As the Device gets hotter, more collector current flows, VBE reduces which limits the increase in collector current

What I don't get in this is how when \$V_{BE}\$ reduces this causes a decrease in the collector current as from one of the equations in the textbook $$I_{c}=\alpha I_{E} $$ and $$I_{E}\ =\frac{V_{Bias}-V_{BE}}{R_{E}}$$.

From my rudimentary understanding if \$V_{BE}\$ were to reduce wouldn't \$I_{E}\$ increase which would case an increase in \$I_{C}\$ from the first equation.

Answer 1

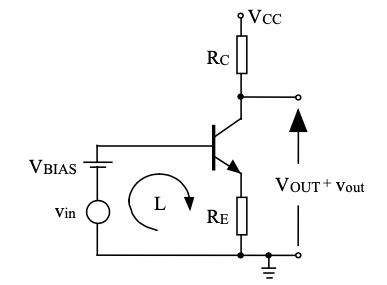
With the following circuit:

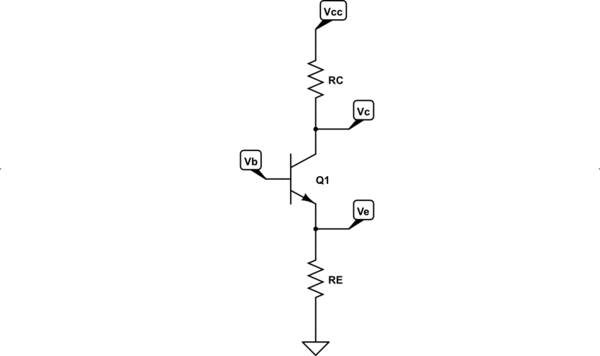
^{simulate this circuit – Schematic created using CircuitLab}

Assume that \$V_\text{B}\$ is a constant (there's no point confusing the issue by including an AC signal riding on the base's DC setpoint.) What are the variables:

• \$V_\text{BE}\$ is temperature-dependent and its value may vary by about \$\frac{-2\:\text{mV}}{^\circ\text{C}}\$ for a given collector current. It gets smaller as the temperature rises.
• \$V_\text{BE}\$ is part-dependent. Different parts from the same bag may have different saturation currents. This alone can be as much as \$\pm 30\:\text{mV}\$, though it is usually not quite that bad.
• \$V_\text{BE}\$ drifts over time.
• \$V_\text{BE}\$ depends upon the collector current (which, with an amplifier stage, varies with signal.) So it's signal-dependent, as well, since the signal impacts the collector current -- it must if it is to affect the collector voltage, in fact.

In short, there are lots of things which impact \$V_\text{BE}\$. Over time and place, all of them are involved.

Considering the above circuit, we can treat small changes in \$V_\text{BE}\$ as similar to small changes in an applied signal. If \$V_\text{BE}\$ makes a small change towards getting smaller, this is just like the input signal changing upward and pulling the emitter up. Similarly, if \$V_\text{BE}\$ makes a small change towards getting larger, this is just like the input signal changing downward and pushing the emitter downward.

You'll probably encounter the following closed loop with negative feedback loop from time to time:

^{simulate this circuit}

Above, B is the negative feedback factor. B is positive when the feedback is negative (per the sign shown at the addition node.) The closed loop gain is \$A_{CLOSED}=\frac{V_\text{OUT}}{V_\text{IN}}=\frac{A_{OPEN}}{1+A_{OPEN}\cdot B}\$. (It's simple to work that out from the above diagram. If you haven't already done so, sit down with pencil and paper and give it a try.)

As you probably already know, the closed loop voltage gain of the above circuit is \$A_v=\frac{R_\text{C}}{R_\text{E}}\$. So we can equate these:

$$\frac{A_{OPEN}}{1+A_{OPEN}\cdot B}=\frac{R_\text{C}}{R_\text{E}}$$

and solve for B:

$$B=\frac{R_\text{E}}{R_\text{C}}-\frac1{A_{OPEN}}$$

Given the Ebers-Moll model for the BJT, we know that \$A_{OPEN}\approx \frac{R_\text{C}\cdot I_\text{C}}{V_T}\$ and also that when \$R_\text{E}\gg\frac{V_T}{I_\text{C}}\$ then \$I_\text{C}\approx I_\text{E}=\frac{V_\text{B}-V_\text{BE}}{R_\text{E}}\$. From these, we can work out that so long as \$\frac{V_T}{I_\text{C}}\ll R_\text{E}\lt R_\text{C}\sqrt[+]{\frac{V_\text{B}-V_\text{BE}}{V_T}}\$ then B is positive and therefore the feedback effect of \$R_\text{E}\$ is negative.

Roughly speaking, for any reasonably design, \$B\approx \frac{R_\text{E}}{R_\text{C}}=\frac1{A_v}\$. So, smaller values of \$A_v\$ imply more negative feedback and therefore more stability with respect to changes in \$V_\text{BE}\$. Another way of putting that is that the more negative feedback you apply, the more stable the circuit is to vagaries relative to variations of \$V_\text{BE}\$ (whether due to temperature, part variation, drift over time, etc.) But also the lower the voltage gain of the circuit. So you make trade-offs. More gain, less stable. Less gain, more stable. It's your call where to place this balancing act.

Answer 2

If \$I_E\$ increases, \$V_{R_E}\$ must increase.

\$V_{\ bias}\$ is fixed, so KVL means \$V_{BE}\$ decreases, which decreases \$I_B\$, which decreases \$I_C\$ and \$I_E\$, stabilizing the Q-Point.

The Q-Point changes, but the addition of \$R_E\$ causes greater stability.

From Input characteristic curve of common collector configuration.

Answer 3

Adding Re makes the base current more stable by reducing the effects of Vbe(T) ~ -2 to -2.4mV/'C as I=Ve/Re is more stable.

So it reduces the rise of current with rising temperature and lower Vbe, as well as reducing the voltage gain to collector by Rc/Re so this makes it more linear. Adding C across Re, will boost the AC gain for f>1/ReCe

Answer 4

With RE equal to 0 Ohms the collector current is determined by VBE and any small variation in temperature will cause a small variation in VBE (-2.1mV/degC) which will cause a significant variation in collector current and the DC bias at the collector.

By adding RE (emitter degeneration) to the circuit, the collector current is now essentially set by the voltage at the emitter divided by RE and any small variation in VBE with temperature change will have a small effect on VE (relative to its magnitude) and therefore a relatively small effect on IC.

This is the reasoning behind the argument that VE should be at least 1V in a common emitter amplifier so that any small variation in VBE with temperature will cause a relatively small variation of the voltage across the emitter resistor (and therefore the current through it) thereby temperature stabilising the collector current.