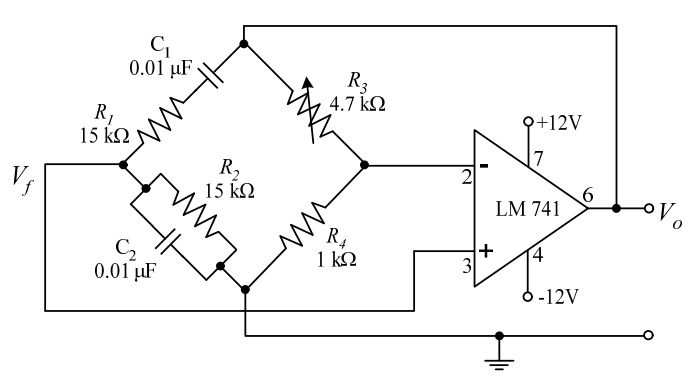
I am learning about Wien bridge oscillators. Following Experiment No. 9: WIEN BRIDGE OSCILLATOR USING OPAMP, they use the following schematic:
They then arrive at the following equation half-way down page 3:
They then equate the imaginary part to 0 to find the resonant frequency, i.e:
This makes sense, because at resonance the current is in phase with the voltage, hence the imaginary part goes to 0. I don't understand the next part where they:
To obtain the condition for gain at the frequency of oscillation, equate the imaginary part to zero.
which expressed as an equation gives you:
Why can you just equate the imaginary part to zero (i.e. the real part goes to 0) to find the gain needed at resonance?
EDIT: After @TimWescott's great answer I created a re-written version of the equation which shows the "missing link", i.e. Im() + Re() = 0:

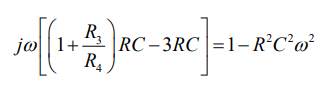

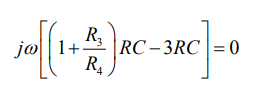
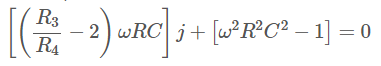
Im() = 0and they had expressed the equation asIm() = Re(), so that you could view them as replacingRe()in theIm() = Re()equation with0. \$\endgroup\$