Find V0(t) in this circuit assuming zero initial conditions.
I solved it (refer image above) using frequency-domain analysis using Laplace and found the answer, but I am not able to get the same answer using time-domain analysis. How to solve it in thr time domain using transient analysis?
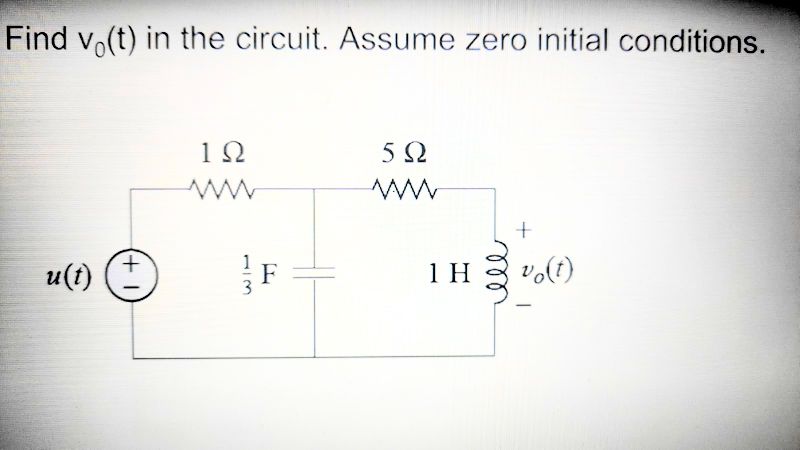
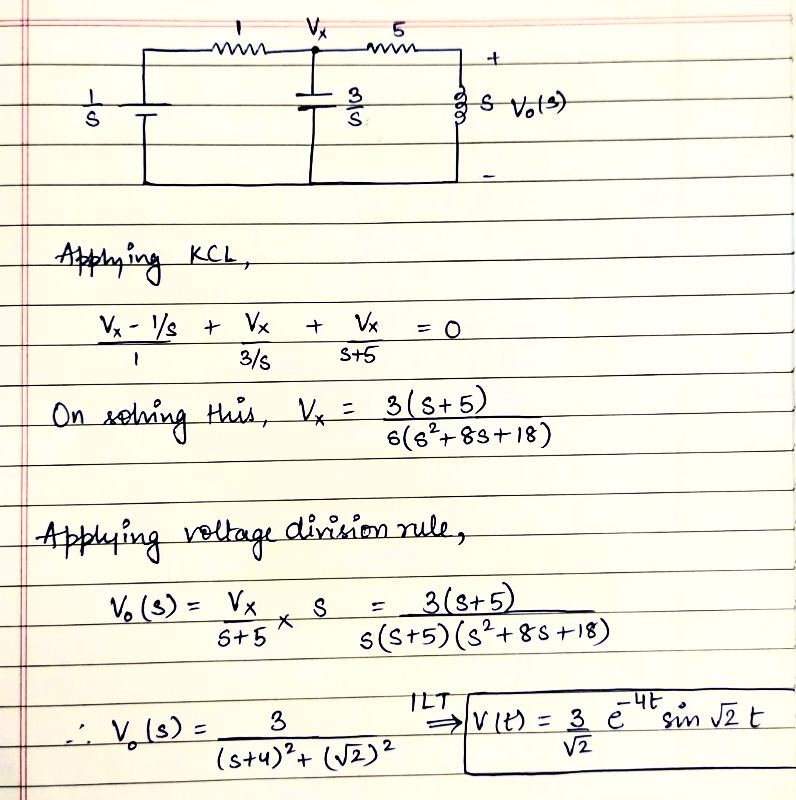
Using nodal analysis at the \$\small V_x\$ node: \$\Sigma \small currents\:away\:from \:node =0 \$
\$\large\frac{(V_x -1)}{1}\: +\large\frac{1}{3}\frac{dV_x}{dt}+\large\frac{1}{5}\left(\small V_x +\large\frac{dV_x}{dt}+\frac{1}{3}\frac{d^2V_x}{dt^2}\right)\small=0\$
Simplifying,
\$\large\frac{d^2 V_x}{dt^2}\small+8\large\frac{dV_x}{dt}\small +18V_x=15 \$
Auxiliary equation:
\$\small m^2 +8m +18=0\$
\$\small m=-4\pm j\sqrt{2}\$
Complementary function:
\$\small V_{xcf}(t)=e^{-4t}(A cos\sqrt{2}t+Bsin\sqrt{2}t) \$
Supplement, in response to comment:
\$\small i_L=\large \frac{1}{5}\small (V_x-V_0)=\large \frac{1}{5}\small \left(V_x-\large\frac{d}{dt}\small i_L\right) =\large \frac{1}{5}\small \left(V_x-\large\frac{d}{dt}\small(i-i_C)\right) =\large \frac{1}{5}\small \left(V_x-\large\frac{di}{dt}+ \frac{di_C}{dt}\right)\$
where \$ i\$ is the source current, \$i_L \$ is the inductor current, and \$\small i_C\$ is the capacitor current.
Thus,
\$ i_L=\large \frac{1}{5}\small \left(V_x+\large\frac{dV_x}{dt}+\frac{1}{3} \frac{d^2V_x}{dt^2}\right)\$
Also, the other two currents are,
\$\small i=1-V_x \$, and \$ i_C=\large\frac{1}{3}\frac{dV_x}{dt}\$
Finally, node current balance,
\$i+i_C +i_L=0\$
gives,
\$\large\frac{d^2 V_x}{dt^2}\small+8\large\frac{dV_x}{dt}\small +18V_x=15 \$
You've already used Kirchhoff for the Laplace domain, why not use it for the time domain, too? I'll use the loop:
$$\begin{align} &\begin{cases} 1\cdot i_1(t)+\dfrac{1}{\frac13}\int{[i_1(t)-i_2(t)]\mathrm{d}t}&=\theta(t) \\ \dfrac{1}{\frac13}\int{[i_1(t)-i_2(t)]\mathrm{d}t}&=5\cdot i_2(t)+1\cdot \dfrac{\mathrm{d}i_2(t)}{\mathrm{d}t} \end{cases} \\ \Rightarrow \\ &\begin{cases} 1\cdot \dfrac{\mathrm{d}i_1(t)}{\mathrm{d}t}+\dfrac{i_1-i_2}{\frac13}&=\delta(t) \\ \dfrac{i_1-i_2}{\frac13}&=5\cdot \dfrac{\mathrm{d}i_2(t)}{\mathrm{d}t}+1\cdot \dfrac{\mathrm{d}^2i_2(t)}{\mathrm{d}^2t} \end{cases} \tag{1} \\ \Rightarrow \\ i_2(t)&=\dfrac16-\dfrac13\mathrm{e}^{-4t}\left(\sqrt2\sin(\sqrt2 t)+\cos(\sqrt2 t)\right) \tag{2} \end{align}$$
\$i_1(t)\$ can be discarded. Since the voltage across the inductor is the derivative of the current through it:
$$\dfrac{\mathrm{d}i_2(t)}{\mathrm{d}t}=\dfrac{3}{\sqrt2}\mathrm{e}^{-4t}\sin(\sqrt2 t) \tag{3}$$
