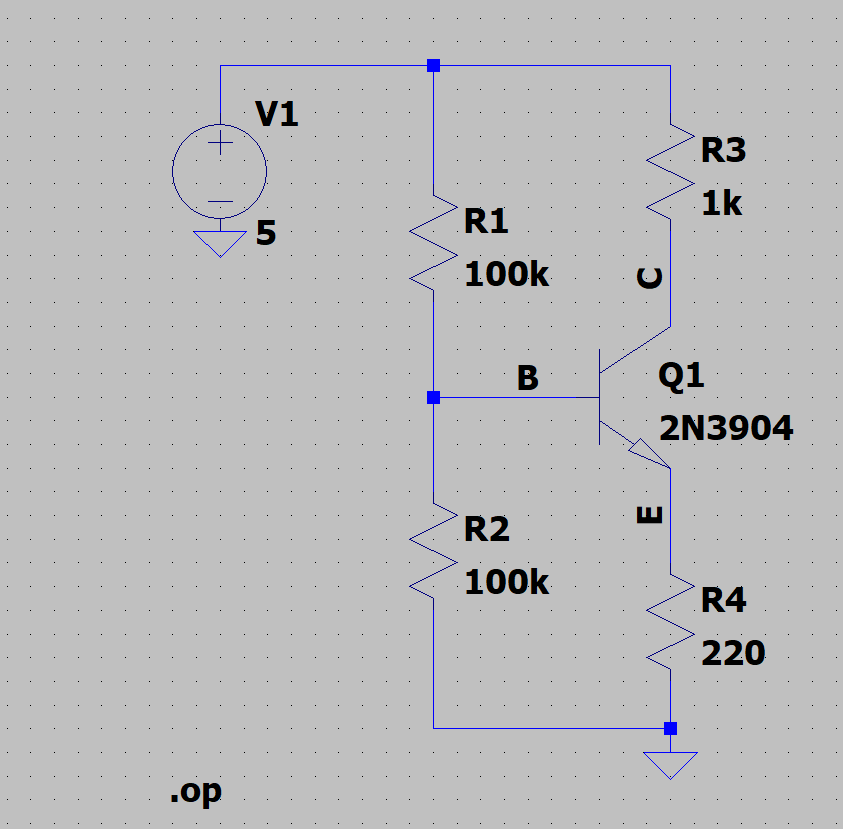
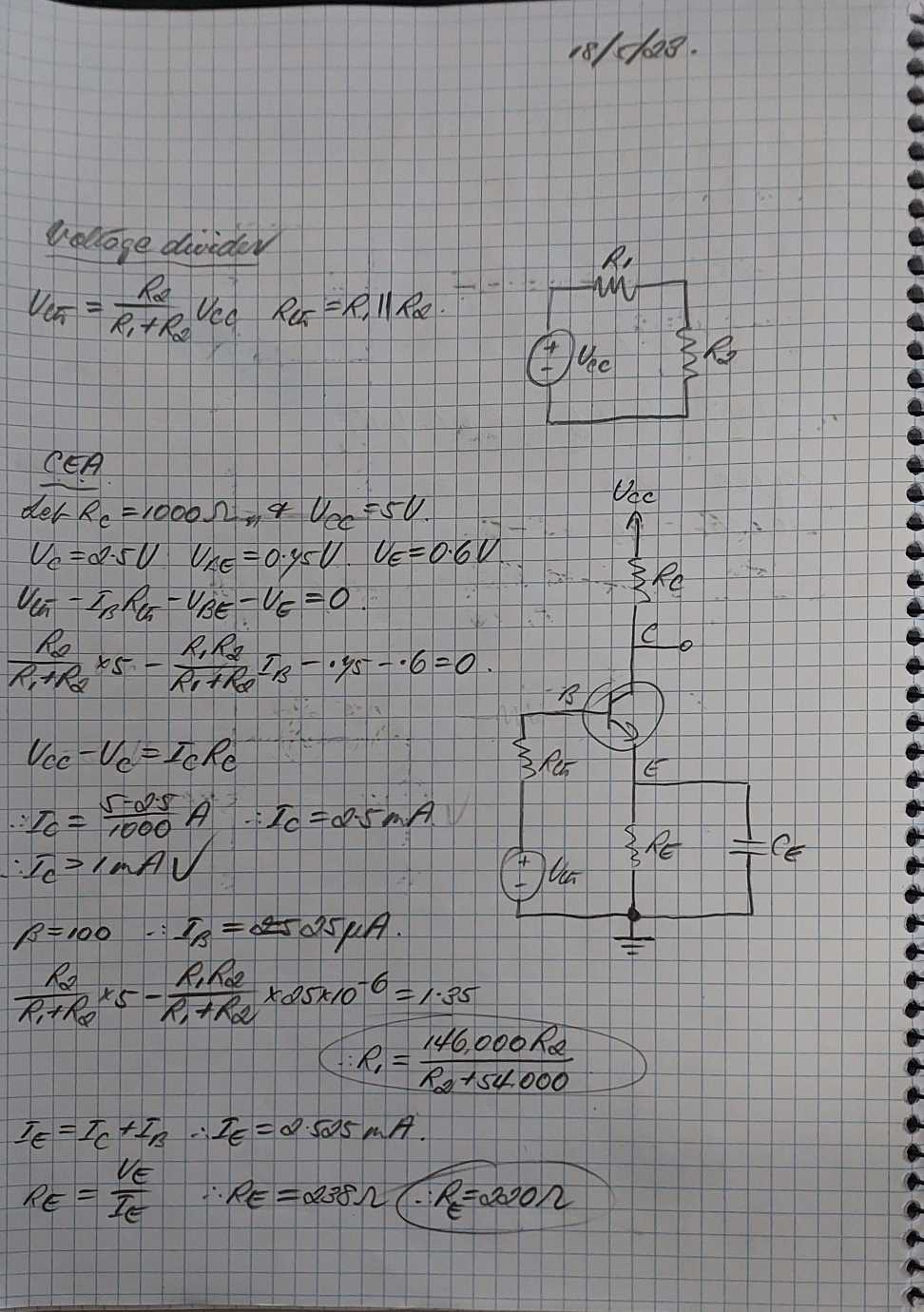
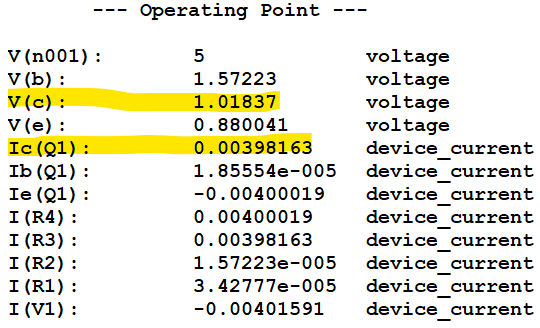
I'm trying to design this common emitter amplifier to have a Q-point of \$(V_C=2.5 V, I_C = 2.5 mA)\$. According to my calculations (see below), if I were to use \$R_2 = 100 k\Omega\$, I should also use \$R_1 = 100 k\Omega\$ to achieve this. However, when I ran a DC analysis of my circuit on LTspice, it said that my Q-point was at \$(V_C=1.02 V, I_C = 3.98 mA)\$. Would anyone be able to explain where I went wrong?
Your emitter is at \$550\:\text{mV}\approx 2.5\:\text{mA}\cdot 220\:\Omega\$. Your base will need to be about \$700\:\text{mV}\$ above this, or \$1.25\:\text{V}\$.
The estimated base current, given \$\beta=100\$, would be \$25\:\mu\text{A}\$. The goal is for this value to be small in comparison with the voltage divider current found in the biasing pair. The general rule is to use 10 times what you expect as the base current, or in this case about \$250\:\mu\text{A}\$.
So \$R_1+R_2=\frac{5\:\text{V}}{250\:\mu\text{A}}=20\:\text{k}\Omega\$.
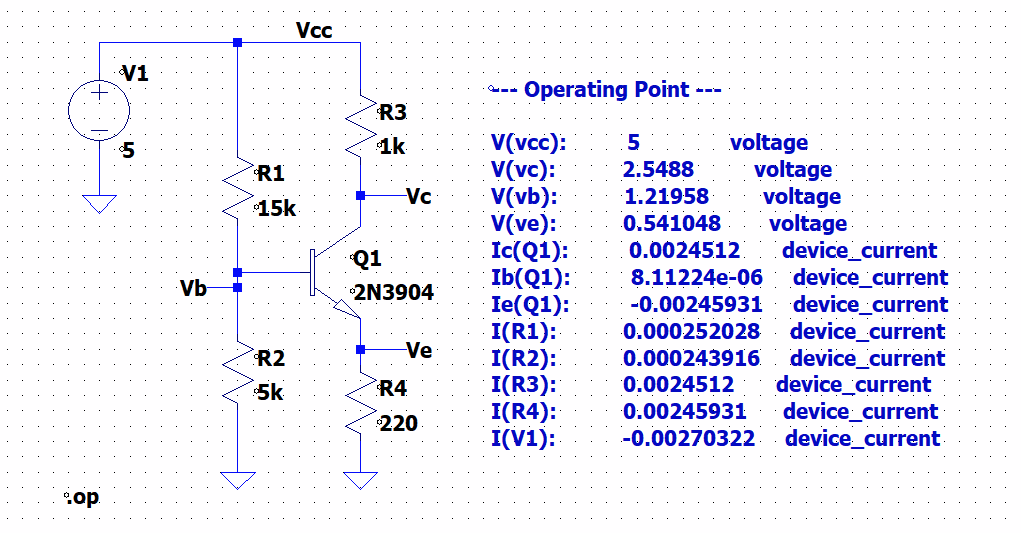
Also, you know that \$5\:\text{V}\cdot\frac{R_2}{R_1+R_2}=1.25\:\text{V}\$ and since \$R_1+R_2=20\:\text{k}\Omega\$ you can plug that in, \$5\:\text{V}\cdot\frac{R_2}{20\:\text{k}\Omega}=1.25\:\text{V}\$, and find that \$R_2=5\:\text{k}\Omega\$ and therefore that \$R_1=15\:\text{k}\Omega\$.
Plugging those into a schematic:
