Voltage between two terminals of an open circuit which's connected in series with a current source

Question

^{simulate this circuit – Schematic created using CircuitLab}

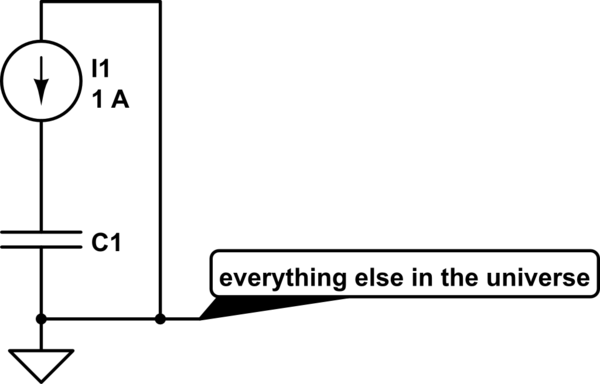
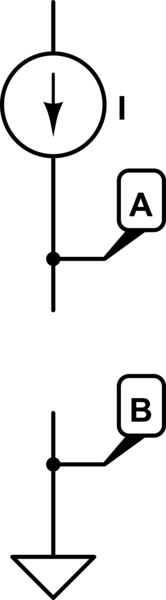
What will be the voltage between nodes A & B?

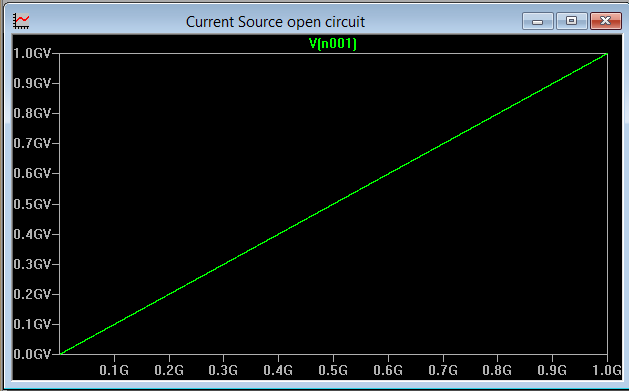
My guess is that the voltage between nodes A & B should be increasing forever as the current source,I will keep on pumping charges to node A & due to increasing charge voltage will keep on rising.

Am I right?If not why?

Will the answer remain same if there's a voltage source at the terminal of current source,other than A?

Thanks for reading a question as silly as this.

If someone can suggest a source(book,site etc) where more such questions may be found,that would be great.

Answer 1

An open circuit, by definition, can have no current. A current source, by definition, has a non-zero current. The two are in series so the currents must be equal, but can't be. You have created an impossible circuit.

The dual of your impossible circuit would be an ideal voltage source into a short:

^{simulate this circuit – Schematic created using CircuitLab}

A short, by definition, can have no voltage across it. A voltage source, by definition, does. The two are in parallel so the voltages must be equal, but can't be. This circuit is equally impossible.

You could say that the current in this circuit is infinite, and the the voltage in your circuit is infinite. What's the power in those circuits?

$$ P = I E = 1A \cdot \infty V = \infty W$$

$$ P = IE = \infty A \cdot 1V = \infty W $$

The power is infinite. What does that even mean? I have no idea: you will have to ask a mathematician.

If you were to consider that any two separated conductors are a capacitor, then maybe the circuit you had in mind was this:

^{simulate this circuit}

Then yes, the voltage across C1 will increase, linearly, forever.

Answer 2

For an ideal theoretical current source with open terminals, you could consider the potential between to be infinite*.
*(though as Phil notes, it is an "impossible" circuit so the answer is meaningless really - an ideal current source has a constant current, so you are asking a current to flow through an infinite resistance which makes no sense)

Since \$ V = I * R \$ it follows that \$ I \cdot \infty R = \infty V \$

Example in SPICE with a 1A ideal source and the resistance increased from \$ 0 \$ to \$ 1G \Omega \$: