3
\$\begingroup\$

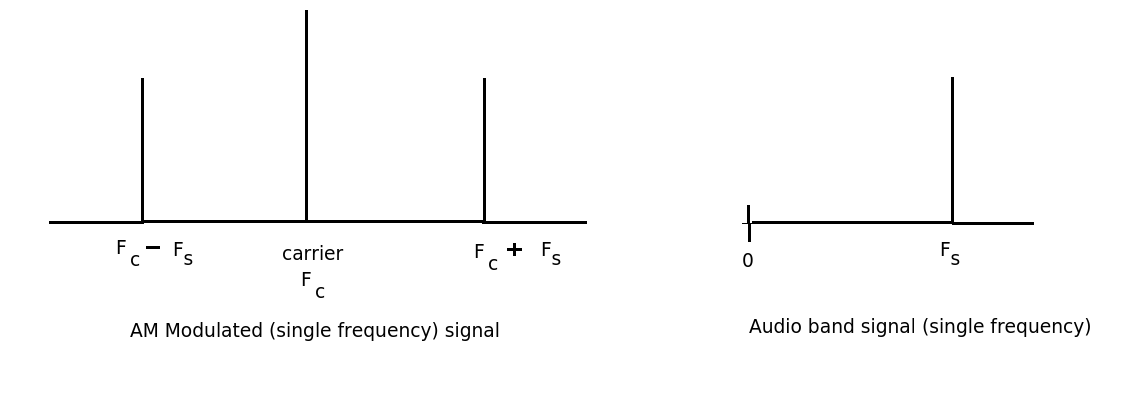
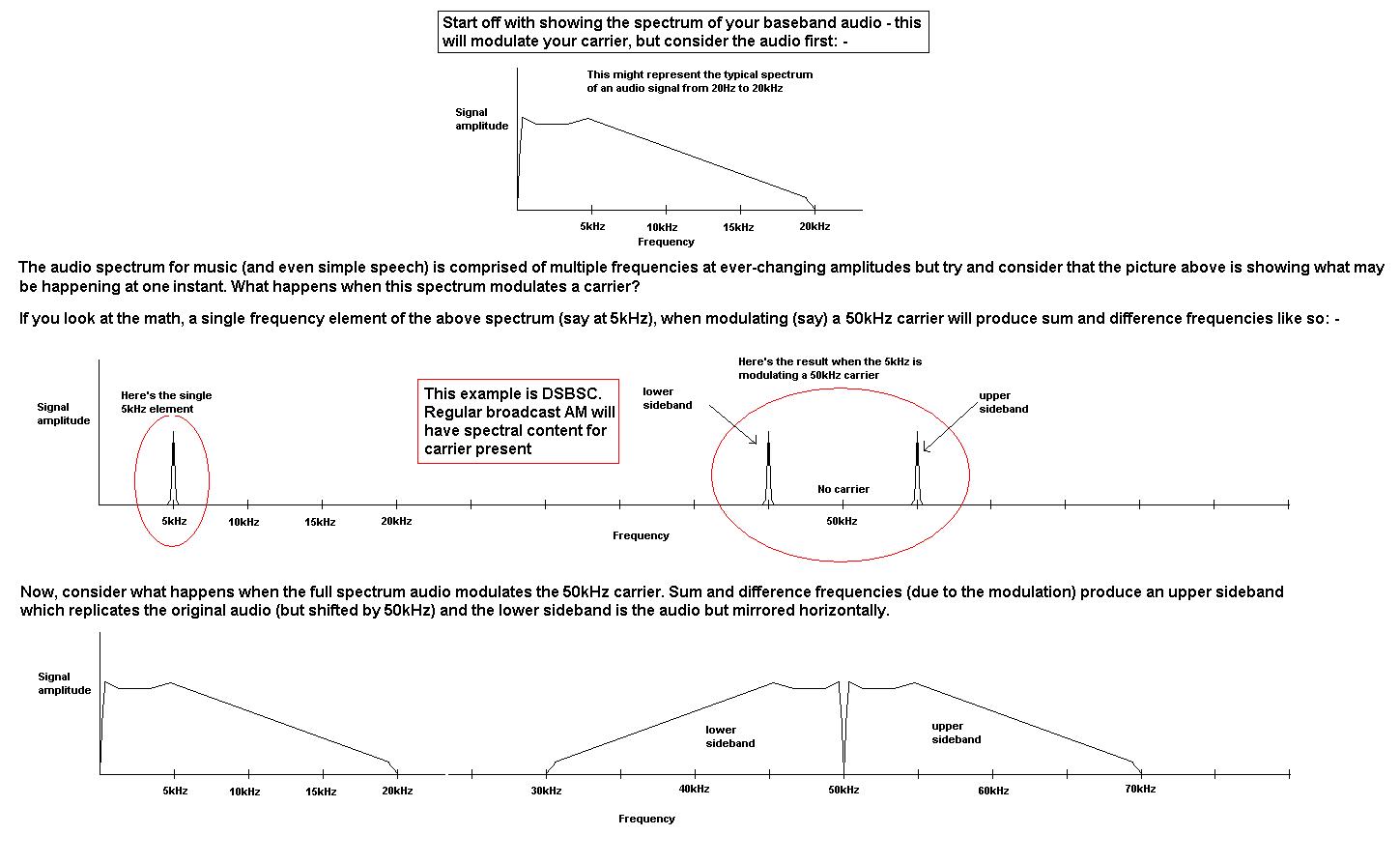
If I have an oscillator at 10 kHz outputting 5 W, using AM, like this.

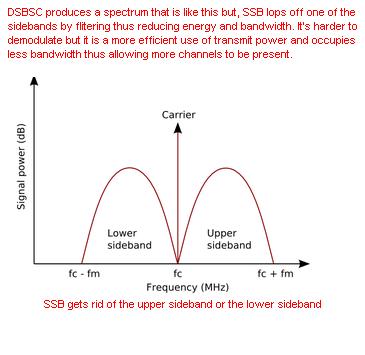
enter image description here

Where does the power in the sidebands come from? Is the power the result of the harmonic relationship between the carrier and it's 2x harmonic and it's 1/2 x (?!) harmonic?

Is the power the result of a fundamental electromagnetic physical principle that power at a certain frequency x always spreads out a bit? And the higher the wattage, the more the spread?

Is this power intentionally generated to produce an RF envelope with a certain bandwidth with the MOST power at the center? If so, don't very high and very low modulating signal frequencies receive attenuation?

And 2) if so, when we say we are broadcasting a 10 kHz, we are really saying we have the most power at. 10 kHz, but we certainly are 'using' a lot more frequency space. And if so, why do we need all that space, I though the AM was transmitting information using modulation of the amplitude of the carrier to propagate a voice waveform.

What are the peaks in the sidebands caused by?

\$\endgroup\$

2 Answers 2

Reset to default
1
\$\begingroup\$

The diagram is misleading you by representing the signal as some perfectly distributed band.

The sideband frequencies are the result (literally the product) of the audio or signal frequencies acting on the carrier. If there was only a single (sinewave) signal the spectrum would look like this.

enter image description here

\$\endgroup\$
2
  • \$\begingroup\$ So, this is CW . Where only bineary state of a single sine wave are used to serially transmit information. If I tried to send my voice over one sine wave it would be garbage output. ? It woud just be on or off when ever my voice hit a particular note, and unintelligible? And if I had say 50 high power output stage transistors , I could be switching sine wave at different frequencies throughout and actually send 50 different serialzed messages, uninterfered with, through this system? \$\endgroup\$
    – Andyz Smith
    Commented Aug 24, 2013 at 13:20
  • \$\begingroup\$ @AndyzSmith No. CW would simply be the tone being switched on and off. The diagram shows only 1 particular frequency in order to clarify what is happening with the carrier and the sidebands produced by that particular frequency. A normal audio signal would be a spectrum of frequencies ranging from about 30Hz up to about 2.5kHz (not the full audio range). This means the sideband take up a 2x 2.5kHz i.e 5kHz with the carrier frequency centred. \$\endgroup\$
    – JIm Dearden
    Commented Aug 24, 2013 at 15:11
1
\$\begingroup\$

Here's a better diagram that might explain your confusion. I'm using baseband audio from 20Hz to 20kHz modulating a 50kHz carrier for the example: -

enter image description here

I'm sorry it's a bit hard to read but if you magnify your web browser it'll be easier. The power in the sidebands comes from both the original baseband signal and the carrier (even though it isn't present anymore). The full spectrum of the final picture goes from 30kHz to 70kHz because it is twice the baseband spectrum of 20kHz.

And now the (simplified) maths and it comes down to accepting that: -

\$sin(a).sin(b) = \frac{1}{2}(cos(a-b) - cos(a+b))\$

\$sin(b)\$ can be regarded as the modulating signal and for simplicity \$b = 2\Pi Ft\$ and let's choose \$F\$ to be a single frequency of (say) 5kHz.

\$sin(a)\$ can be the carrier at 50kHz.

Now look what \$sin(a)sin(b)\$ yields in the formula. There is a \$cos(a-b)\$ and a \$cos(a+b)\$ term. These are new frequencies of 45kHz and 55kHz. OK they're cosine waves but that just means they're shifted by 90º to the equivalent sinewave.

\$\endgroup\$
14
  • \$\begingroup\$ The power in the sidebands comes from the oscillator output stage we control with the input signal? So actually the 'transmitting' on 50 kHz is really allowing the output stage to travel and radiate electromagnetic energy at 50.1 kHz at 4 W at 50.2 kHz at 4 w, a 50.3 kHz a 4 w and so on , forever. S when we say 'carrier' we just mean, well, if I produce a high amplitude baseband modulation input 'right snack in the middle of my baseband bandwidth' , then I will get a very high energy output 10 w at exactly 50 kHz. \$\endgroup\$
    – Andyz Smith
    Commented Aug 24, 2013 at 13:13
  • \$\begingroup\$ If the baseband power is 1W and the carrier is 1W and we used a perfect mixer (aka analogue multiplier because that's what it is) then the composite signal will be 2W; 1W in the lower s/b and 1W in the upper s/b. Power comes from both carrier (single frequency) and the baseband and for DSBSC there will be no power at 50kHz because there are no dc components in the audio. \$\endgroup\$
    – Andy aka
    Commented Aug 24, 2013 at 13:17
  • \$\begingroup\$ The power comes from the output stage of the transmitter, period? \$\endgroup\$
    – Andyz Smith
    Commented Aug 24, 2013 at 13:21
  • \$\begingroup\$ @AndyzSmith actually the power comes from the power supply that feeds the PA (LOL) but I'm talking about the \$relative\$ power levels in the signal and how they are distributed in the modulated spectrum. It doesn't matter if it's 1W or 1kW, the spectral shape remains the same and ignoring the PA, the power comes equally from the original carrier and the original modulation signal at baseband. \$\endgroup\$
    – Andy aka
    Commented Aug 24, 2013 at 13:49
  • \$\begingroup\$ So, if I have a high amplitude spectral peak n my baseband audio envelope at 5 kHz ( sounds like a dial tone, but not a whistle ) then when that baseband audio is controlling the frequency of a high power output transistor oscillation frequency the I will get a electromagnetic radiated energy peak at 55 kHz and 45 kHz. \$\endgroup\$
    – Andyz Smith
    Commented Aug 24, 2013 at 13:49

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.