4
\$\begingroup\$

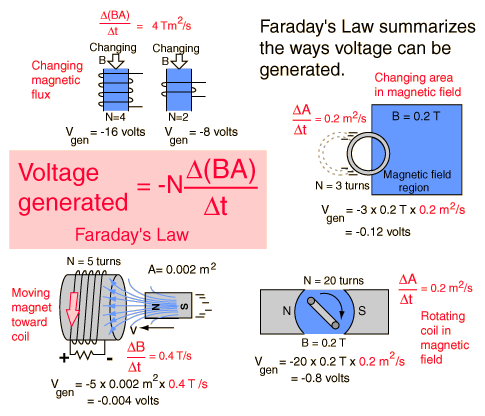
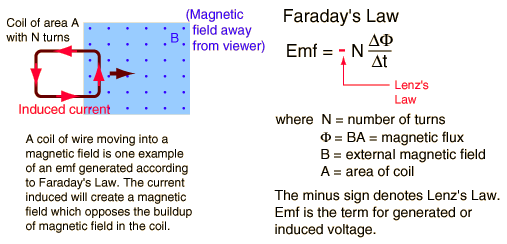
I always hear something like "reduce the loop area" when reading materials on EMI reduction. Why we need to minimize the loop area? I have two guesses:

  1. Smaller loop area will influence less signal tracks, since it will overlap with less tracks.
  2. Small loop area will radiate less?? (If so, why is this?)

Are my guesses correct? How could I learn the EMI issue with in a more systematic way, maybe starting on teaching myself RF theories?

\$\endgroup\$
1
  • 1
    \$\begingroup\$ RF is a different matter. Knowledge of RF comes in handy when understanding EMC / EMI, but it is not essential knowledge to get about what is going on. \$\endgroup\$
    – jippie
    Commented Jul 9, 2014 at 5:11

3 Answers 3

Reset to default
7
\$\begingroup\$

At a high level ... I hope someone else will explain in more detail and with the underlying theory.

Loops have to do with magnetic coupling / interference / compatibility between two circuits.

With a larger loop, more magnetic field lines will fit inside the loop, so the magnetic coupling between this loop on your PCB and an interfering outside magnetic field can be better. If you make your loop infinitely small, then no field lines fit inside your loop, hence no magnetic coupling is possible.

\$\endgroup\$
4
  • \$\begingroup\$ Why does the size of the loop decide the size of the antenna? \$\endgroup\$
    – richieqianle
    Commented Jul 9, 2014 at 8:09
  • \$\begingroup\$ @richieqianle because at a given 'flux' density or field line density, there are more field lines enclosed in a larger loop than in a smaller loop. The amount of flux inside the loop is what really counts. \$\endgroup\$
    – jippie
    Commented Jul 9, 2014 at 9:01
  • \$\begingroup\$ Thanks! I mean, why does the size of the loop decide how much field lines will be enclosed, instead of the size of, say the board? \$\endgroup\$
    – richieqianle
    Commented Jul 14, 2014 at 7:19
  • \$\begingroup\$ @richieqianle It is easier to determine the surface (and with that its effective number of field lines) inside a loop than the infinitely large surface outside the loop (where numerous different fields will largely cancel each other out). The size of the board doesn't matter at all as that is just an arbitrary size, if you change the size of the board, you won't change the EM field, nor the loop. \$\endgroup\$
    – jippie
    Commented Jul 14, 2014 at 8:29
5
\$\begingroup\$

It's all about Faraday's Law when it comes to picking up interference (one half of the story).

enter image description here

Faraday's law is a fundamental relationship which comes from Maxwell's equations. It serves as a succinct summary of the ways a voltage (or emf) may be generated by a changing magnetic environment. The induced emf in a coil is equal to the negative of the rate of change of magnetic flux times the number of turns in the coil. It involves the interaction of charge with magnetic field.

enter image description here

All taken from this website. Basically the bigger the area of the coil the more flux it can receive and the greater the voltage it can produce across its terminals. For the creation of a mag field see this (taken from same site): -

enter image description here

Basically, the bigger the area of the coil, the bigger the magnetic field generated hence the greater the interference received.

\$\endgroup\$
2
  • \$\begingroup\$ Thanks Andy! It explains well that larger loop reads larger interference from the environment. But why do larger loops produce more EMI? \$\endgroup\$
    – richieqianle
    Commented Jul 14, 2014 at 7:18
  • 1
    \$\begingroup\$ @richieqianle - a larger loop area has more inductance and inductance is defined as total flux per amp therefore, for a given current (and a larger loop area), more interfering flux is generated. \$\endgroup\$
    – Andy aka
    Commented Jul 14, 2014 at 7:26
1
\$\begingroup\$

The answer that's most easy to understand is that a big antenna radiates more than a small antenna. And that is what the loop is. Also, as you make the loop (antenna) smaller, you raise the frequency at which it will (want to) radiate. At some point it is so small that the radiation is greatly reduced.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.