1
\$\begingroup\$

In this circuit, the switch has been open for a long time that is the 2F capacitance is full. Now we close the switch. What is the equation of the current and voltage of the 2F capacitance after closing the switch?

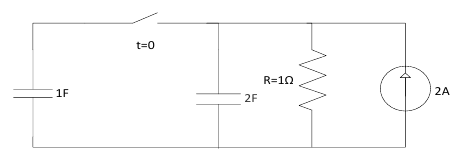
enter image description here

Here is my solution which I think is wrong:

$$ 3\frac{dv}{dt} + v = 2 $$

$$ 3\frac{dv}{dt} + v = 0 \\ v = ae^{bt} \\ 3b + 1 = 0 \\ b = \frac{-1}{3} \\ v = ae^{\frac{-1}{3}t} + 2 $$

in t = 0 V should be 2 : $$ a + 2 = 2 \\ a = 0 $$

Which means that V will be constant after closing the switch and no current will pass through the 1F or 2F capacitance, which is wrong.

\$\endgroup\$
0

4 Answers 4

Reset to default
1
\$\begingroup\$

You have the right general idea, but you can't just consider the two capacitors as one 3F capacitor. Just before the switch is closed, the 2F capacitor will be fully charged and (I presume) the 1F capacitor is fully discharged. So when the switch is closed, the 2F capacitor will discharge and the 1F capacitor will charge.

Remember that \$Q=CV\$ for a capacitor, and that \$\displaystyle \frac{dQ}{dt} = C\frac{dV}{dt} = i_C\$ and this should help you derive the answer.

\$\endgroup\$
1
  • 1
    \$\begingroup\$ And keep in mind that at the moment the switch closes, there will be an arbitrarily large, arbitrarily short pulse of current that flows between the two capacitors. This "singularity" causes some of the normal rules to break down, so be careful. For example, conservation of charge holds, but conservation of energy does not. \$\endgroup\$
    – Dave Tweed
    Commented Dec 13, 2014 at 18:42
1
\$\begingroup\$

What you're trying to do is not well-defined, and is not allowed in circuit theory anyway. You need an initial state for the 1 F capacitor. And you can't just short together two capacitors at different voltages. That would imply infinite current and violate conservation of energy.

http://www.users.on.net/~ithilien/tam/electronics/CapacitorParadox.html

You need to assume some resistance or inductance between the capacitors in order to analyze the circuit.

\$\endgroup\$
1
\$\begingroup\$

You cannot use the standard (lumped element) circuit analysis to model the circuit at the time the switch is closed because dv/dt of the capacitors being infinite.

So this has to be done in two steps. Apply conservation of charge at the instance when the switch is closed to figure out the state of the capacitors. That becomes the initial condition for the subsequent analysis, which is just an RC circuit.

\$\endgroup\$
-2
\$\begingroup\$

The capacitors will explode or the wire will melt.

The hydraulic analogy is a dam burst.

You have emptied the capacitor into a dead short.

\$\endgroup\$
4
  • \$\begingroup\$ No, this is essentially incorrect. There's a finite amount of energy involved, so nothing will explode. \$\endgroup\$
    – Dave Tweed
    Commented Dec 13, 2014 at 22:29
  • \$\begingroup\$ But there is infinite power. With capacitors in the farad range, something could certainly explode or melt. Regardless, the model will not be correct, because you can't really have an ideal switch and zero-impedance wires. \$\endgroup\$
    – Adam Haun
    Commented Dec 14, 2014 at 4:11
  • \$\begingroup\$ @AdamHaun: No, as long as we're talking about theoretically ideal components, there's zero power dissipation. \$\endgroup\$
    – Dave Tweed
    Commented Dec 14, 2014 at 15:55
  • \$\begingroup\$ Circuit theory doesn't cover this situation. There can't be a discontinuity in the voltage across an ideal capacitor. If you try to do it, you end up having to choose between conversation of charge and conservation of energy. (This is called the capacitor paradox.) Obviously, this means the model is broken. The limiting case of a very small resistance between the capacitors shows what really happens -- charge is conserved, and the "missing" energy is dissipated in the resistance. Infinitesimal resistance gives infinite current and infinite power over an infinitesimal period of time. \$\endgroup\$
    – Adam Haun
    Commented Dec 14, 2014 at 17:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.