transmission lines voltage drop

Question

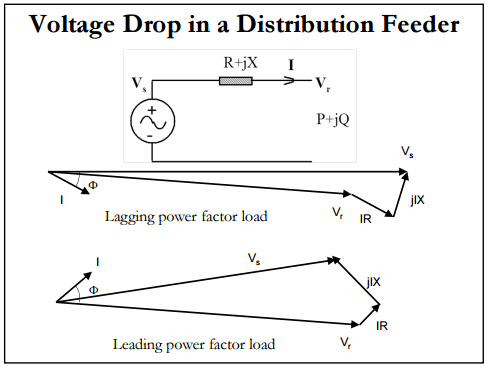
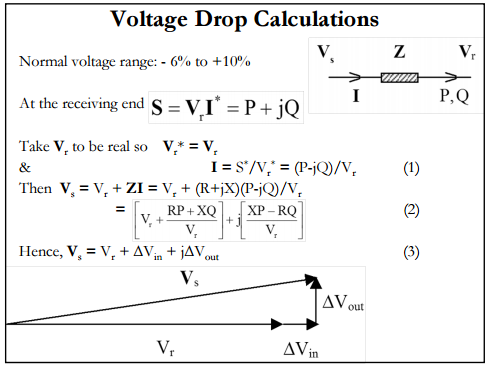
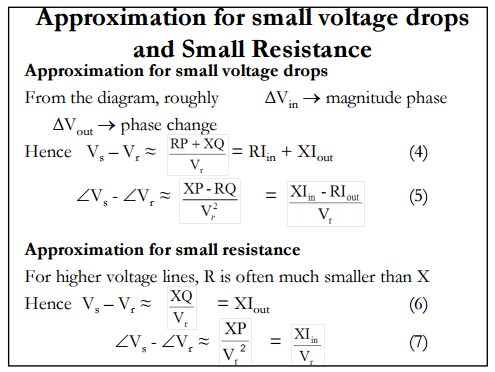
When trying to explain the need for high voltage in transmission lines, I came across a formula in one of my old courses: A transmission line is considered, and between the generator end and the load there is a resistor \$R_k\$ and inductor with an impedance \$ X_k\$ modelizing the transmission line. The voltage drop across it became this:

\$ \Delta U\;=\; U_1 \;-\;U_2 \; = \; \frac{1}{U_L}\big( PR_k+QX_k \big) \$

I'd like to know where this formula comes from, I might not have searched enough but I have not found it so far... Also, would it be correct to write the following:

\$ P=RI^2= \frac{U^2_1-U^2_2}{R}= \; \frac{1}{R\cdot U_L}\big( PR_k+QX_k \big)(U_1+U_2)\$
==> "To reduce transmission losses, current can be decreased, or the line voltage increased".

Answer 1

The formula

$$ \Delta V \approx P R + Q X \textrm{ [per unit]}$$

appears to be based on an assumption that the voltage drop will be relatively small.

I have excerpted a few slides from a presentation by Kashem Muttaqi, originally found at http://egpreston.com/VoltageRegulation.pdf , below. The slides explain how we get to the approximate formula.

(This was found on the third page of a search for voltage drop pr qx - apparently pr qx is a fairly unique "phrase".)