0
\$\begingroup\$

I need to find the transfer function of two RC circuits connected together.

This is the schematic: Circuit

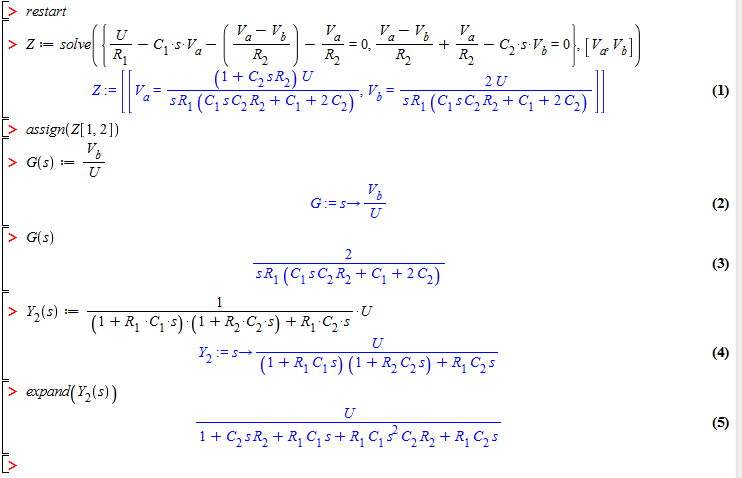
Here is how I tried solving it, using maple, the two equations for nodal tensions are found at (1) as the solve({eq1, eq2}) parameters: Solution attempt

eq1 is the sum of currents at node A, eq2 is the sum of currents at node B.

Well my problem is that I get a result at (3) that is not the answer from the solutions booklet (which is at (4)).

I am quite rusty into solving circuits, could anyone take the time to explain me how to get to the correct answer? I must find the transfer function \$ G(s) = Y_{2}(s)/U_{1}(s) \$. \$ u_{1}(t) \$ is a voltage excitation, and \$ u_{2}(t) \$ is the same voltage as \$ V_{C_{1}}(t) \$.

Thanks!

P.S. If anyone could tell me what I did wrong in nodal analysis it would be great.

\$\endgroup\$
6
  • 2
    \$\begingroup\$ There is at least one mistake in first equation (node A). According to your sign conventions it should be \$(U-V_a)/R_1-...\$ and not \$V_a/R_1-...\$ \$\endgroup\$
    – Roger C.
    Commented Oct 30, 2015 at 21:54
  • \$\begingroup\$ @RespawnedFluff Circuit isn't exactly the same, there's no controlled source there, question differs. \$\endgroup\$
    – Yannick
    Commented Oct 30, 2015 at 22:22
  • \$\begingroup\$ @RogerC.Thanks for sharing, it indeed makes it "nearer" than the solution, but it still doesn't give the same equation as the solution. Not sure if I'm just too tired to see an obvious mistake... \$\endgroup\$
    – Yannick
    Commented Oct 30, 2015 at 22:23
  • \$\begingroup\$ What are your controlled sources? I don't see them in the circuit. While the other question may differ, the top-voted answer solves your problem too. Do you realize that if you eliminate "U" from your (5) you basically have the transfer function? \$\endgroup\$
    – got trolled too much this week
    Commented Oct 30, 2015 at 22:24
  • \$\begingroup\$ u2(t) is afaik a "controlled controlled" source as it has a gain of 1, voltage of 1 * Vc1. No I don't realize anything to be honest at this hour. All I wish is to find why I have failed at the nodal analysis. I could care less if there's another question out there that's similar to mine. The eq at (5) is the solution given to me, I wish to know how I can get to there, as explained already in my question. \$\endgroup\$
    – Yannick
    Commented Oct 30, 2015 at 22:38

0

Reset to default