1
\$\begingroup\$

As far as I understand, the characteristic impedance matters when a signal is oscillating since the BNC coaxial cable can be modeled as having inductors and capacitors as in the figure:

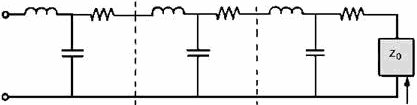
enter image description here

I have couple of questions that confused me:

1-)I'm wondering if I measure a low frequency oscillating signal like 100Hz through a BNC cable with a length "X", how can I calculate the voltage drop across the BNC cable. Ok maybe my signal is not an RF signal, but if I'm after accuracy and I guess the signal voltage will attenuate some amount since it is oscillating right? Any ideas how to calculate the voltage drop?

2-)And how come different lengths of BNC cables have 50 or 75ohm fixed characteristic impedances? Isnt the length of BNC effect its characteristic impedance?

\$\endgroup\$

2 Answers 2

Reset to default
3
\$\begingroup\$

The characteristic impedance of a cable is this mathematically: -

\$Z_0 = \sqrt{\dfrac{R + jwL}{G + jwC}}\$

Where

  • R is the series ohms per metre
  • G is the parallel conductivity per metre
  • L is the series inductance per metre
  • C is the parallel capacitance per metre

It has nothing to do with the overall length of the cable. It has everything to do with the ratios of the parameters listed in the equation above.

Simplifications exist - at above about 1MHz you can say that inductive reactance dominates series resistance and capacitive effects dominate the leakage conductance. This makes the equation: -

\$Z_0 = \sqrt{\dfrac{L}{C}}\$

If you are testing at 100 Hz you won't get any sensible indication of characteristic impedance. In fact at 100 Hz it makes no sense to terminate the cable at all.

If you want to know how characteristic impedance is determined try a few experiments using this website: -

enter image description here

If you are intent on understanding the attenuation at 100 Hz then use only R and C and, because standing waves will be virtually non-existent, you can consider R and C as lumped parameters equivalent to the whole length of the cable.

For instance, find a coax spec and look-up the capacitance per unit length and resistance per unit length and convert to equivalent values for your cable length. Basically you get an RC low pass filter with maybe 2 ohm and 1nF (numbers off the top of my head for 10m of cable).

Ask your self how much attenuation is going to happen at 100 Hz. Not much is my impression because the basic cut-off frequency is 79 MHz but, for a cable that is 1 km long, R might be 200 ohms and C might be 200nF and the cut-off frequency will be more like 2 kHz and you will start to see 100 Hz sinewaves being attenuated a little bit.

\$\endgroup\$
6
  • \$\begingroup\$ you wrote: "you won't get any sensible indication". how would you prove it by a formula? how would you show the voltage drop at 100Hz is almost zero mathematically with a formula? that was what I was asking. \$\endgroup\$
    – user16307
    Commented Nov 17, 2015 at 11:35
  • \$\begingroup\$ See my additions. \$\endgroup\$
    – Andy aka
    Commented Nov 17, 2015 at 11:42
  • \$\begingroup\$ great answer! so model as rc and calculate. that was what I was curious about. just one more thing. can you give me a hint why standing waves wouldn't exist and we can neglect inductor effect when modelling at 100Hz low freq? \$\endgroup\$
    – user16307
    Commented Nov 17, 2015 at 11:49
  • \$\begingroup\$ Standing waves are only important when the wavelength of the lowest frequency approaches one-tenth the length of the cable. Wavelength of 100 Hz is 3000 km and I'd start to think of considering worrying about standing waves if the cable was 300 km or longer. However, given that the dominant impedances are R and C at these low frequencies, even if the cable were very long, standing waves are going to be a minor issue due to the overall losses at 300 km+ \$\endgroup\$
    – Andy aka
    Commented Nov 17, 2015 at 11:54
  • \$\begingroup\$ oh i see. btw your answers brings the importance of straightness of the cable as well. imagine 200m cable which is rolled like a spiral on a Cable Reel. this would increase inductance right? something like this:encrypted-tbn2.gstatic.com/… \$\endgroup\$
    – user16307
    Commented Nov 17, 2015 at 12:00
1
\$\begingroup\$

First: signals are not "oscillating" through a cable. There IS NO oscillation ! Oscillation means that it generates a signal by itself. That would make a very lousy cable because it would add its own signal to my wanted signal !

The inductors and capacitors model the behavior of the cable, they attenuate the signal but there is no oscillation going on. For an oscillation to be present there would need to be an active component present that can add energy to the oscillation. In a cable, there is no active element !

1) A low frequency signal like 100 Hz will only be affected by the resistance of the coax cable. So just measure the series resistance and take that into account. And again: NO OSCILLATIONS !

2) The (50 or 75 ohm) characteristic impedance is a property of the cable itself. It is also INDEPENDENT of the LENGTH of the cable ! It is NOT something you can measure with an ohm meter ! The cable is a transmission line (read more here) and it will transport a signal properly ONLY when the input and output of the cable/transmission line is TERMINATED by the proper impedance ! So a 50 ohm coax cable needs a source with a 50 ohm output impedance and it needs a 50 ohm load at the other end of the cable. If this is not the case (no proper termination) then the signals will reflect and be garbled up !

\$\endgroup\$
12
  • \$\begingroup\$ The signal is coming from a signal generator which is 100Hz sinusoid. Thats what I meant. You wrote: " A low frequency signal like 100 Hz will only be affected by the resistance of the coax cable" This is just a neglection because the voltage drop would be very small but not zero. But how would you calculate it theoretically? I hope you get my question now. \$\endgroup\$
    – user16307
    Commented Nov 17, 2015 at 11:24
  • \$\begingroup\$ "This is just a neglection because the voltage drop would be very small but not zero" Oh yes it can be zero ! You cannot say anything about the voltage drop unless you specify the source impedance and the load impedance. If you leave the output side of the cable open (infinite load impedance) there would be ZERO voltage drop ! Why ? Because no current flows so no voltage is dropped ! Leaving the output open (no termination) is OK for a 100 Hz signal as is confirmed by Andy's answer. I just assumed this from my experience. \$\endgroup\$
    – Bimpelrekkie
    Commented Nov 17, 2015 at 12:38
  • \$\begingroup\$ if it was pure resistive, the current would not drawn because the input impedance of the DAQ or a scope is extremely high. but in case RC filter behaivour this doesnt hold. \$\endgroup\$
    – user16307
    Commented Nov 17, 2015 at 12:45
  • \$\begingroup\$ Why not ? In theory you are correct but in practice, will it be a problem ? Think about the wavelength of your 100 Hz signal and the length of the cable as in Andy's answer. \$\endgroup\$
    – Bimpelrekkie
    Commented Nov 17, 2015 at 12:47
  • \$\begingroup\$ no my question is about to prove it mathematically by a formula. Andy's answer is satisfactory. \$\endgroup\$
    – user16307
    Commented Nov 17, 2015 at 12:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.