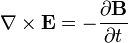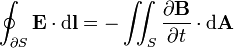Why voltage lags by 90° from magnetic flux?

Question

I was not sure if I should write it here or on Physics board, but I will give a try.

As I understood current (generally emf) can be generated in a system by varying magnetic flux.
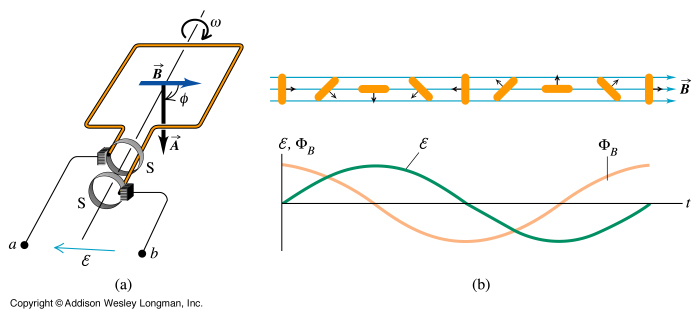
For example, let's take AC generator. A loop of wire rotates between N and S magnets.

\$ \Phi = B \times A \times cos(\Omega)\$, where \$ cos(\Omega)\$ is the angle between the magnetic field B and normal of the loop A.

So the maximum flux can be achieved when the normal of the loop is parallel with magnetic field. But why emf at that moment is 0? And why emf is max when flux is 0?

Answer 1

The rate of change of flux linkage is equal to the emf.

So it's your above equation should be differentiated with the subject of time. In that case you will get a sine equation. So that's the reason.

when you considering the flux linkage, Flux Linkage = << note the additional N there.

\$ B \times A \times N \times cos(\Omega)\$

if we took omega as angular velocity , then \$ \Omega \times t \$ is the angle relative to the starting position. So differentiate it in the subject of 't'.

In your sine train you could see clearly the rate of change of flux[gradient of flux]is max when angle is 90 deg. So that's the reason.

Answer 2

Actually, that is incorrect. The maximum flux is the point where the magnetic field is flowing through the CENTER of the loop (i.e., pushing through the hole)

When talking about the normal of a loop we are talking about the normal of the surface (imaginary surface) area of the loop which points NORMAL the 2D plane of that surface area.

as for the EMF,

EMF = -d(flux)/dt 

Where the Flux = BA*cos(w*t) (remember w = Omega) (w*t = theta...lets call it theta mod 360)

the emf = w*B*A*sin(w*t); 

so when the Normal of the surface area and the magnetic field are parallel that means

theta = (w*t mod 360) = 0  
sin(theta) = 0

and the Flux is at a maximum because

cos(theta) =  1 where theta = 0

From this you can see that the when the Flux is at a peak the abs(EMF) is at the smalest value. (I mention Absolute value because the signal shifts between positive and negative of equal magnitude)

Answer 3

That 90º lag comes from the derivative with respect to time in the Faraday's law of induction:

(Differential form)

(Integral form)

The left hand side of this last equation is the EMF. If those equations didn't have a derivate with respect to time, |EMF(t)| would be maximum when the apparent |B(t)| was maximum, but the (negative) derivative causes the sin(t) that there would be in EMF(t) to turn into a -cos(t), and that is where the 90º lag comes from.