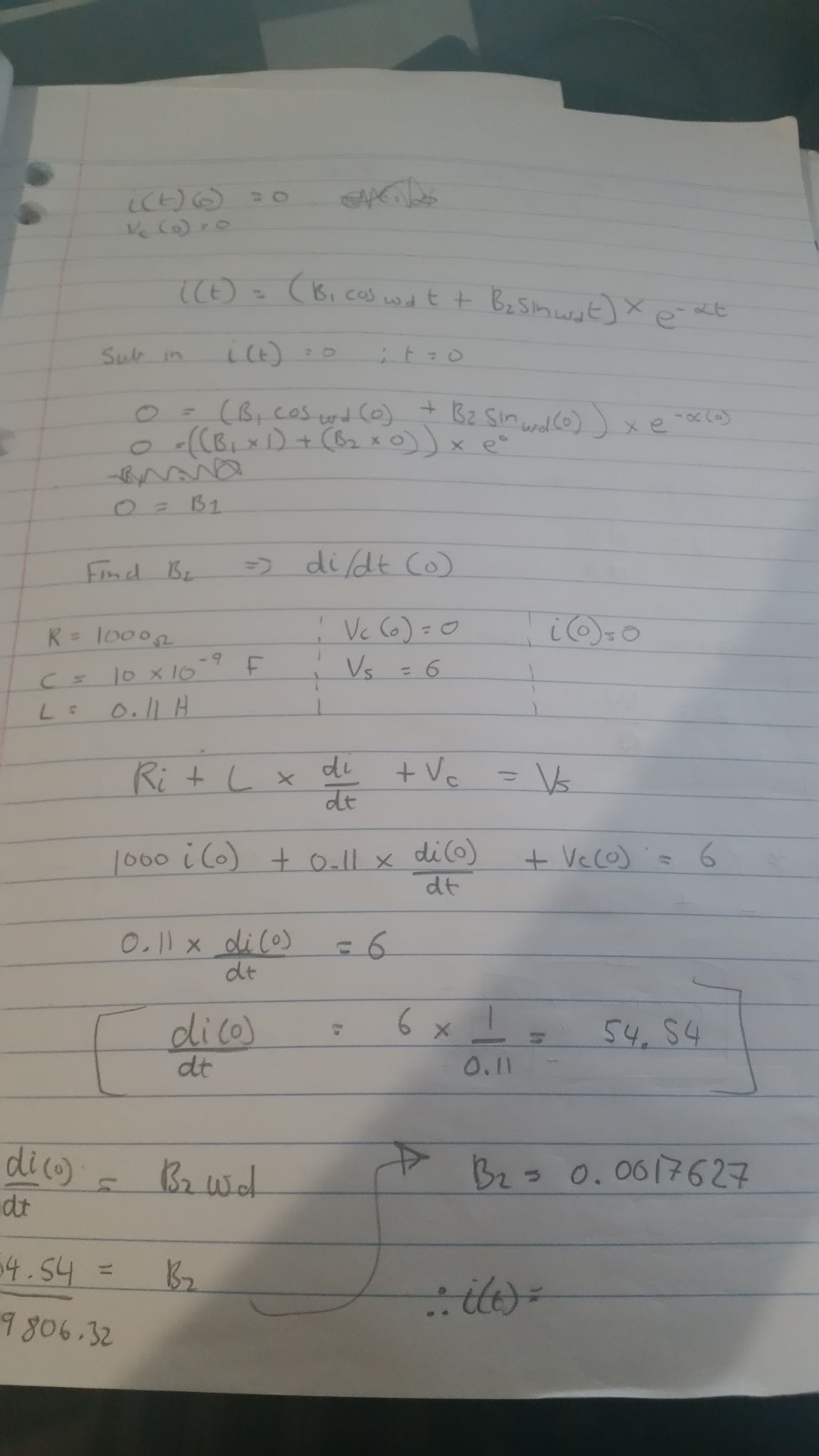You messed up with the math. I don't fully follow, what you are doing, it doesn't seem totally wrong, but you certainly miss some stuff.
First of all: you get a non-damped solution with your calculations. This is wrong, the math and the physics require you to find a solution, that damps down over time, till your voltage and current exponentially decrease to zero.
Secondly: As far as I get your notes, the capacitance has never been used.
This is something you certainly need!
Thirdly: Your exponential term gets lost in the beginning, which is wrong, you will need it.
Solution:
Follow the stuff described here from page 29 is exactly what you're looking for.
In case you want to do it yourself you should write the problem down as an ordinary differential equation of order two, and view the problem from a mathematical point of view.
Then you use
$$
A_1e^{s_1t}+A_2e^{s_2t}
$$
(as in the paper I referred you to.)
$s_1,s_2$ are most likely complex numbers, resulting in a sin/cos and exponential solution.
I tried to do that when I started this answer, then I deleted it because it became very tiresome. However, nearly no good explanation has been found. Most stuff looks ugly, and is poorly explained, the link above is the best I found.
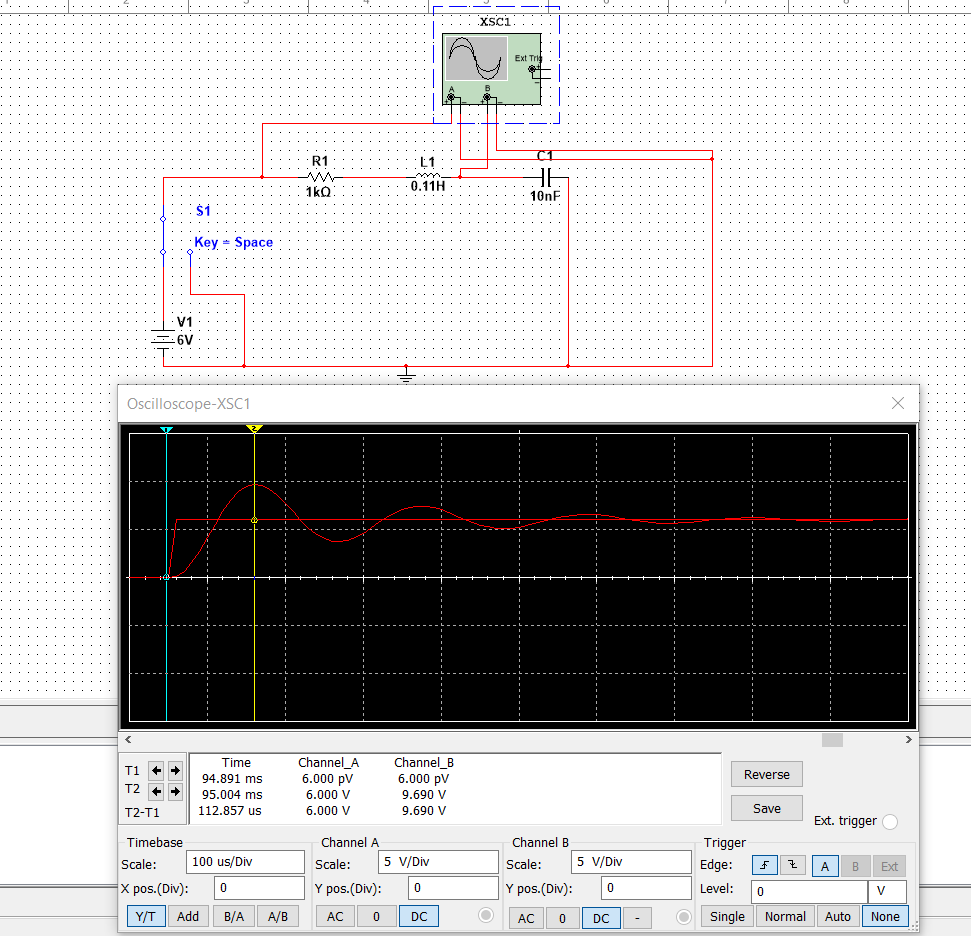 When I simulate the circuit, the values obtained from my v(t) expression do not match the simulated circuit results.
When I simulate the circuit, the values obtained from my v(t) expression do not match the simulated circuit results.