I am trying to derive the input impedance for the transresistance amplifier (shunt feedback) on page 119 in the Art of Electronics.
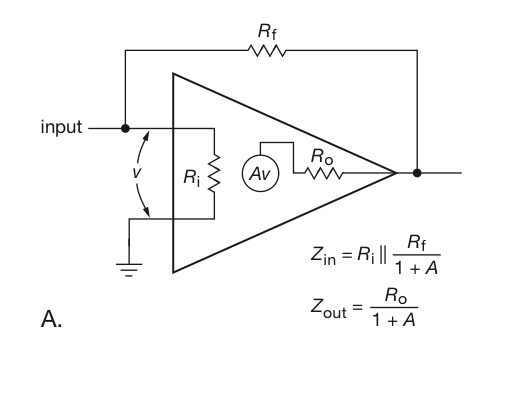
I am considering a voltage \$v_i\$ applied to the input. As described in the book, it is the normal input impedance \$R_i\$ in parallel with something else due to feedback. When \$v_i\$ is applied, it causes a current $$I=v_i(1-A)/(R_f+R_o)$$ to flow from the input (so there is really current flowing into the input with A>1).
So shouldn’t the parallel part of the input impedance be $$v_i/I=(R_f+R_o)/(1-A)$$ rather than what is shown in the image? This is a negative impedance in parallel with \$R_i\$.
