Feedback impact of collector-base shunt resistor in common emitter amp stage

Question

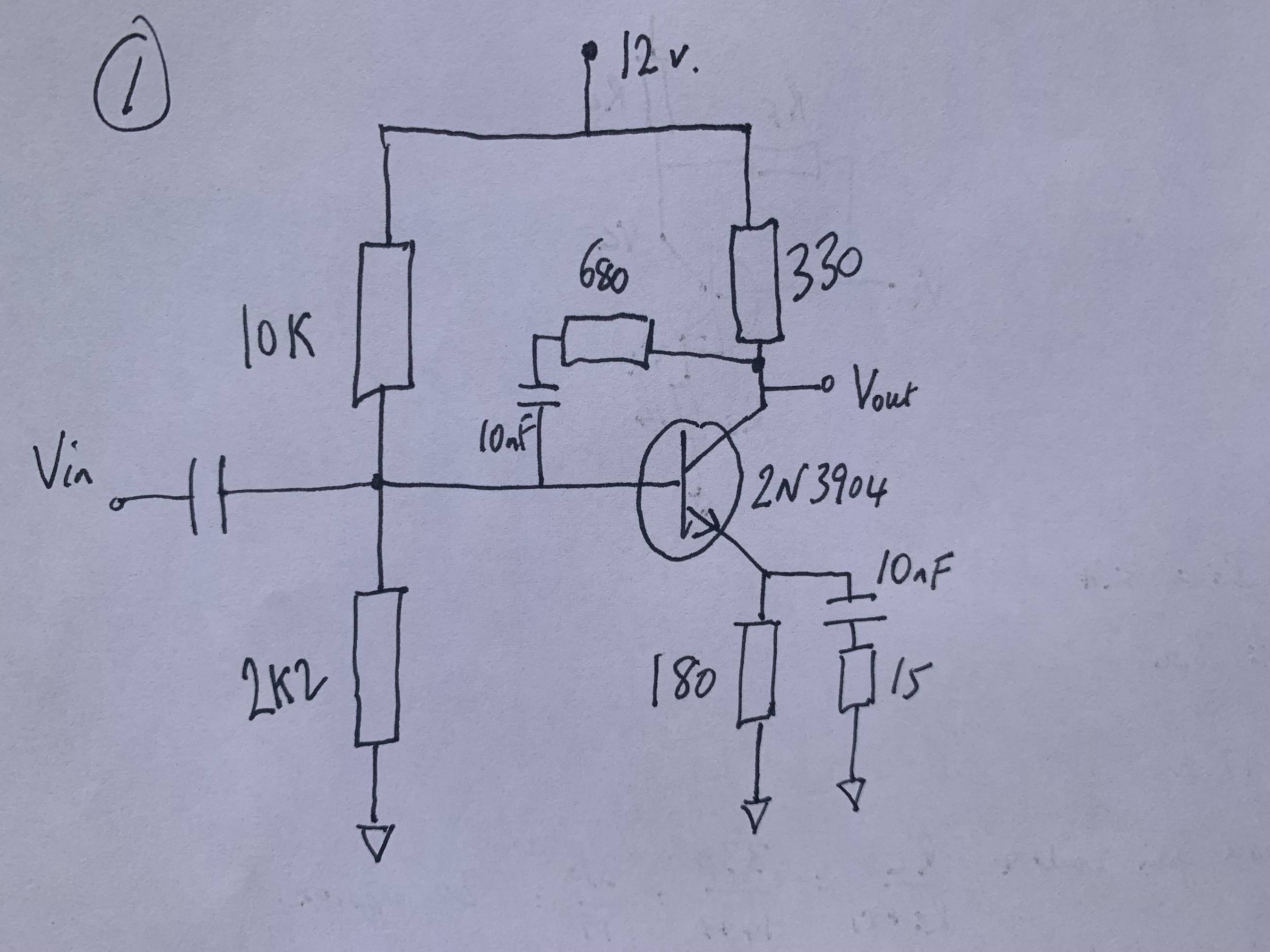
I made this 3 transistor Rf amp which works well at 10 MHz and has 50 ohm output impedance, a gain of 8.2 (18.3 dB) and quiescent curent of 28.6 mA. It consists of a common emitter stage followed by 2 emitter follower stages. (Credit: Nick the vic M0NTV)

My question concerns only the first stage and, in particular the 680 Ohm feedback shunt resistor between collector and base.

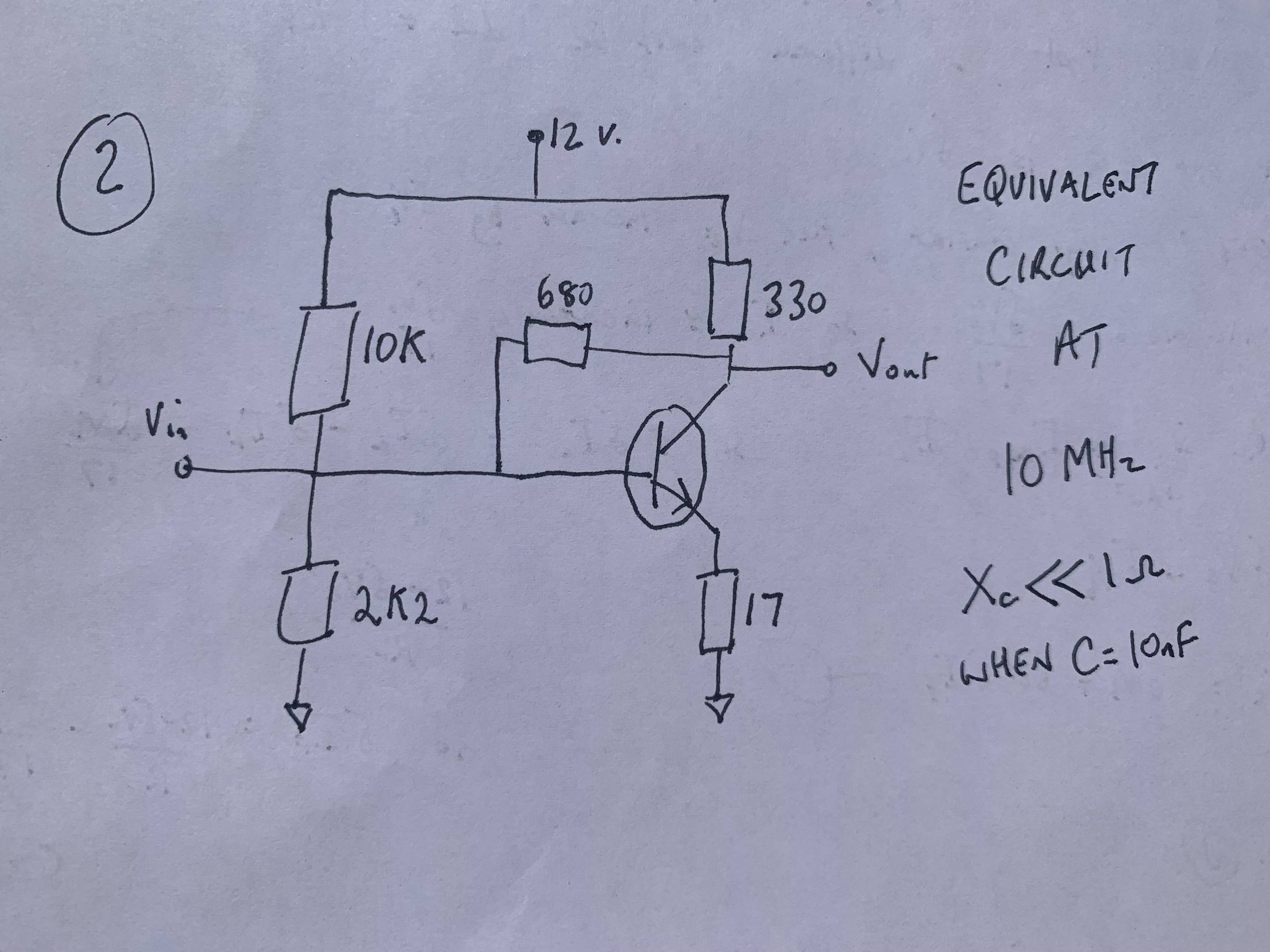
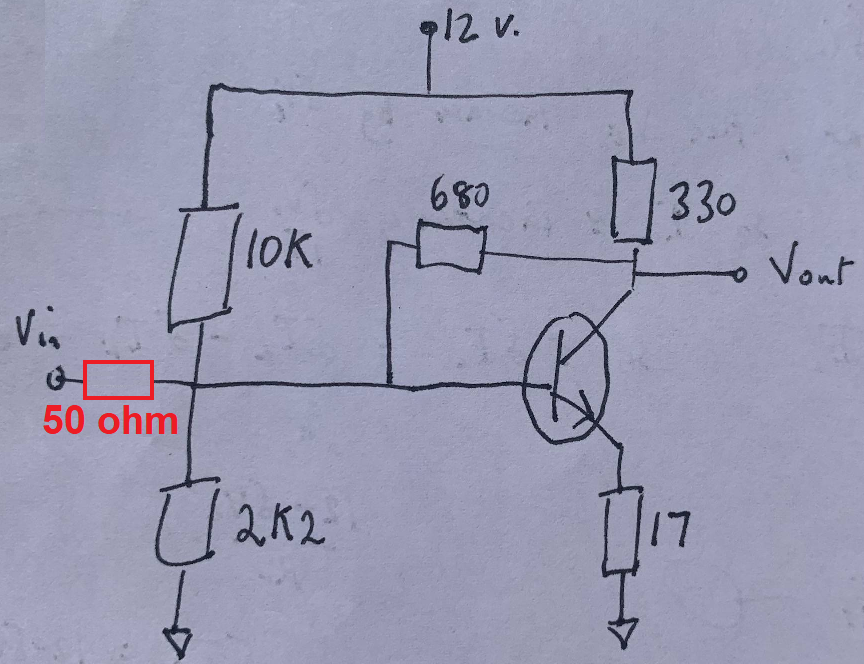
At 10 MHz the reactance of a 10 nF capacitor is <<1 Ohm so, as far as the signal is concerned, all capacitors can be replaced by a short. This results in having 2 emitter resistors in parallel (180 & 15) which make us 14 Ohms together. To this I have added the internal emitter resistance of re = 3 Ohms (25 mV divided by the 8 mA emitter current). This gives the simplified circuit:

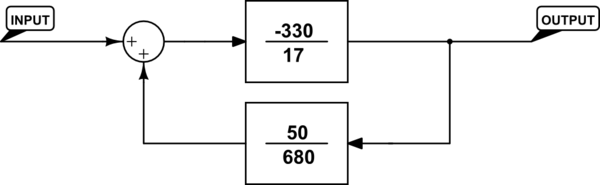
If I'm correct, but for the feedback resistor the gain would be approx 330/17 i.e. 19.4 or 25.9 dB (Rl divided by RE total). So the effect of introducing the feedback resistor is to halve the gain.

I've tried doing the calculation considering the effect of a small dV increase in Vb to work out the gain Av = dVc/dVb but end up going round in circles. Going to LTSpice might give the answer but would add nothing to my understanding.

Can someone help me out?

Answer 1

You need to take the source impedance into account to calculate this correctly. I'm assuming that the input source is meant to have a 50 Ω impedance. Hence I'd visualize it like this: -

I'd then analyse it like an amplifier with an open-loop gain of \$\frac{-330}{17}\$: -

^{simulate this circuit – Schematic created using CircuitLab}

If I call the output Y and the input X, the signal after the summing node (Z) is: -

$$Z = X + Y\cdot\dfrac{50}{680}$$

Then we know that Z multiplied by -330/17 = Y: -

$$Y = \left(X + Y\cdot\dfrac{50}{680}\right)\cdot\dfrac{-330}{17}$$

Hence: -

$$Y\left(1 + \dfrac{16500}{11560}\right) = X\cdot\dfrac{-330}{17}$$

Or,

$$Y = X\cdot\dfrac{-19.412}{\left(1 + \dfrac{16500}{11560}\right)}$$

Can someone help me out?

Chew on it a tad more and the gain (Y/X) comes out at -7.997.