I am trying to understand the Friis transmission equation. My scenario: I consider transmit antennas (\$T_x\$) and receive antennas (\$R_x\$) at a distance R [km]. f [GHz], \$G_{T_x}\$ and \$G_{R_x}\$ in dBi, \$L_a\$ in dB ( loss) (<0). \$G_{R_x}\$ =0 that is why I would like to make a calculation in logarithmical scale. Friis equation: \$P_r=P_tG_tG_r(\lambda/4\pi R)^2L_a\$
and then I wrote in log scale:
\$P_r(dB)=P_t(dB)+G_t(dB)+G_r(dB) + 10 log(\lambda/4\pi R)^2 + 10log(L_a)\$
In Fundamentals of Digital Communication by Upamanyu Madhow, p 134
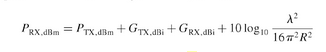
- Why is G in log scale in dBi ? I thought that dBi is in log scale dB
- \$L_a\$ in dB, in log scale is it also in dB?
