Why adding capacitor to a 10x passive oscilloscope probe

Question

I'm trying to understanding input impedance and its circuitry of a simple, passive oscilloscope 10x probe. From reading Input Impedance of an Oscilloscope and the video EEVblog #453 - Mysteries of x1 Oscilloscope Probes Revealed, I don't understanding why we add these capacitors in the probe circuitry and make things more complicated. The point is to add enough impedance to a passive probe to minimize loading effect of the circuit being measured. A big enough resistor will do and the with just resistors, voltage divider works the same for both AD and DC source regardless of frequencies. If there are intrinsic reactances in the wiring, then can't we just add a big enough resistor in series with those to make any reactance negligible?

Answer 1

I don't understanding why we add these capacitors in the probe circuitry and make things more complicated.

They make things far less complicated. The low-pass filter that would otherwise occur is eliminated.

The point is to add enough impedance to a passive probe to minimize loading effect of the circuit being measured. A big enough resistor will do and the with just resistors, ...

No. Increasing the probe resistance decreases the high-pass cut-off as it will be proportional to \$ \frac 1 {RC} \$.

... voltage divider works the same for both AD and DC source regardless of frequencies.

No. You have omitted the effect of the scope's input capacitance.

If there are intrinsic reactances in the wiring, then can't we just add a big enough resistor in series with those to make any reactance negligible?

Increasing the resistance decreases the signal available to the 'scope to the point that you won't be able to get high enough resolution and ADC noise will become a problem.

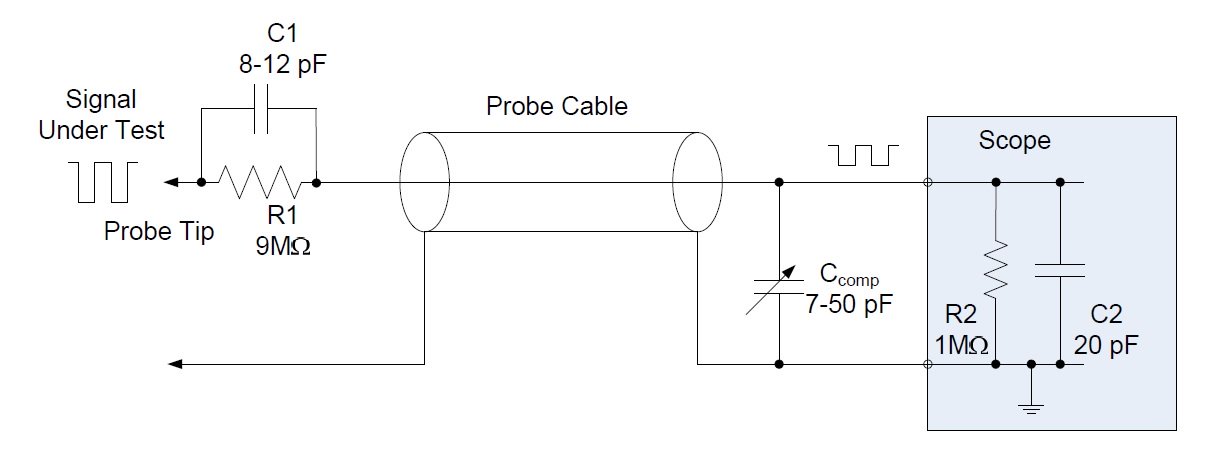
Figure 1. From Introduction to oscilloscope probes.

The trick is to use two potential dividers; one resistive and one capacitive. Since we've got a ratio of 9:1 with the resistive divider we need to do the same with the capacitive divider. Remember that the capacitors impedance is given by \$ Z_C = \frac 1 {2\pi fC} \$ so $$ \frac {R_1}{R_2} = \frac 9 1 = \frac {Z_{C1}}{Z_{C2}} = \frac {\frac 1 {2\pi fC_1}} {\frac 1 {2\pi fC_2}} = \frac {C_2}{C_1}$$

From this we get \$ C_2 = 9C_1 \$. With the values shown in Figure 1 we can just about achieve this if C1 is 8 pF and C_COMP is wound up to the max to give us 72 pF total (although this model omits the cable capacitance so we have room to spare).

Answer 2

The cable has capacitance, and the scope input has also capacitance. If you add resistance, it would just reduce the bandwidth.

That's why you add capacitance to the probe, to nullify the other capacitances.

Basically it's the same thing with the resistances and the capacitances. If you want a 10x probe, and scopes have 1M resistance, you add 9M so that it divides DC voltages by 10. If you want also the AC waveforms to attenuate by 10x, and you already have some capacitances in the system, you select a correct capacitance to get 10x attenuation.

Answer 3

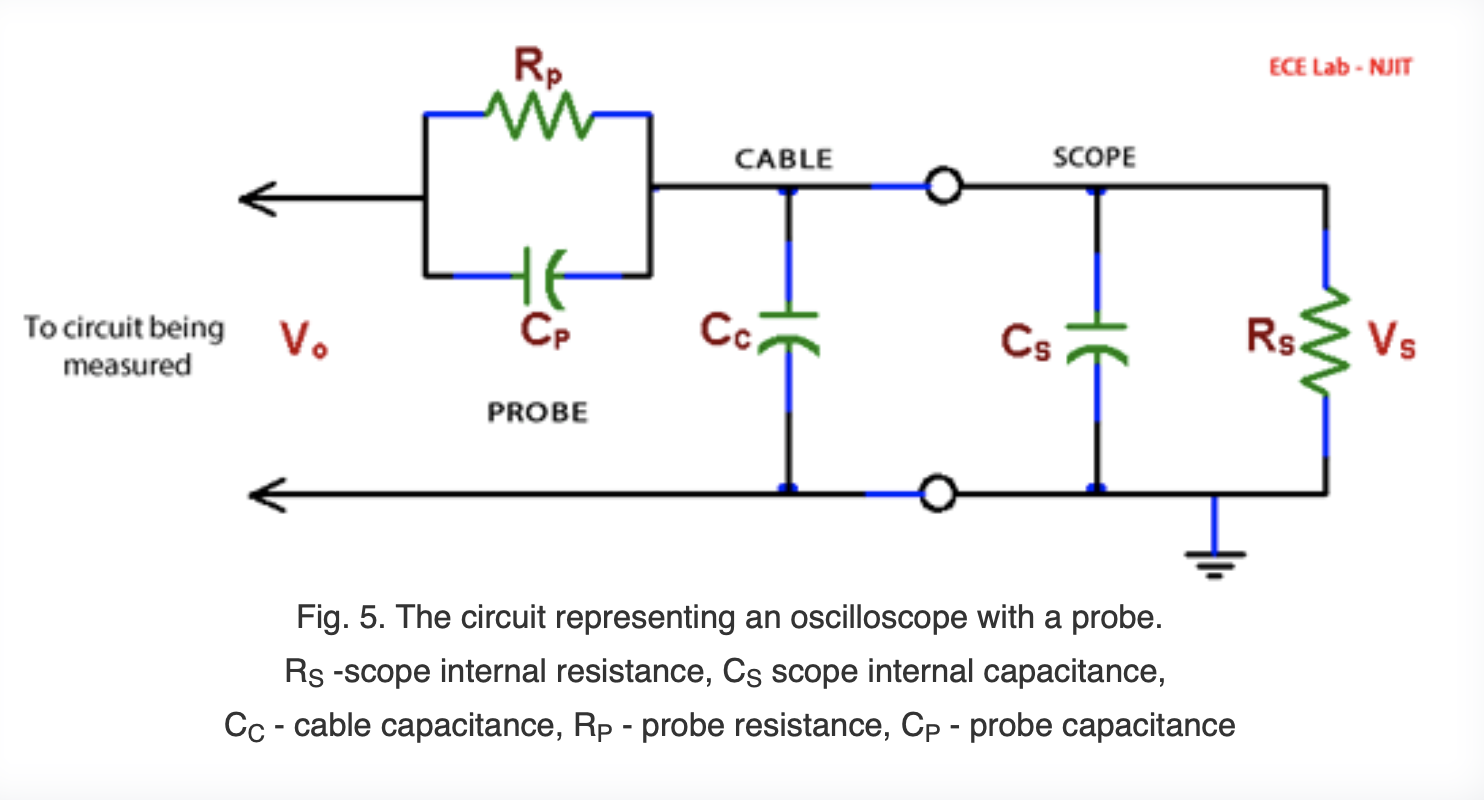
You cannot take off Cs nor Cc. Together with Rp they would make a RC lowpass filter. Elementary impedance calculations reveal that the low-pass filtering effect can be compensated by adding Cp. You can consider it a high frequency boost. The total loading of the circuit under measurements is still lighter than without the probe.

General rule for flattest frequency response: RpCp=Rs(Cc+Cs) That's useful as long as the cable is short enough. As the frequency grows the user must finally take into the account that the cable is actually a transmission line which is badly matched at both ends. The compensation becomes gradually worse as the frequency grows. There's no strict limit when this happens.