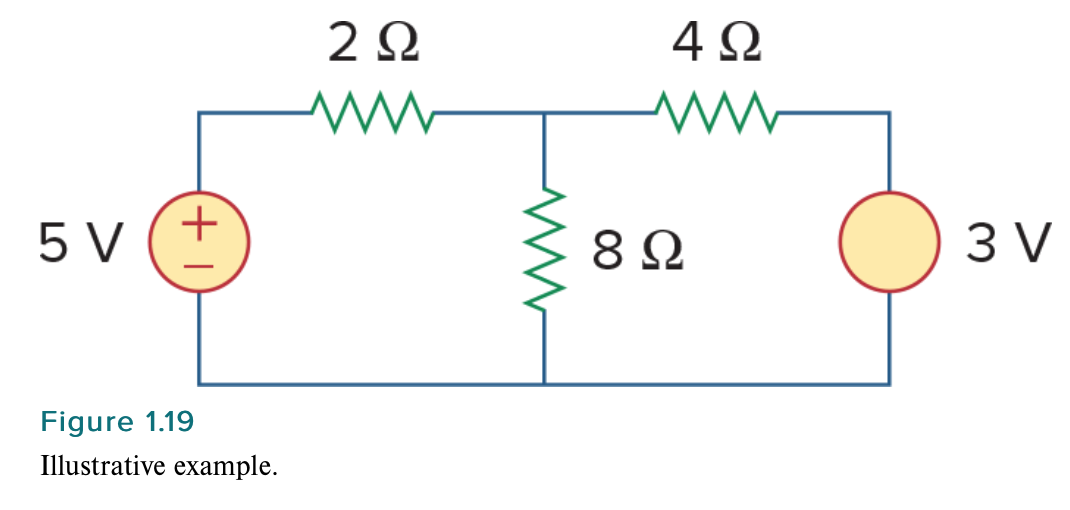
I will further develop Spehro Pefhany's intuitive explanations with some interesting observations on this configuration that is widely used in electronic circuits (with resistors having higher resistance).
This approach has the advantage over the mechanical use of formal methods of analysis because it aids understanding and makes a connection between simple electrical circuits and their more sophisticated applications in electronics.
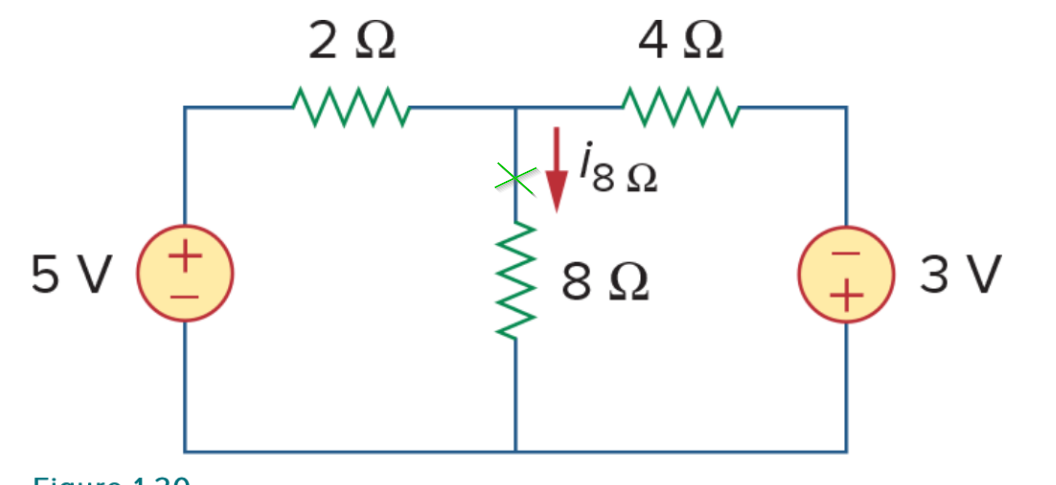
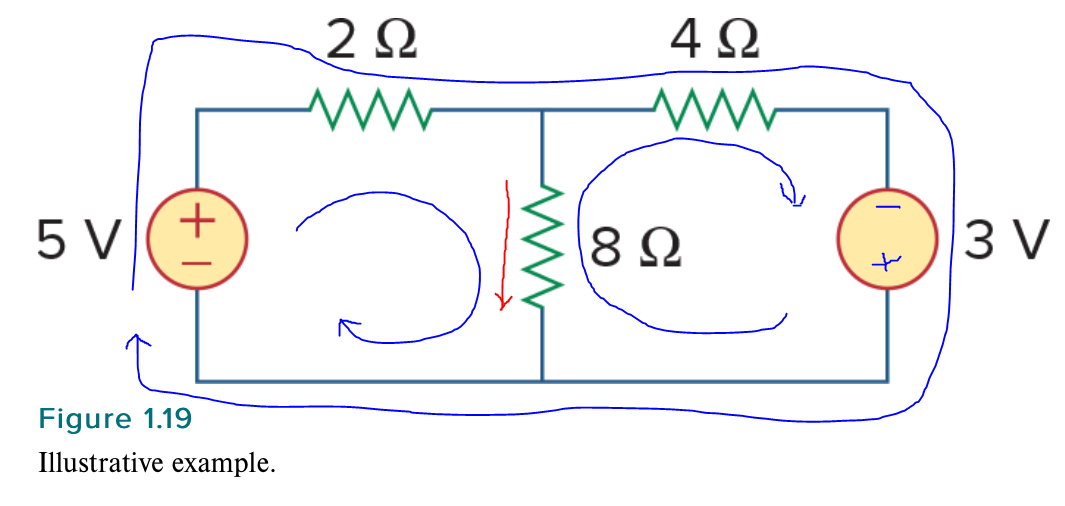
As Spehro said, the task is to find the voltage of the upper node relative to the lower node (ground). This will automatically show the current direction through the 8 ohm resistor. So, we can remove it and consider only the 2-resistor network.
"Pull-up" and "pull-down" resistors in series
Thus we get the famous circuit arrangement used in op-amp inverting amplifiers that consists of two voltage sources with opposite polarity connected through resistors to a common point. Each of them struggles to change the node voltage in its direction. Figuratively speaking, like in the game of "tug of war", the left (+5V) source "pulls" the common point up while the right (-3V) source "pulls" it down.
If the sources have the same voltage and the resistors have the same resistance, the voltage at the common point will be zero (the so-called "virtual ground"). Let's label the left voltage source with V1 and the right one with V2, the left resistor with R1 and the right one with R2. Then V1 will appear across R1 and V2 will appear across R2… and the same current I = V1/R1 = V2/R2 will flow through this circuit of four elements (V1, R1, R2 and V2) in a loop.
Current source and current sink in series
Thus the combination of the left two elements (V1 and R1) in series acts as a current source that sources a current I1 = V1/R1 and the combination of the right two elements (V2 and R2) in series acts as a current sink that sinks a current I2 = V2/R2… and I1 = I2.
Now, if we increase V1 so V1 > V2 or we decrease R1 so R1 < R2 (the OP's case), the left source will try to source higher current than the right source can sink. As a result, because it is impossible for a current in a circuit of series-connected elements to be different, the voltage of the common point will become positive… and, if we reconnect the 8 ohm resistor, a current will flow downwards through it...
2-input voltage divider
From another viewpoint, the two resistors in series form a more sophisticated voltage divider with two inputs. It can be controlled both from the left thus producing a partial voltage V+ = V1.R2/(R1 + R2) and from the right thus producing a partial voltage V- = V2.R1/(R1 + R2). Since the denominators of the two fractions are the same, we can compare only the products. So, we see that V1.R2 > V2.R1, i.e., the node voltage is positive.
Voltage summer with weighted inputs
This is a good illustration of the superposition principle that shows this circuit sums two voltages with weighted coefficients K1 = R2/(R1 + R2) and K2 = R1/(R1 + R2) . So it acts as a voltage summer with weighted inputs. We see that K1 > K2 and draw a conclusion that the node voltage is positive.
Bridge circuit
A more sophisticated intuitive approach is to think of this circuit as of a kind of bridge. In the simplest case, if V1 = V2 and R1 = R2, the "bridge" is balanced and the node voltage is zero. When the values of voltages and resistances change, the bridge becomes unbalanced and a node voltage appears.
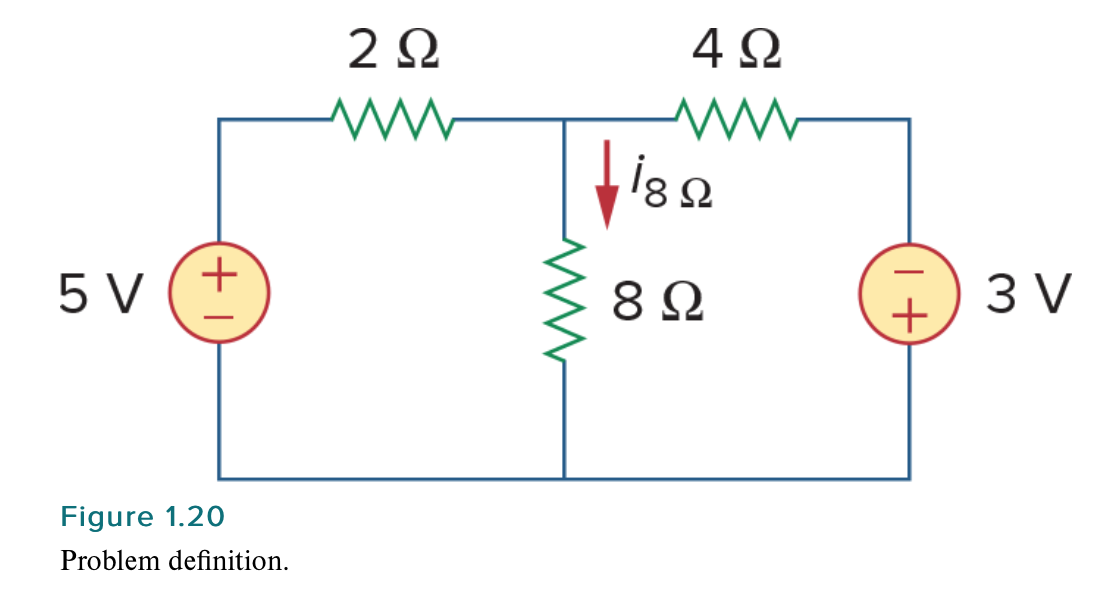
 How does one know that the current is flowing downwards through the \$8 \ \Omega\$ resistor? This seems to be the immediate assumption, but there's no explanation from the authors as to why this is the case.
How does one know that the current is flowing downwards through the \$8 \ \Omega\$ resistor? This seems to be the immediate assumption, but there's no explanation from the authors as to why this is the case.