What is the direct coupling problem which differential amplifiers help avert?

Question

The discussion below comes from Chapter 3.5 of Analysis and Design of Analog Integrated Circuits by Gray, Hurst, Meyer, and Lewis.

In particular, I do not follow what is meant when they write

This property allows direct coupling of cascaded stages without offsets.

I understand the property, but I do not understand why the property allows direct coupling of consecutive stages. Perhaps someone can provide an example that might show how a regular (?) stage does not allow for direct coupling but a differential stage does?

Answer 1

Because a decently designed differential amplifier will have very little (or near-zero) common-mode gain (irrespective of a high differential gain), you can attach the differential outputs from one stage to another differential stage's inputs without great worries of the 2nd stage transistors becoming saturated.

This can only happen with differential stages because the common-mode gain is near-zero. A non-differential input has only gain and cannot benefit from any common-mode cancellation.

For instance, imagine a single-ended amplifier with a gain of ten having a nominal output voltage of half the supply rails when the input is 0 volts. You could not connect this directly to another similar stage because that extra stage wants to see 0 volts as a neutral input but, the first stage is supplying it with 6 volts. In other words it would hit the end-stops.

On the other hand you could engineer a single-ended input that produced 0 volts out with 0 volts in then, you could cascade multiple stages but, you then introduce a problem hidden in the first example; your common 0 volt line is likely subject to all sorts of noise currents from other parts of the circuit and, the final result would be a noisy output.

Not so with differential (and impedance balanced) circuits; using differential signal lines means no shared noise with other system components and, any noise that does come along (such as EMI) would affect both simultaneously and, because the CM gain is near-zero, that noise would hardly influence the output differential voltage.

Answer 2

Basic idea

Single-ended configuration

In direct-coupled amplifier stages, the output voltage of the previous stage is a sum of the amplified AC voltage and the quiescent DC voltage. This composite AC+DC output voltage should be “shifted down” almost to ground level so that the input voltage of the next stage “wiggles” around the zero level. This actually means that a DC voltage equal to the quiescent DC voltage must be subtracted from the composite AC+DC output voltage so that only the AC voltage remains.

Also, under the influence of temperature, the quiescent output voltage and the shifting voltage slightly vary. To be compensated, these changes must be equal and opposite. But in this “single-ended configuration” the two voltages are not under the same conditions - the output voltage is referenced to ground and the shifting voltage is floating; this makes compensation difficult.

Differential configuration

In contrast to the single-ended configuration, here, to make perfect compensation, the two DC voltages are exactly the same and both referenced to ground. However, the resulting AC voltage is "floating" and requires a differential input to the next stage. Note that the “shifting" DC voltage is introduced by another input voltage source which determines the behavior of the former.

Figuratively speaking, in common mode the differential stage “lifts" its internal ground (the common emitter point) to the level of the two output voltages of the previous stage, and in differential mode, these voltages "wiggle" around this "shifted ground". So, the clever trick on which the differential amplifier is based is that in common mode its "internal ground" is not fixed but "movable", following.

Conceptual circuits

We can illustrate the ideas above using simple equivalent electrical circuits.

Immovable real ground

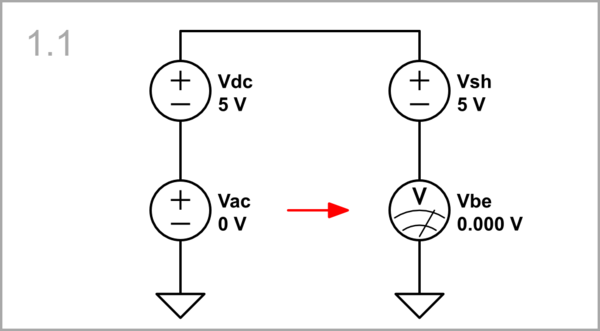
Let's first consider cascading two single-ended stages. The output of the previous stage is represented as two voltage sources (5 V quiescent voltage Vdc and input voltage Vac), connected in series. The "shifting" voltage source Vsh is connected oppositely and in series to Vdc so that its voltage is subtracted. The resulting voltage appears across the voltmeter Vbe (its voltage is decreased to 1 kΩ to roughly resemble a base-emitter junction).

Vac = 0: In the initial case, Vsh compensates Vdc, and the Vac zero voltage appears across Vbe.

^{simulate this circuit – Schematic created using CircuitLab}

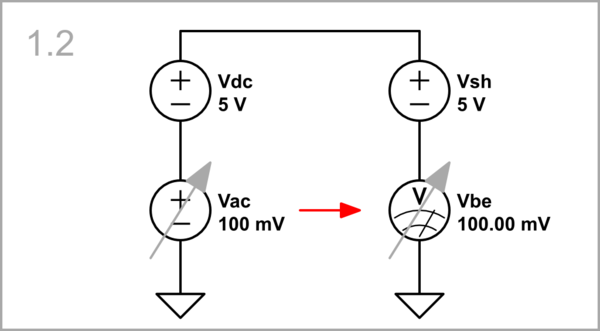
Vac = 100 mV: When Vac slightly increases above Vdc...

^{simulate this circuit}

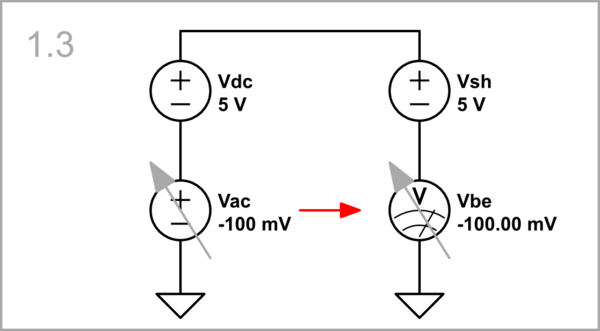
Vac = -100 mV: ... or decreases below Vdc...

^{simulate this circuit}

... its variations appear shifted down across Vbe (Vac "wiggles" around ground).

The disadvantage of this single-ended DC coupling is that the Vdc and Vsh variations act as an input signal and are amplified by the next stage (try it by slightly changing Vdc and Vsh, and looking at Vbe). Let's see how we can solve this problem.

Immovable virtual ground

With the same success we can put Vsh below Vbe instead above (i.e. to attach it to the next stage). It actually means to "lift" the ground with Vdc.

Vac = 0, Vmg = 5 V: If we keep the movable ground constant (Vmg = Vdc = 5 V)...

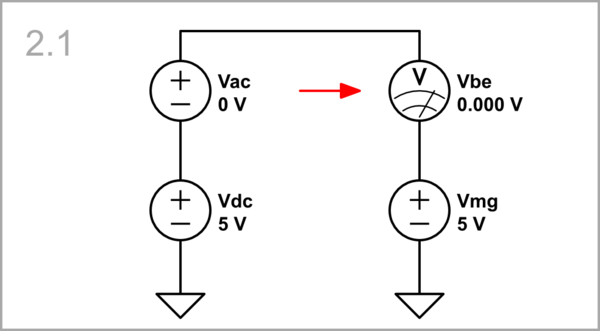
^{simulate this circuit}

Vac = 100 mV, Vmg = 5 V: ... and increase Vac...

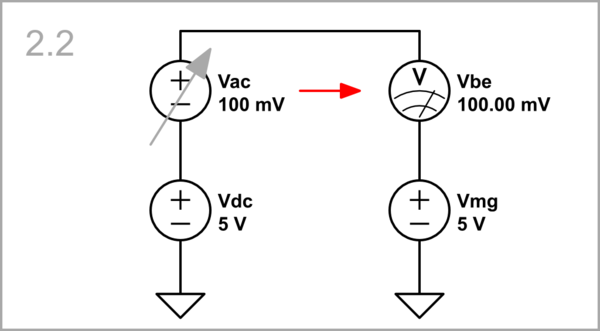
^{simulate this circuit}

Vac = -100 mV, Vmg = 5 V: ... or decrease Vac...

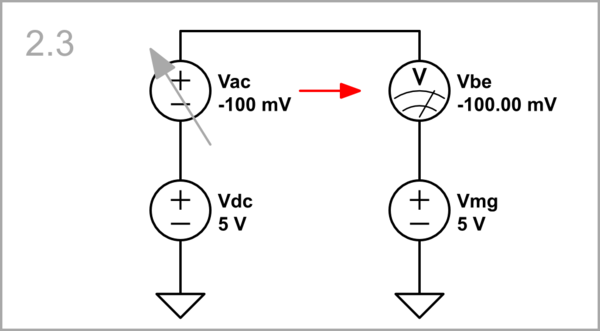
^{simulate this circuit}

... the result is the same - the Vac variations appear across Vbe. This mode corresponds to the differential mode of the differential amplifier.

Movable virtual ground

But if we move the ground in the same direction...

Vac = 100 mV, Vmg = 5.1 V: ... simultaneously increasing both Vac and Vmg...

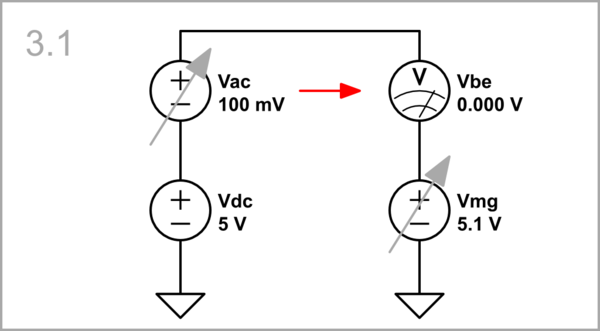
^{simulate this circuit}

Vac = -100 mV, Vmg = 4.9 V: ... or decreasing them...

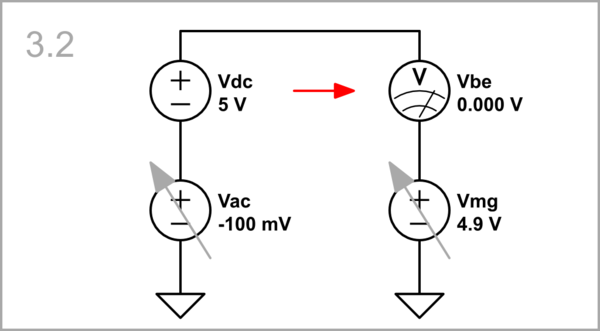
^{simulate this circuit}

... the result is the same - Vbe = 0 V. This mode corresponds to the common mode of the differential amplifier.

But what is the advantage of this way of coupling amplifier stages? To answer this question, let's see how the "movable ground" is implemented in differential amplifiers.

Movable ground implementation

This "long-tailed pair" arrangement consists of three "sources" in parallel - two (input) voltage sources V1 and V2, and a current source I. For our purposes, we can ignore the current source and focus only on the two voltage sources. So this differential configuration is doubled compared to the single-ended configuration above.

Movable ground: If the two input voltages change in the same way (common mode), the midpoint FG will also change this way, and will act as a "following movable ground".

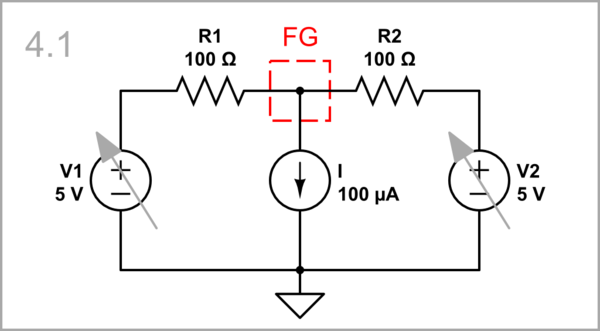
^{simulate this circuit}

Fixed ground: If the two input voltages change in the opposite directions (differential mode), the VG midpoint voltage will not change, and will act as a "virtual ground".

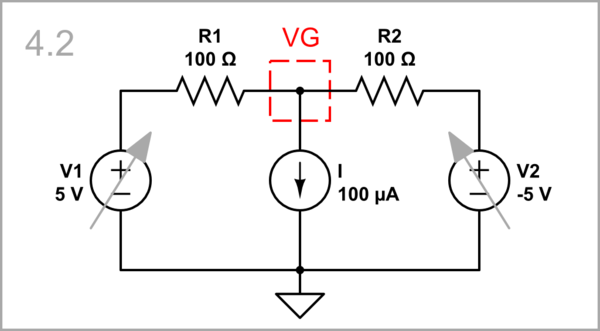
^{simulate this circuit}

So the mechanism of this differential coupling is as follows: When V1 = V2, the "movable virtual ground" moves freely to the Vdc level, and when V1 = -V2, it is fixed there. Its advantage is that the "movable ground" is automatically set equal to the input DC voltage and will move when it varies due to temperature for example.

Practical circuit

This concept can be seen in the practical circuit of the transistor differential amplifier below. The previous stage is simplified and split into two halves, and they are placed on either side of the next step.

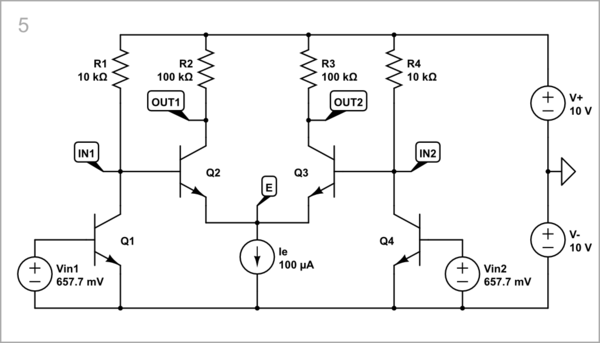
^{simulate this circuit}

You can conduct the conceptual experiments above by this practical circuit.

Conclusion

DC amplifiers are made by cascading differential amplifier stages because their input voltages are referenced to a movable internal ground which follows the input quiescent voltages.