Initial condition across capacitor - RLC

Question

The switch has been in the original position for a long time and is flipped at t=0 (apologies for my drawing skills)

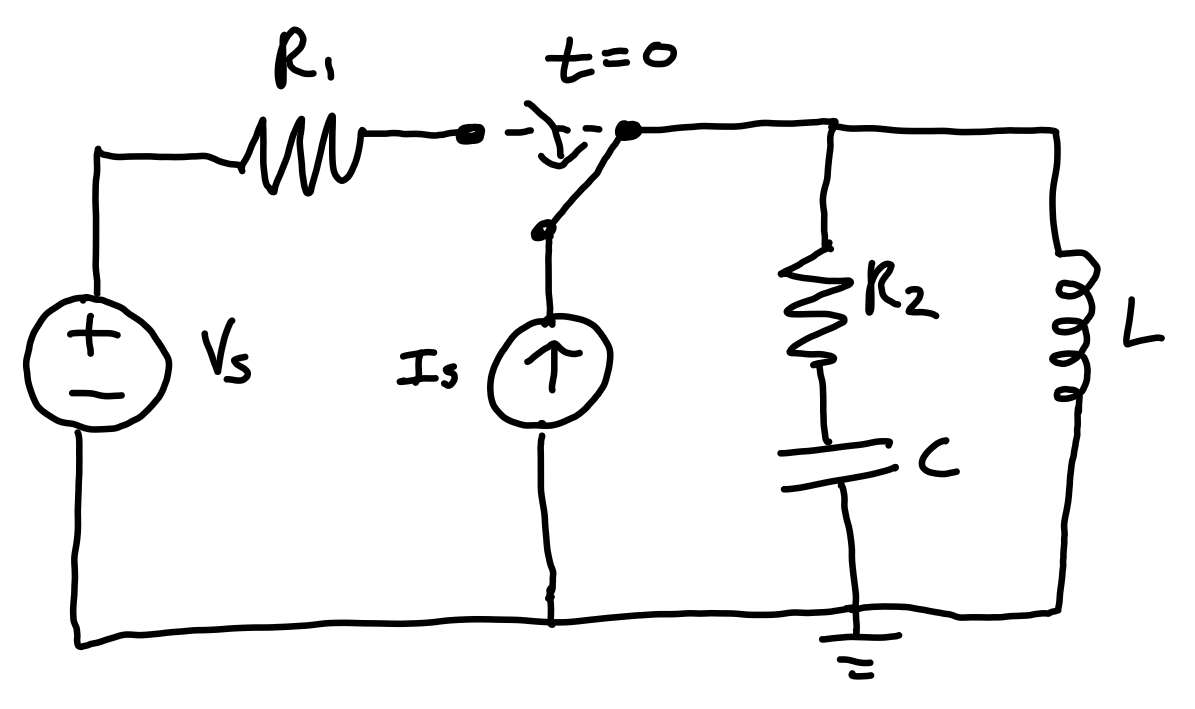
I am trying to find the initial condition across the capacitor. I am confused because the capacitor will resemble an open circuit and the inductor will resemble a short circuit, right? So there is no current flowing through the capacitor or R2. It was easy enough to get the initial condition for the inductor, i_L(0-), but I am confused on how to get the initial condition of the capacitor v_C(0-) without current.

I thought I could get the voltage across the inductor and then use that to do V_R2+V_C=V_L but then I got even more confused. How do I go about this?

Answer 1

In the very long time between \$t=-\infty\$ and \$t=0^-\$, the current in the inductor \$L\$ will stop changing. Because of \$V_L=L\cdot \frac{\text{d}}{\text{d}t}I_L\$, this means \$V_L=0\:\text{V}\$ and all of the voltage drop available from \$V_s\$ will appear across \$R_1\$. This also means, regardless of what happened much earlier, prior to \$t=0^-\$, there will be \$0\:\text{V}\$ across the series branch of \$R_2\$+\$C\$. If \$C\$ ever did have a voltage across it prior to \$t=0^-\$, it most certainly cannot have one at \$t=0^-\$. Any charge would have discharged via \$R_2\$ and \$L\$ by \$t=0^-\$.

At the moment of switching, the state of \$C\$ should be \$0\:\text{V}\$ and the state of \$L\$ should be \$I_L=\frac{V_s}{R_1}\$. At this point, \$t=0^+\$, KCL tells you that the current into the top node will be \$I_s\$, the current leaving the node via \$L\$ will be \$I_L=\frac{V_s}{R_1}\$ so any difference, \$I_s-\frac{V_s}{R_1}\$, is the downward-pointed current into \$R_2\$. From this, and the fact that \$V_C=0\:\text{V}\$, you can compute the initial node voltage at \$t=0^+\$. That initial node voltage will provide a rate of change in the current in \$L\$ to start altering \$I_L\$ and will also provide a current into \$C\$ that will start altering \$V_C\$.