I will briefly state my understanding of the following equation, and then pose three questions at the end.
Equation (1) (2) refers to the circuit in the first picture, Equation (3)(4)(5) refers to the circuit in the second picture.
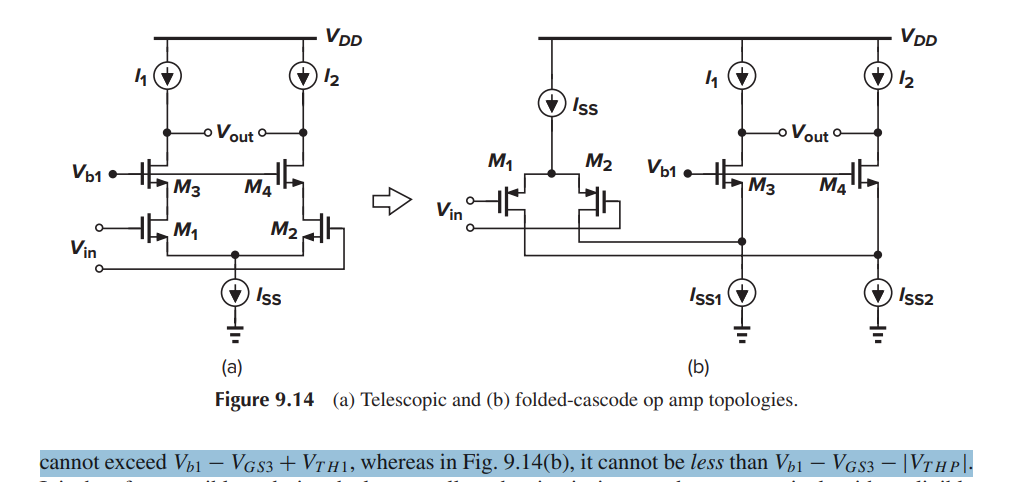
In Razavi’s book, chapter 9.2.4 Folded-cascode op amps, he stated that
In Fig. 9.14(a), the CM input level, cannot exceed
$$V_{b1} − V_{GS3} + V_{TH1} \tag{1}$$ whereas in Fig. 9.14(b), it cannot be less than
$$V_{b1} − V_{GS3} − |V_{THP}| \tag{2}$$ (where \$V_{THP}\$ stands for the threshold voltage of PMOS transistor)
I am wondering, how is these 2 equations come from?
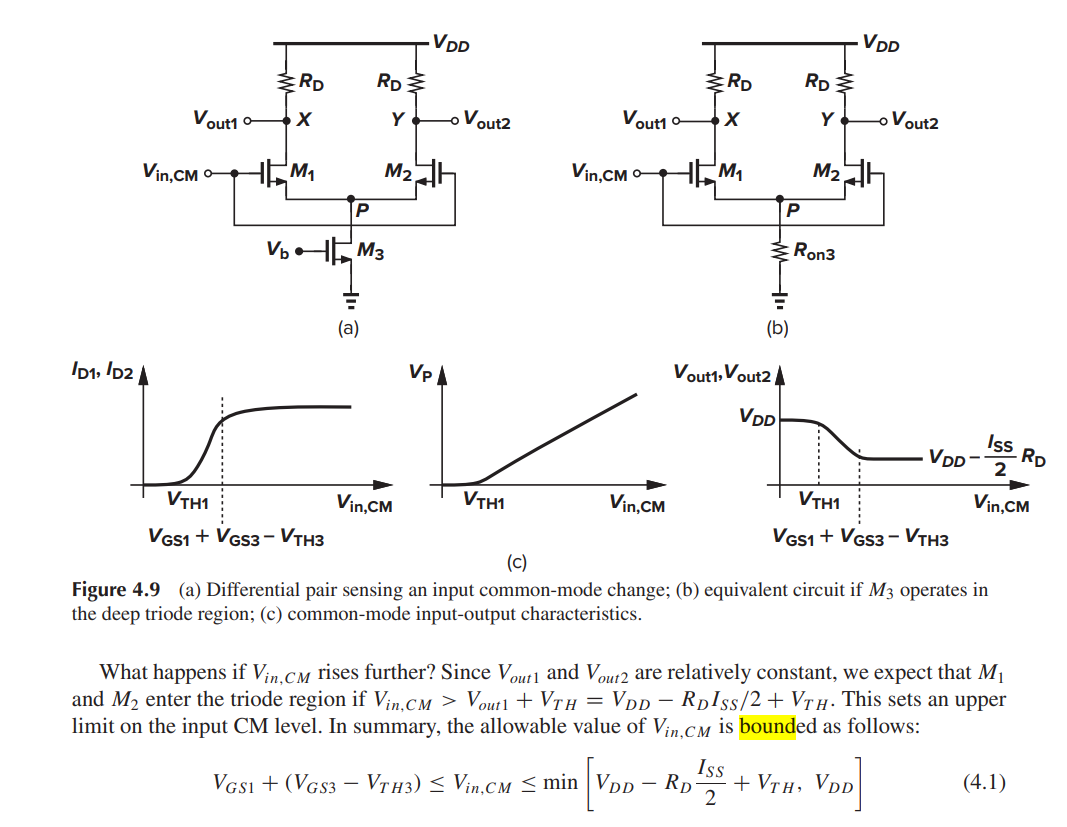
I have searched other parts of the book related to the range of CM input level, trying to find the origin of these two equations. In chapter 4.2, he provides the following formula, as shown in the attached picture.
I think that I understand the right half the inequality equation, that we need to make sure the
\$ V_{DS} \geq V_{OV} \$ so that those transistors not enter into triode region.
But I have confusion about the left half of the inequality equation, for the left half, he stated that
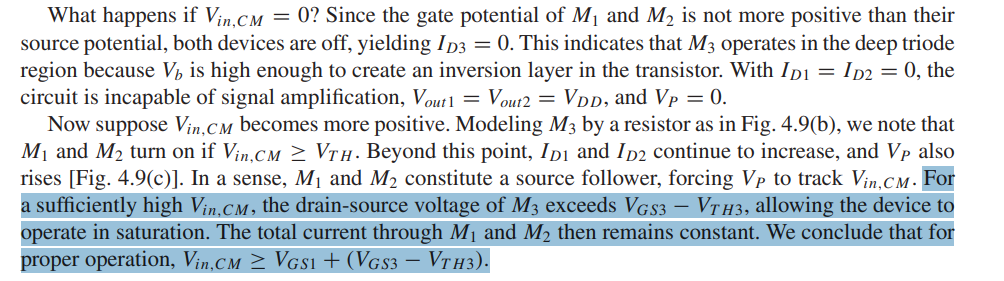
For a sufficiently high \$V_{in,CM}\$ , the drain-source voltage of \$M_3\$ exceeds \$V_{GS3} − V_{TH3}\$, allowing the device to operate in saturation. The total current through \$M_1\$ and \$M_2\$ then remains constant. We conclude that for proper operation, \$V_{in,CM} ≥ V_{GS1} + (V_{GS3} − V_{T H3})\$.
If I understand his words, correctly, his inequality equation.
$$V_{in,CM} - V_{GS1} ≥ + (V_{GS3} − V_{T H3}) \tag{3} $$
Comes from that
$$V_{DS3} ≥ V_{OV3} \tag{4}$$
Then this suggests that
$$V_{in,CM}-V_{GS1}=V_{DS3} \tag{5} $$
I am curious about 3 questions
1: How is the boundary of CM input level of folded cascode amplifier calculated, that is to say, how is equation (1) and equation (2) derived from?
2: Is there any relation between the inequality of Fig 9.14 and Fig 4.9?
3: How is the Drain source voltage of transistor 3 in Fig 4.9 equal to \$ V_{in,CM}-V_{GS1} \$, that is, how is equation 5 derived from?