The following is a question from 8.18 in Design of Analog CMOS Integrated Circuit, page 342.
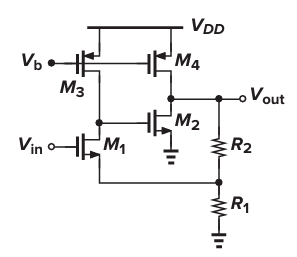
Consider the circuit this following Fig, \$(\frac{W} {L})_{1-4} =\frac{50} {0.5} \$, \$|{I_D}_{1-4}| = 0.5 mA\$, \$R_2 = 3 k\Omega\$.
Calculate the closed-loop gain and output impedance.
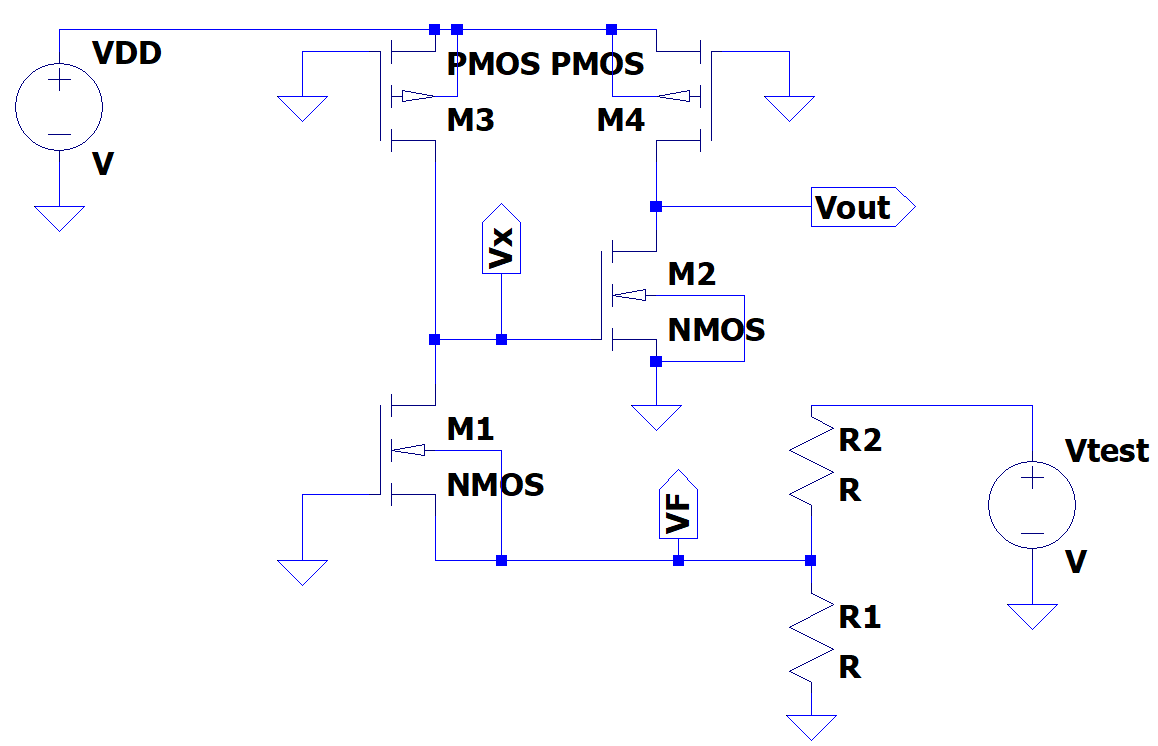
Break the loop and add test voltage.
$$\frac{V_x -V_F} {r_{o1}} - V_F g_{m1} = -\frac{V_x} {r_{o3}}$$
$$\Rightarrow \frac{V_x} {V_F} = (r_{o1} \parallel r_{o3}) (r_{o1} \parallel \frac{1} {g_{m1}})$$
$$- \frac{V_{out}} {r_{o4}} = V_x g_{m2} + \frac{V_{out}} {r_{o2}}$$
$$\Rightarrow \frac{V_{out}} {V_x} = - g_{m2} (r_{o2} \parallel r_{o4})$$
$$\Rightarrow \frac{V_{out}} {V_F} = \frac{V_{out}} {V_x} \frac{V_x} {V_F} = - g_{m2} (r_{o1} \parallel r_{o3}) (r_{o1} \parallel \frac{1} {g_{m1}}) (r_{o2} \parallel r_{o4})$$
$$V_F = \frac{R_1} {R_1 + R_2} V_{test}$$
$$A \beta = \frac{V_{out}} {V_{test}} = - g_{m2} (r_{o1} \parallel r_{o3}) (r_{o1} \parallel \frac{1} {g_{m1}}) (r_{o2} \parallel r_{o4}) \frac{R_1} {R_1 + R_2} $$
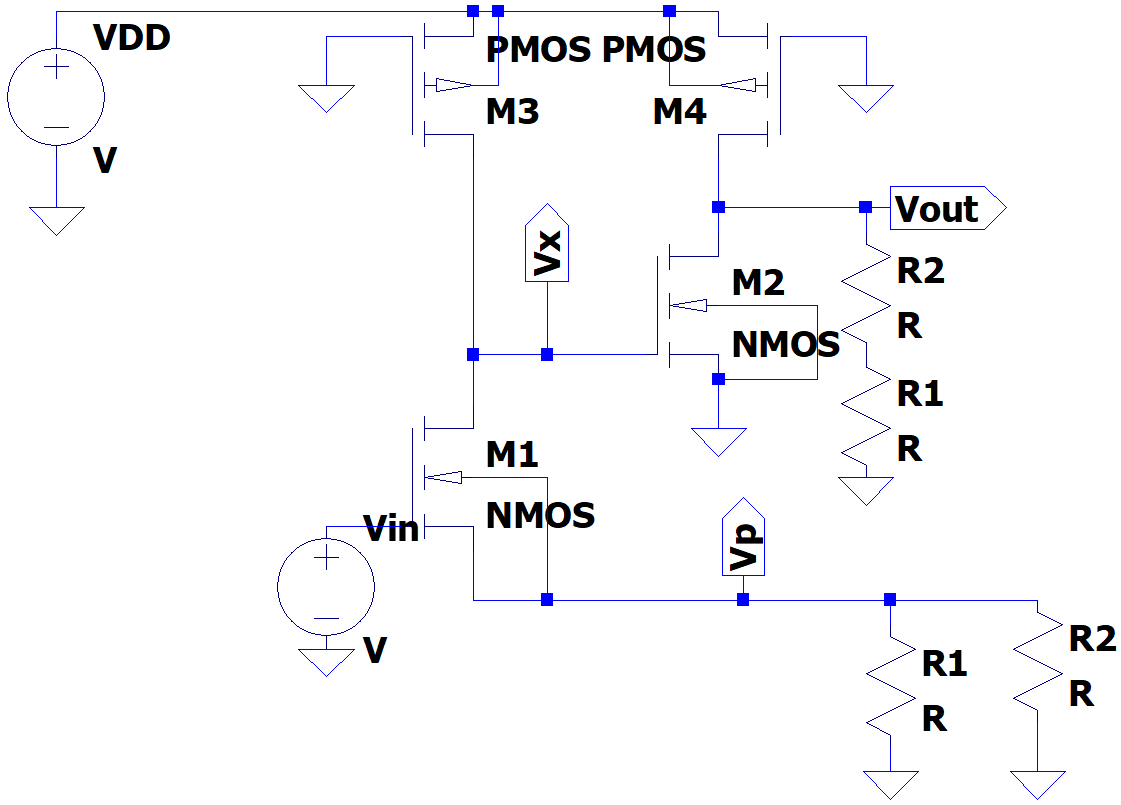
To calculate open loop gain, we can have this circuit.
Between M1 and M3, we have $$-\frac{V_x} {r_{o3}} = \frac{V_p} {R_1 \parallel R_2}$$ $$\frac{V_x - V_p} {r_{o1}} + (V_{in} - V_p) g_{m1} = - \frac{V_x} {r_{o3}}$$
$$\Rightarrow \frac{V_x} {V_{in}} = \frac{g_{m1}} {1 / (r_{o1} \parallel r_{o3}) + \frac{R_1 \parallel R_2} {r_{o1} \parallel \frac{1} {g_{m1}}} }$$
Between M2 and M4, we have
$$- (\frac{V_{out}} {r_{o4}} + \frac{V_{out}} {R_1 + R_2}) = V_x g_{m2} + \frac{V_{out}} {r_{o2}}$$
$$\Rightarrow \frac{V_{out}} {V_x} = -g_{m2} \bigr(r_{o2} \parallel r_{o4} \parallel (R_1 + R_2) \bigr)$$
$$A_{v,open} = \frac{V_{out}} {V_{in}} = \frac{V_{out}} {V_x} \frac{V_x} {V_{in}} = -g_{m2} \frac{g_{m1}} {1 / (r_{o1} \parallel r_{o3}) + \frac{R_1 \parallel R_2} {r_{o1} \parallel \frac{1} {g_{m1}}} } \bigr(r_{o2} \parallel r_{o4} \parallel (R_1 + R_2) \bigr)$$
$$A_{v,close} = \frac{A_{v,open}} {1 + A \beta}$$
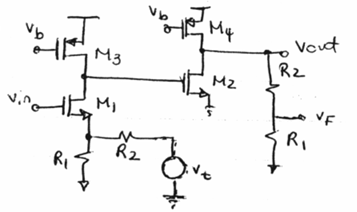
The following is what I found from internet. Is this correct?
$$A \beta = \frac{V_F} {V_t} = \frac{1} {R_2} (R_1 \parallel R_2) \frac{r_{o3}} {(R_1 \parallel R_2) + \frac{1} {g_{m1}}} g_{m2} (r_{o4} \parallel (R_1 + R_2) \parallel r_{o2}) \frac{R_1} {R_1 + R_2}$$
$$A_{v,close} = \frac{r_{o3}} {(R_1 \parallel R_2) + \frac{1} {g_{m1}}} g_{m2} \Bigr(r_{o4} \parallel (R_1 + R_2) \parallel r_{o2} \Bigr)$$