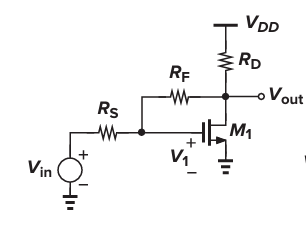
The following is from the book Design of Analog CMOS Integrated Circuit, page 319.
Let's break the loop at the gate of M1.
$$V_{out} = - V_T g_m \biggr[ (R_S + R_F) \parallel R_D\biggr] $$
$$V_F = V_{out} \frac{R_S} {R_F + R_S} $$
$$\Rightarrow \frac{V_F} {V_T} = - \frac{g_m R_S R_D} {R_D + R_S + R_F}$$
$$\Rightarrow L(s) = \frac{V_F} {V_T} = -\frac{g_m R_S R_D} {R_D + R_S + R_F}$$
\$\beta\$ negative since it's negative feedback.
$$V_G = \frac{V_{in} R_F + V_{out} R_S} {R_F + R_S} $$
$$\Rightarrow \beta = -\frac{R_S} {R_S + R_F}$$
$$A_{v, close} = \frac{L} {1 + L} \frac{1} {\beta} = \frac{g_m R_D (R_F + R_S)} {R_D + R_S + R_F - g_m R_D R_S} $$
However, the exact close loop gain is
$$A_{v,close} = \frac{(1 - g_m R_F) R_D} {R_D + R_F + R_S + R_D R_S g_m}$$
You can see how I derive this exact close loop gain from my previous post previous post.
the closed-loop frequency response of a feedback circuit can be expressed without reference to A(s)
$$A_{CL} = \frac{1} {\beta} \frac{L(s)} {1 + L(s)} $$
In conclusion, these two gains are not the same. Is there anything wrong here?