1
\$\begingroup\$

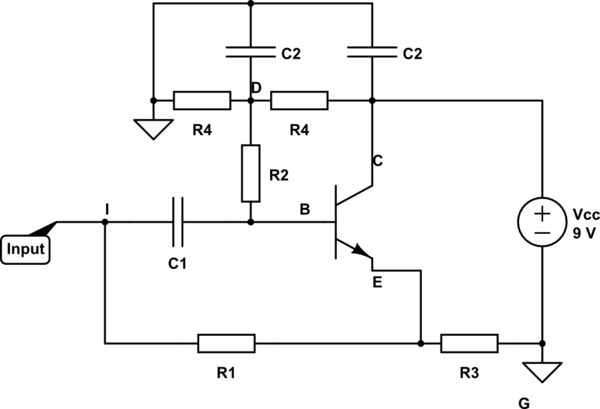
According to this link, the following schematic is equivalent to an inductance R1*R2*C placed between input and ground. Under which conditions is this true? As a guideline, we assume Vcc=9V. See this post for the context of the question.

schematic

simulate this circuit – Schematic created using CircuitLab

Application with R1=390 Ohm, R2=22 kOhm, R3=2.2 kOhm, R4=70 kOhm, C1=100 nF, C2=200 uF.

\$\endgroup\$
5
  • \$\begingroup\$ You're forgetting the explicit voltage divider for Vcc/2. This is important, because this is not a low impedance Vcc/2 source. \$\endgroup\$
    – user36129
    Commented Oct 29, 2013 at 8:11
  • \$\begingroup\$ When you read "VCC/2" on a schematic it is reasonable to assume a low impedance voltage source, such as a well decoupled supply, unless otherwise stated. So, that would be one of the conditions... \$\endgroup\$
    – user16324
    Commented Oct 29, 2013 at 9:55
  • \$\begingroup\$ Well on the PCB I see that R4 is 70k and R2 is 22k so that might not be exactly the case. No idea if that plays a role. \$\endgroup\$
    – yannick
    Commented Oct 29, 2013 at 10:06
  • \$\begingroup\$ At point D there should be a decoupling capacitor to ground that is very much bigger than C. Without it theformula for inductance will include R4. \$\endgroup\$
    – Andy aka
    Commented Oct 29, 2013 at 10:18
  • \$\begingroup\$ that was indeed the case. Could you explain their role? \$\endgroup\$
    – yannick
    Commented Oct 29, 2013 at 10:27

2 Answers 2

Reset to default
2
\$\begingroup\$

There are better gyrator circuits so let me give you the downsides of this circuit first.

The idea behind this gyrator circuit is that at the emitter there is a voltage that connects back to the input via R1. If the emitter voltage is phase shifted to the input, it will take a current (via R1) from the input that appears to be reactive i.e. it looks like an inductor's current.

However the input is also feeding the network (C1 etc.) which does the phase shifting and so this "capacitive circuit" is in parallel with the "intentional" inductive current via R1. This makes it a band-pass filter but, it can look like an inductor across a range of frequencies.

Better gyrators use an op-amp or another transistor to buffer C1, Anyway, the analysis: -

At point B (base) the AC voltage relative there to the input voltage is: -

\$\dfrac{R_2}{R_2+\dfrac{1}{sC_1}}\$ and this voltage is also at the emitter (the emitter voltage is fractionally less in AC terms but this can be largely ignored). The emitter also acts as a reasonably good ideal voltage source so we don't have to worry about its output impedance of a few ohms.

The current into R1 is the voltage across it divided by R1 (I = V/R): -

Current = \$\dfrac{V_{IN}}{R_1}(1-\dfrac{sC_1 R_2}{sC_1 R_2+1})\$

The impedance, Z into R1 is \$V_{IN}\$ divided by current: -

Z = \$\dfrac{R_1}{1-\dfrac{sC_1 R_2}{sC_1 R_2+1}}\$ = \$\dfrac{R_1+sC_1 R_1 R_2}{1+sC_1 R_2-sC_1 R_2} = R_1+sC_1 R_1 R_2\$

In other words the impedance looking into R1 is an inductance of C1*R1*R2 in series with a resistor of R1 ohms. Remember there is current through the capacitor but this can be ignored if R2 is a lot bigger than R1 and the gain of the transistor is high.

\$\endgroup\$
10
  • \$\begingroup\$ Fantastic! I extended your conclusion a bit. Could you also explain the ground capacitors? Also, I'm guessing \$R_3\$ is only here to set the quiescent point. \$\endgroup\$
    – yannick
    Commented Oct 30, 2013 at 12:16
  • \$\begingroup\$ @yannick Your extensions are incorrect. Current through the capacitor is not what you say and Zin does not become what you suggest. I'm sorry but I'm rejecting your additions. \$\endgroup\$
    – Andy aka
    Commented Oct 30, 2013 at 12:30
  • \$\begingroup\$ @Anindo can you please recheck the additions made by yannick that you approved. They are incorrect and they are largely irrelevant given that I've already described this gyrator as being a poor circuit in my opening paragraph. \$\endgroup\$
    – Andy aka
    Commented Oct 30, 2013 at 12:38
  • \$\begingroup\$ @Davetweed can you please recheck the additions made by yannick that you approved. They are incorrect and they are largely irrelevant given that I've already described this gyrator as being a poor circuit in my opening paragraph. \$\endgroup\$
    – Andy aka
    Commented Oct 30, 2013 at 12:38
  • \$\begingroup\$ Given that your first equation is a voltage divider and therefore assumes that the current in the base is negligible, I think the current in the capacitor is correct. Ohm's law through series-connected capacitor and R2, with Vin applied to it. \$\endgroup\$
    – yannick
    Commented Oct 30, 2013 at 14:31
0
\$\begingroup\$

Redoing the analysis, I find a somewhat different result for the real part of the impedance

\$Z=R_1+\frac{R_2}{\beta+1} + j\omega C_1 R_1 R_2\$

\$\endgroup\$
1
  • \$\begingroup\$ Your impedance Z' - it is made of two parts; the one I derived (R1 + sC1.R1.R2) and (1/sC1 + R2). Called them Z1 and Z2 - the parallel combination is \$\frac{Z1\times Z2}{Z1+Z2}\$ \$\endgroup\$
    – Andy aka
    Commented Oct 31, 2013 at 10:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.