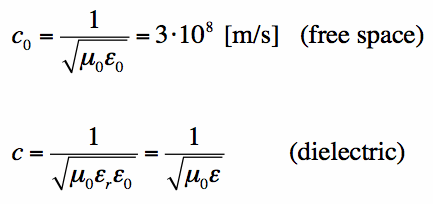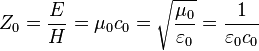How much is the propagation velocity affected by the characteristic impedance?

Question

Someone posted the (very educational!) video Similiarities of Wave Behavior from 1959 in another answer, and after watching the part about impedance mismatches, it strikes me that the propagation velocity in the two sections with a different impedance is remarkably different. It's hard to estimate from the video, but it looks like the wave moves at least twice as fast through the narrow section.

This made me wonder if the difference is really that big even in electrical circuits, and particularly on a circuit board.

I've mostly used the rule-of-thumb half the speed of light when estimating the signal delay on circuit boards using plain old FR4, but I have never designed anything that required me to know or care about the characteristic impedances of the trace. I do know that sometimes it matters, and that you may have to make sure that the board house can manufacture controlled impedance boards.

I have seen characteristic impedances of cables from 50Ω to 300Ω, but I'm sure there are ranges outside of this. Presumably, you can match these on a PCB, and that should make the propagation velocity different as well. I have also played with trace impedance calculators and they often give impossibly large trace widths for the common impedances when you use the standard 1.6 mm, two layer board. This makes me think that the impedance of a trace will vary by a lot even on the same PCB unless you actively manage it.

There are numerous questions and answers that explains how to match the characteristic impedance of a PCB trace to a given requirement, but is there an equation that relates this to the propagation velocity? If so, what is it? Can it be calculated just using the impedance?

Answer 1

The velocity that electricity propagates at is related to the dielectric in the cable. In free-space, an EM wave travels at the speed of light but there is only the permittivity of free space (\$\epsilon_0\$)to hinder it: -

So, as the permittivity (\$\epsilon_r\$) rises, velocity decreases. Magnetic permeability is the other factor but this barely changes between a cable and free-space.

The characterisitic impedance of free space is also related to those terms: -

Basically, if you compared both equations, as characteristic impedance drops (due to permittivity increasing), velocity of propagation also drops.

The product of speed and impedance is \$\dfrac{1}{\epsilon}\$

And the ratio of impedance to speed is \$\mu_0\$.

I've used \$\mu_0\$ above (rather than \$\mu\$) because there is no significant increase in magnetic permeability due to a cable's construction.