Power absorbed by a resistor in a crossed circuit

Question

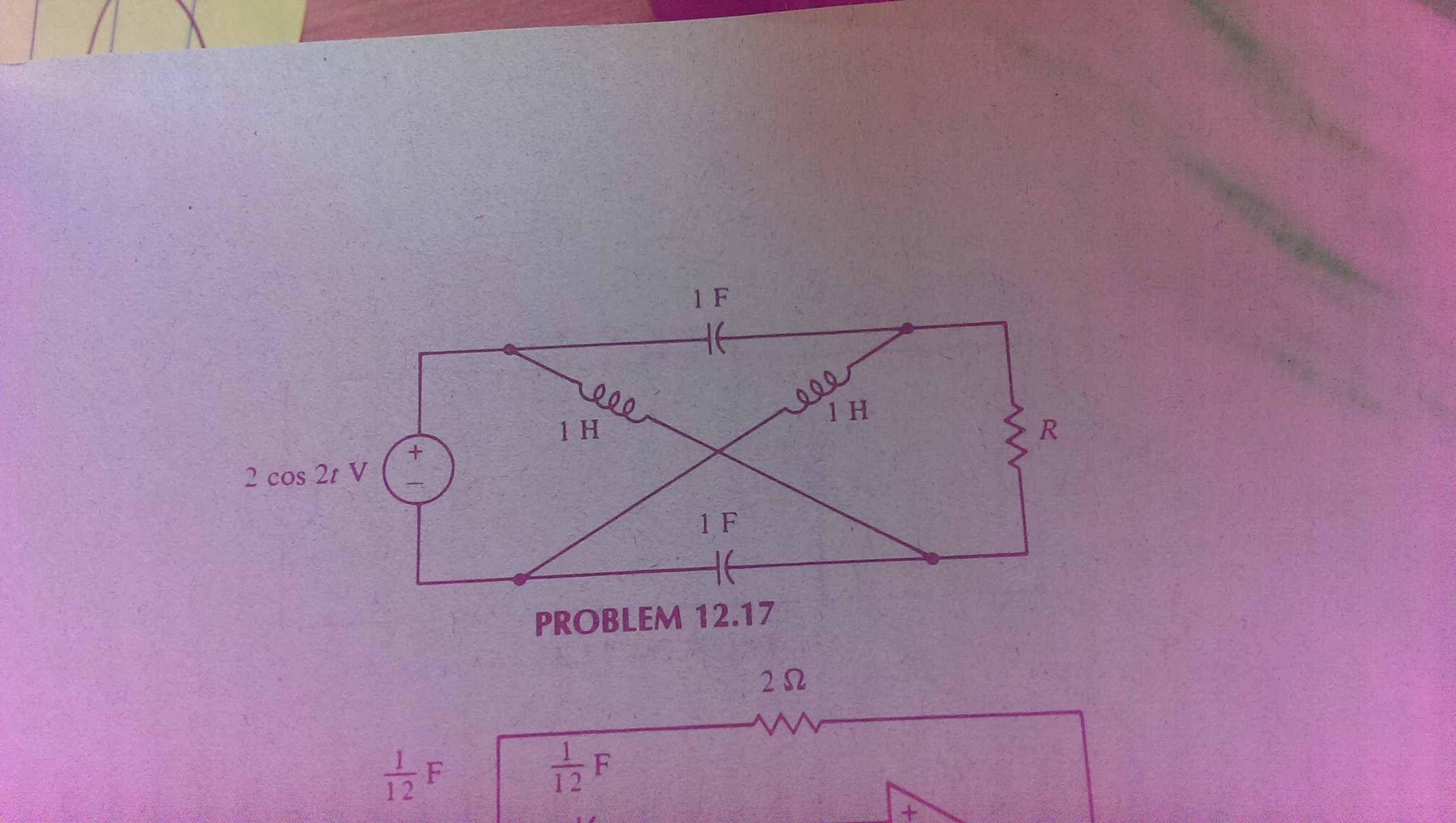
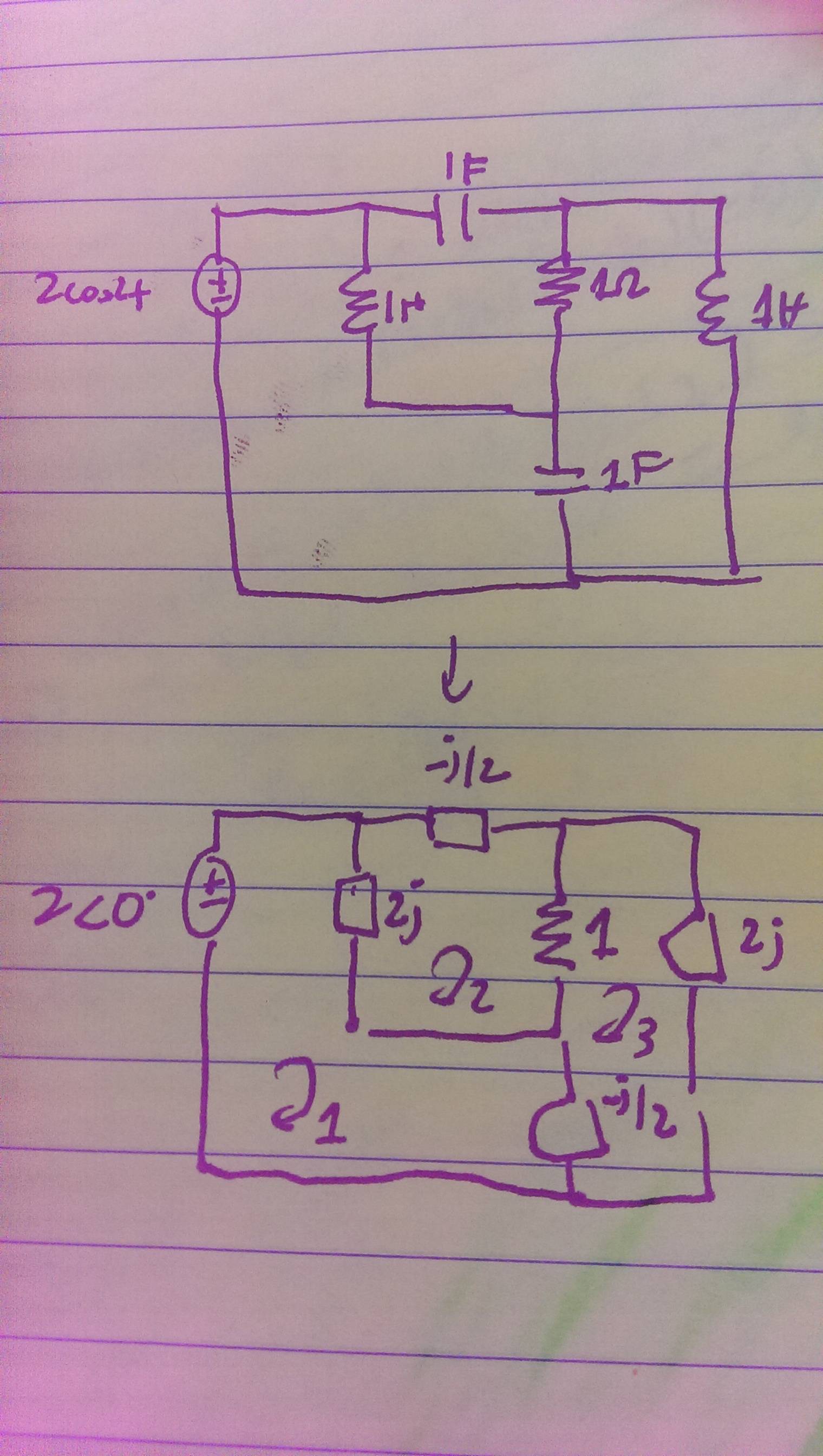
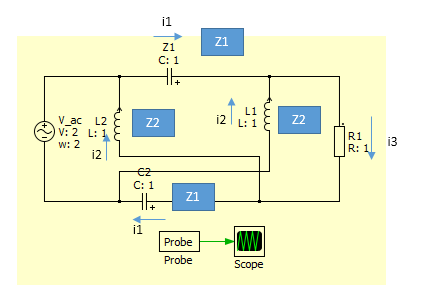
The given problem above is asking for the power absorbed by the resistor R given R = 1 Ohm. According to my professor, the two inductors do not cross so I redrew the circuit into the one shown in the image below. I then redrew the circuit into its phasor equivalent also shown in the same photo.

I then did the following steps:

KVL at I1:

-2 + I1(2j-j/2) - I2(2j) – I3(-j/2) = 0

(3/2)j I1 - 2j I2 + j/2 I3 = 2  (1)

KVL at I2:

I2(2j+1+-j/2) – I1(2j) – I3(1) = 0

-2jI1 + (1+(3/2)j)I2 – I3 = 0     (2)


KVL at I3:

I3(1+2j – j/2) – I1(-j/2) – I2(1) = 0

j/2 I1 – I2 + (1+(3/2)j I3) = 0  (3)


Using Cramer’s Rule to get I2 and I3:


Divisor:

[((3/2)j)(1+(3/2)j)(1+(3/2)j) + (-2j)(1)(j/2) + (j/2)(-2j)(-1)] - 

[(j/2)(1+(3/2)j)(j/2) + (-1)(-1)((3/2)j) + (1+(3/2)j)(-2j)(-2j)]

= -1/4 + 3j

Dividend for I2:

[0 + 2(-1)(j/2)+0] – [0+0+(1+(3/2)j)(-2j)(2)] = -6+3j

Dividend for I3:

[0+0+(2)(-2j)(-1)] – [(j/2)(1+(3/2)j)(2)] = 3/2 + 3j


I2 = (-6+3j )/ (-1/4 + 3j) = 168/145 +(276/145)j

I3 = (3/2 +3j) / (-1/4 + 3j) = 138/145 – (84/145) j


I2 + I3 =   [168/145 +(276/145)j] + [138/145 – (84/145) j] = 306/145 + (192/145)j

Using the Average power equation:

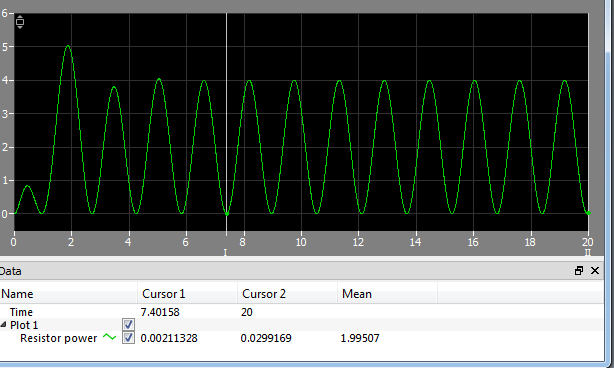
P(1-Ohm) =(1/2)|306/145 + (192/145)j|^2 Re{1} = 3.1 W

The answer in the book however is 2 W. Where did I go wrong? Is my redrawn circuit correct? Thanks.

Thoughts on using the Thevenin equivalent circuit to solve this problem?

Answer 1

The inductors are crossed.

The circuit is also symmetrical - such that the voltage across the top capacitor is opposite to the voltage of the bottom capacitor. Similar rule applies to inductor current. Let's exploit the symmetry.

Result: the rms dissipated power in the resistor is indeed 2 W.

KCL:

\$ i_3=i_1+i_2 \$

KVL1: (around the voltage source, the two capacitors, and the resistor)

\$ V=2Z_1i_1+i_3R = 2Z_1i_1+Ri_1+Ri_2 \$

KVL2: (around the voltage source, L2, and C2)

\$ V=-Z_2i_2+i_1Z_1 \$

Solution:

\$ R=1\Omega, V = 2V, Z1 = 1/(\omega Cj) = -0.5j, Z2 = \omega Lj = 2j\$

\$ i_1= (V+VR/Z_2)/(2Z_1+R+RZ_1/Z_2)\$

\$ i_2= (i_1Z_1-V)/Z_2\$

\$ i_1= 1.6+0.8j\$

\$ i_2= -0.4+0.8j\$

\$ i_3= 1.2+1.6j\$

Absolute value of the resistor current phasor is 2 A. The rms is then \$ \sqrt 2A \$ and the power dissipation \$ P = Ri_3^2=1\Omega*(\sqrt2A)^2=2W\$

Notice that due to the capacitor/resistor value symmetry the dissipated power is independent of the excitation frequency.

Schematic (Z1 are the capacitors, Z2 are the inductors):

Results:

Thoughts on using the Thevenin equivalent circuit to solve this problem?

Not sure what Thevenin will give you here- the circuit can be solved using two KVLs! Also, the impedances are frequency dependent and Thevenin/Norton theorems are typically used for DC voltage/current sources and resistive networks.

Btw. thank you for this interesting question! I had fun solving it :).