The triangle waveform and the square wave amplitude ideally (as in using an ideal op-amp/comparator) will not vary with frequency- it would be constant for a given supply voltage.
That is because the amplitude of both is determined by the output saturation voltage of the right-hand op-amp (used as a comparator), which (ideally) depends on the supply voltage only (once it gets there).
To put it succinctly, the left hand op-amp is responsible for the frequency,and the right-hand op-amp for the amplitude.
The problem with an aggregate measurement of a complex waveform is that you may be missing something. In this case, I believe you are missing the fact that the square wave is not really square (and as a result, the triangle wave is not really triangular).
The op-amp (used as a comparator with hysteresis proportional to the supply voltage as determined by the R1/R2 ratio) has a finite slew rate and thus the edges of the square wave are really ramps. Thus the square wave spends less time at the limits. Similarly the triangle wave is created by integrating the square wave, so the slopes of the triangle wave will not be straight lines, but will curve upward or downward on rising/falling slopes.
A secondary effect is that some op-amps take a long time to recover from saturation so the output of the comparator op-amp will be delayed by a (more-or-less) fixed amount on each edge. However that would tend to make the output triangle waveform amplitude increase with frequency and would not affect the square wave, so I don't think that's a factor here.
Edit: Andy is also correct that loading of the op-amp/comparator output may be a factor. The limiting output voltage of the op-amp will change with loading.
You can avoid this problem by using a proper comparator instead of the right-hand op-amp with hard output limiting using a pair of zeners, say, so the square wave amplitude is controlled. It will transition in nanoseconds (maybe a couple hundred for a really slow comparator) and your variation with frequency will (mostly) go away until you run into the slew rate limit of the left-hand op-amp on the triangle wave slopes.
TL;DR
It's the comparator op-amp slew rate limitation and/or loading making crummy square waves of non-fixed shape and amplitude.
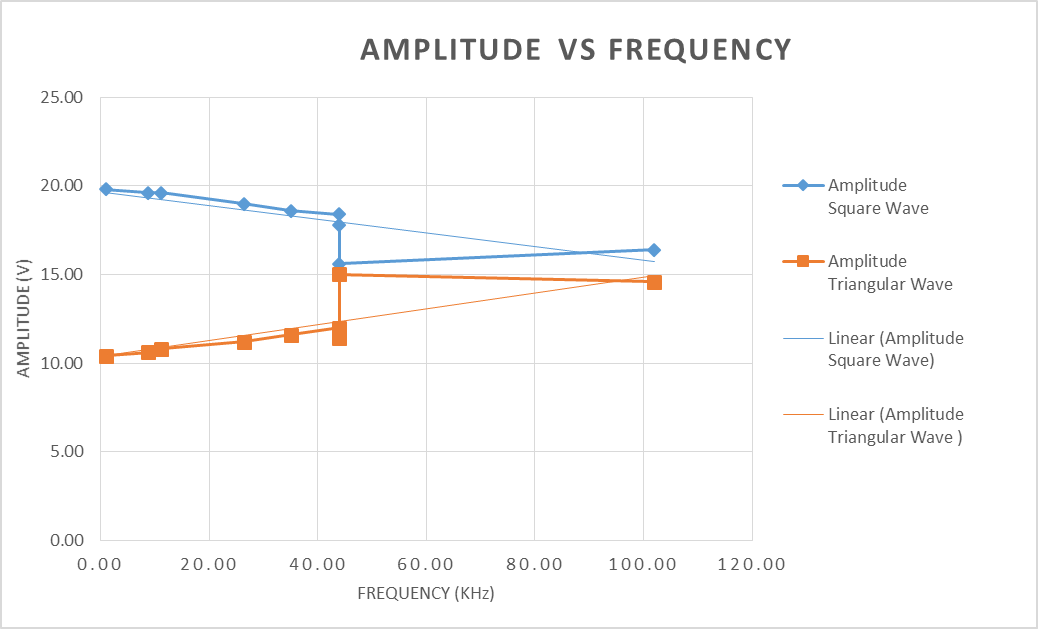 Note: There are two points at 45Khz. The lowest amplitude point of square wave are taken when I selected a greater value of capacitor and smaller value of resistor to form the same combination for 45KHz.
Note: There are two points at 45Khz. The lowest amplitude point of square wave are taken when I selected a greater value of capacitor and smaller value of resistor to form the same combination for 45KHz.