1
\$\begingroup\$

In my book there is a circuit like the one shown bellow. The author (G. Randy Slone) explains the operation and performance of it but there is still one thing that left me wondering.

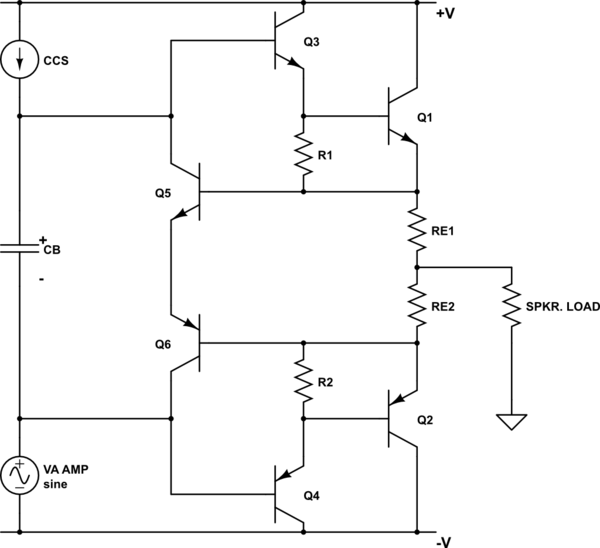
schematic

simulate this circuit – Schematic created using CircuitLab

  • Since the signal from Voltage Amplification (VA) stage is not applied directly to both of bases of Q3 and Q4 but only for Q4, how can whole sine wave be normally buffered through the Output Power Stage (OPS)? Or does the signal from the VA goes to both of the bases via capacitor? Since author wrote: "...CB filters small signal variation applied to bias network...", does that mean that VA signal goes to the base of Q3 via CB?
\$\endgroup\$

1 Answer 1

Reset to default
2
\$\begingroup\$

The (AC) signal from VA does directly end up at the base of both Q3 and Q4 through the capacitor CB.

The DC voltage at the base of Q3 and the base of Q4 cannot be the same so we cannot short Q3 and Q4's bases. If we did that then the whole DC biasing of the circuit would be influenced and it would not work anymore. So we cannot short the bases for DC. But we can still short them for AC (the signal), that's what capacitor CB does.

The capacitor CB and the rest of the circuit act like a DC voltage source (like a battery) so the signal at VA also appears at the base of Q3 but with an additional DC-voltage added.

How much is that DC voltage?

Follow this path: Base-Emitter of Q3 + BE of Q1 + V(RE1) + V(RE2) + EB Q2 + EB Q4 so about 4 x a Vbe + the voltage across RE1 and RE2 which depends on their value and the DC biasing current through them.

Sure but how much voltage will there be across RE1 and RE2?

That's where Q5 and Q6 come in, if the voltage across RE1 and RE2 starts to become more than 2 x 0.6 = 1.2 V then Q5 and Q6 start to conduct, they then "steal" some of the base current of Q3 and Q4 making Q3 and Q4 conduct less which makes Q1 and Q2 conduct less which makes less current flow through RE1 and RE2.

\$\endgroup\$
2
  • \$\begingroup\$ That is one good explanation! Although I was only curious if that "small signal variation" means AC. Nice! \$\endgroup\$
    – lucenzo97
    Commented Dec 22, 2017 at 15:57
  • 1
    \$\begingroup\$ Thanks, yes I mean small signal variation when writing AC. As you know AC usually means something different but in this context AC does mean "small signal variations". AC is so much shorter and more convenient to write :-) \$\endgroup\$
    – Bimpelrekkie
    Commented Dec 22, 2017 at 16:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.