I cannot interpret couple of things from the datasheet of this optoisolator.
1-) Is there and hint in the datasheet regarding the maximum CM voltage interference it can handle at its inputs?
2-) The input capacitance is given as 18 pF. Is this the capacitance between the input and the output of the optoisolator? Is it possible to say what frequency of CM interference would this optoisolator pass through regarding this capacitance?
The capacitance stated (18 pF) is the typical capacitance of the diode when it has zero bias (VR = 0 volts). That is the capacitance between the diode terminals.
Is this the capacitance between the input and the output of the optoisolator?
No, that capacitance will be around 1 pF or less. If you look at the equivalent data sheet for the Fairchild device it states a typical capacitance of 0.4 pF and a max value of 0.6 pF. The Fairchild DS also states that the isolation resistance is a minimum of \$10^{11}\$ ohms.
Is there and hint in the datasheet regarding the maximum CM voltage interference it can handle at its inputs?
Given that the diode is "floating" you can make a reasonable assumption that it may be imbalanced to earth with 1 pF on one lead and 0 pF on the other - this I would estimate to be a worst case scenario. External diode drive circuits (even the most simplest) would dominate this capacitance by a factor of ten or so. Basically nobody will quote such a value for just the opto/diode because of this. In other words, the external circuitry attached to the diode will swamp the diode's own capacitance and be the limiting factor that defines the common-mode capabilities.
Is it possible to say what frequency of CM interference would this optoisolator pass through regarding this capacitance?
It has a input/output capacitance of less than 1 pF and it is highly likely that any circuit board it is attached to will increase this by a factor of 2 to 10. It's a capacitor and all frequencies will pass through a capacitor but higher frequencies are of course going to produce more current.
If you drive the optocoupler STRONGLY, the receiver diode will be strongly conducting, making the receiver more immune to charge coming thru the 0.6pF between TX and RX.
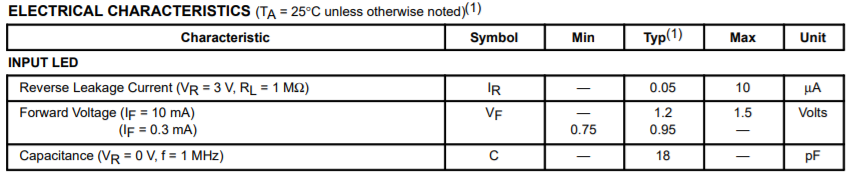
Assuming 1mA thru the RX diode, assume that 1mA produces a 26 ohm resistance at the diode bias point.
You certainly should expect to inject up to 50% of that 1mA into the Rx diode, without upsetting the data movement. I'd dial that back to 10%, for safety.
What does 10% of 1mA mean, thru a coupling cap of 0.6pF?
Given 1pF and 1 volt per nanosecond will produce 1mA, we know 0.6pF and 1.6 volts per nanosecond will also produce 1mA.
Since we cannot control the 0.6PF, your only adjustable knob is the slewrate. To reach 10%, you must have a SLOWER edge of interference between TX and RX.
For safety, you need your interference to be slower than 0.16 volts per nanosecond.
OR you need filtering across the RX side of the optocoupler.
