What is the exact meaning of 0.7V voltage drop in a forward-biased diode?

Question

this question is partially (but not totally) discussed here:

Why do diodes have a voltage drop?

Does the power drop on a diode become entirely heat?

The conclusion is that, in a forward-biased diode with steady DC current:

1. the voltage drop represents the necessary energy (per unit charge) to push charge carries (electrons and holes) from one semiconductor to the other.

2. the power drop on a forward-biased diode is entirely heat.

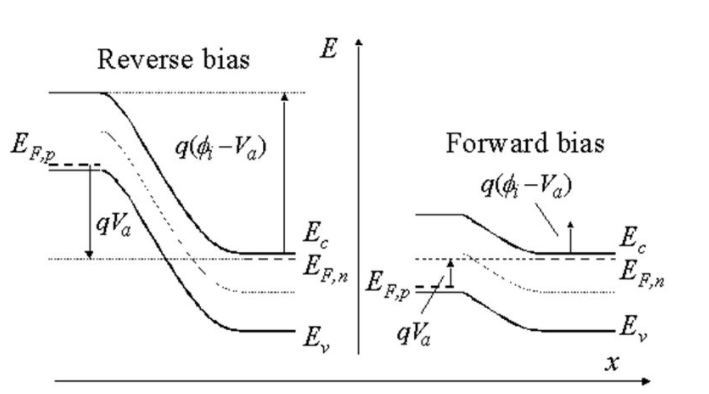
Let's focus more in detail on both statements. Let's consider the following band diagram in case of forward biasing:

I'd say that:

• when the diode is forward biased, holes are pushed from P to N and electrons from N to P, correct?

• both electrons and holes' levels change along the junction, since they correspond respectively to the conduction and valence bands. Their jump is the same since both levels are represented by parallel curves. Is the voltage drop of the diode equal to this jump, to twice this jump or other?

• what do the charge carriers injected in each semiconductor do after they have passed the discontinuity? Do they recombine and produce heat? Do they pass undisturbed and continue flowing as electric current? In the first stack question it's written that there is a loss of carriers. How can the KCL law be true if some carriers disappear at the discontinuity?

Answer 1

when the diode is forward biased, holes are pushed from P to N and electrons from N to P, correct?

Yes. [However, "forward biased", really only means that the P side is more positive than the N side. When the forward bias is very small, "leakage" current may predominate. Leakage current is caused by minority carriers. Although majority carriers are (usually) more common (hence "majority") they have a harder time crossing a PN junction than minority carriers. So, when the bias is very low, the few minority carriers that easily cross the junction may form a larger current than the many carriers that have a hard time crossing the junction.]

what do the charge carriers injected in each semiconductor do after they have passed the discontinuity?

They become minority carriers. There are multiple fates for such minority carriers. They may recombine with majority carriers, they may diffuse back across the junction, they may survive and reach the metal-semiconductor junction.

Do they recombine and produce heat?

Whatever their fate, they will eventually interact with the crystal lattice, with other carriers, with metal, etc, and their trajectory will become randomized. That is they will produce heat. However, the electric field will cause those carriers or new carriers to accelerate, thus maintaining the current.

How can the KCL law be true if some carriers disappear at the discontinuity?

When an electron and a hole recombine (at the discontinuity or elsewhere) there is electron current in one direction and conventional current in the other. So algebraicly, current is conserved.