Transfer function of three cascaded filters (including loading)

Question

I am looking for the transfer function Vout / Vin of the following system, including loading. Could you help me find it?

I have tried deriving the equation myself, but the derivation is too complex because of the parallel inductors and resistances, and it gets very messy very fast when using Kirschoff's laws to derive it. Maybe there is a book somewhere with this transfer function already given?

A similar question has been asked before here, but in that case there were no inductors involved. One of the answers recommended using fast analytical circuits techniques, and I'm not sure how to use them in this case.

Answer 1

"There's only one way to rock", as old Sammy says and here it's called the fast analytical circuits techniques or FACTs. You can try to use Thévenin to extract the transfer function and it will surely work provided you have a math solver. But if you do it by hand, you may quickly reach algebraic paralysis and even a good glass of wine won't help.

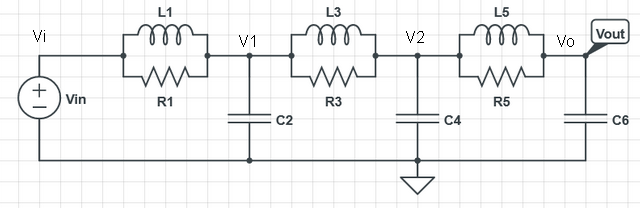
A quick introduction to the FACTs was given in my APEC 2016 seminar that you can look at. It details how poles and zeroes can very often be determined painlessly, without writing a single line of algebra. Look at your circuit below:

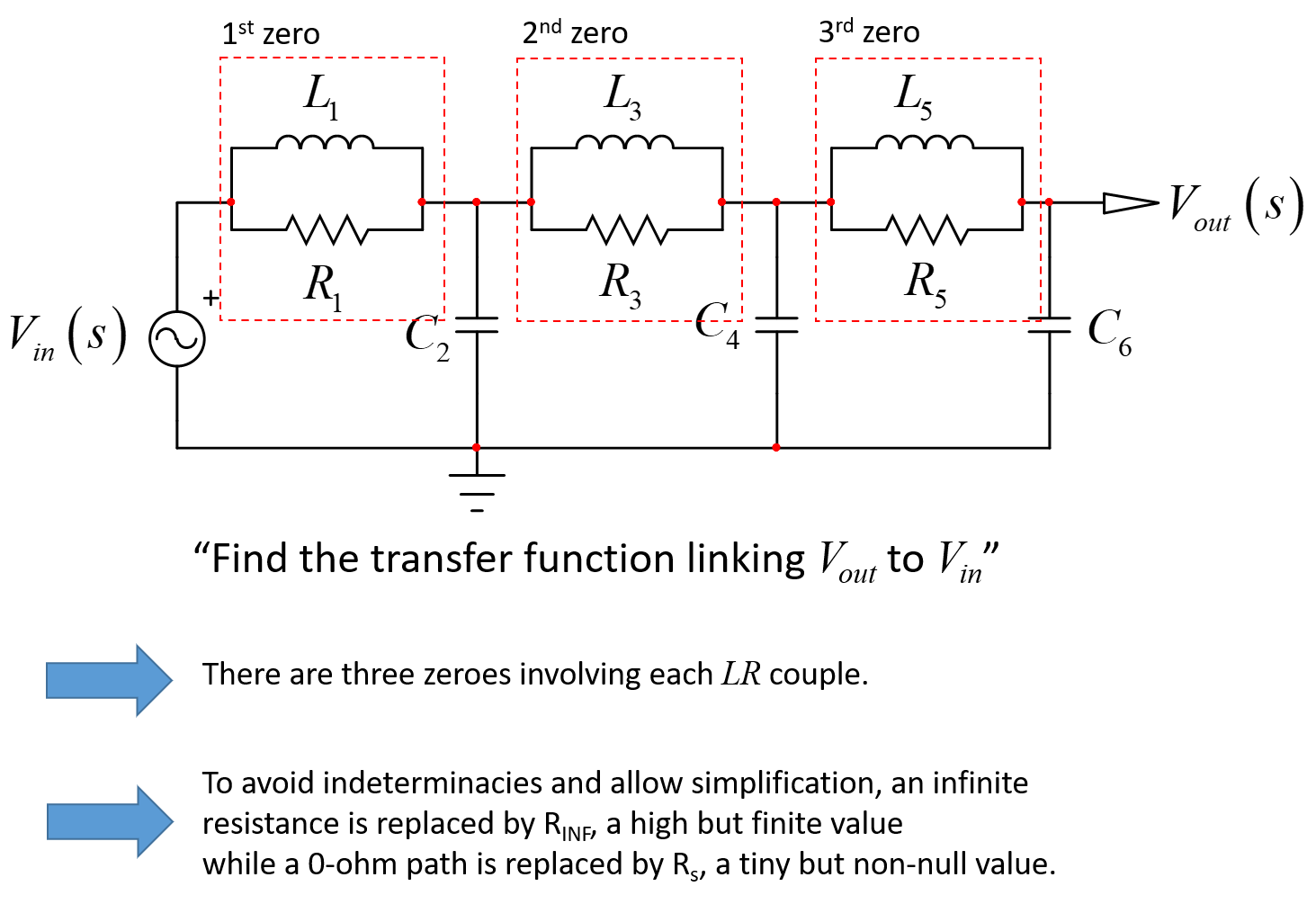
Just by looking at it - and it's not the prestige : ) - I can see 3 zeroes and a unity dc gain:

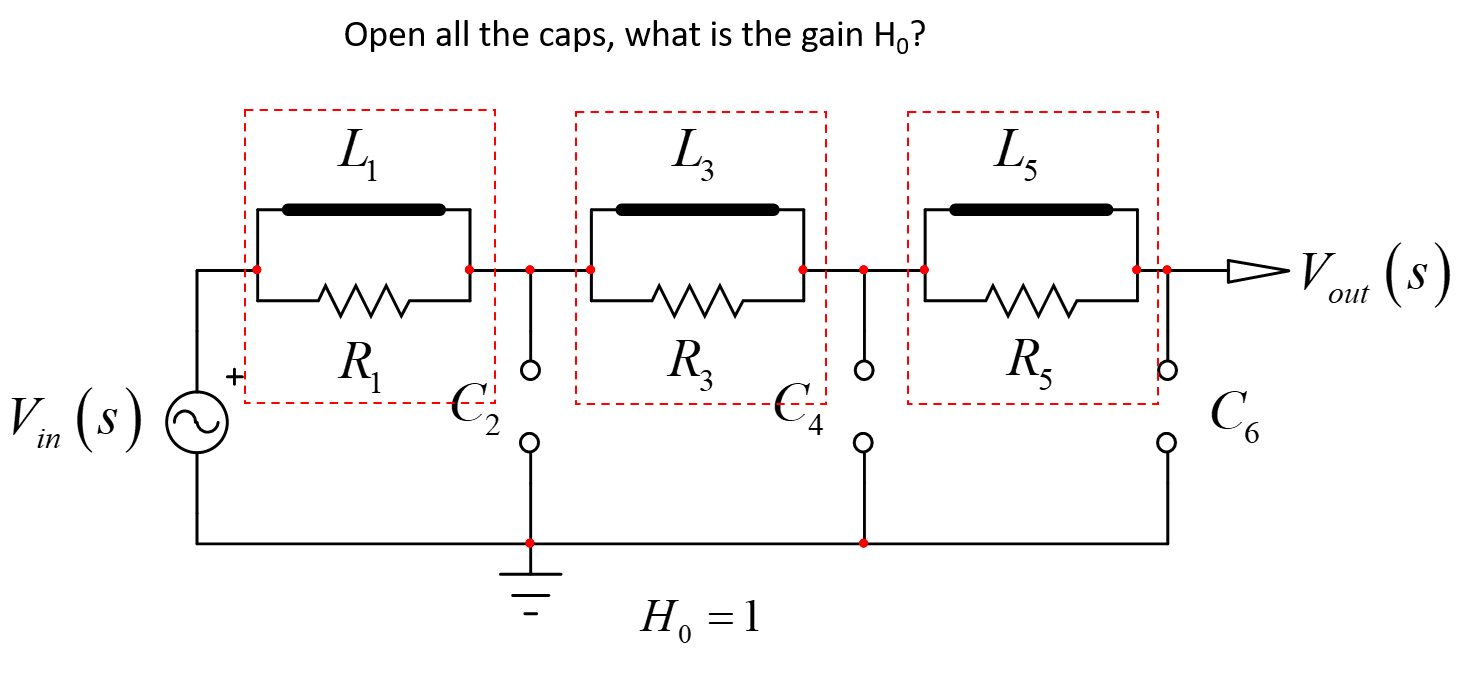
The zeroes are determined by finding in this circuit one or several particular impedances which can become either an infinite impedance in the path of the stimulus or a transformed shunt, bringing one branch to the ground. Both of these behaviors null the response. It means that despite a stimulus present at the input, it is lost somewhere in the circuit and the output is 0 V ac. By inspecting the circuit, I can already write the numerator:

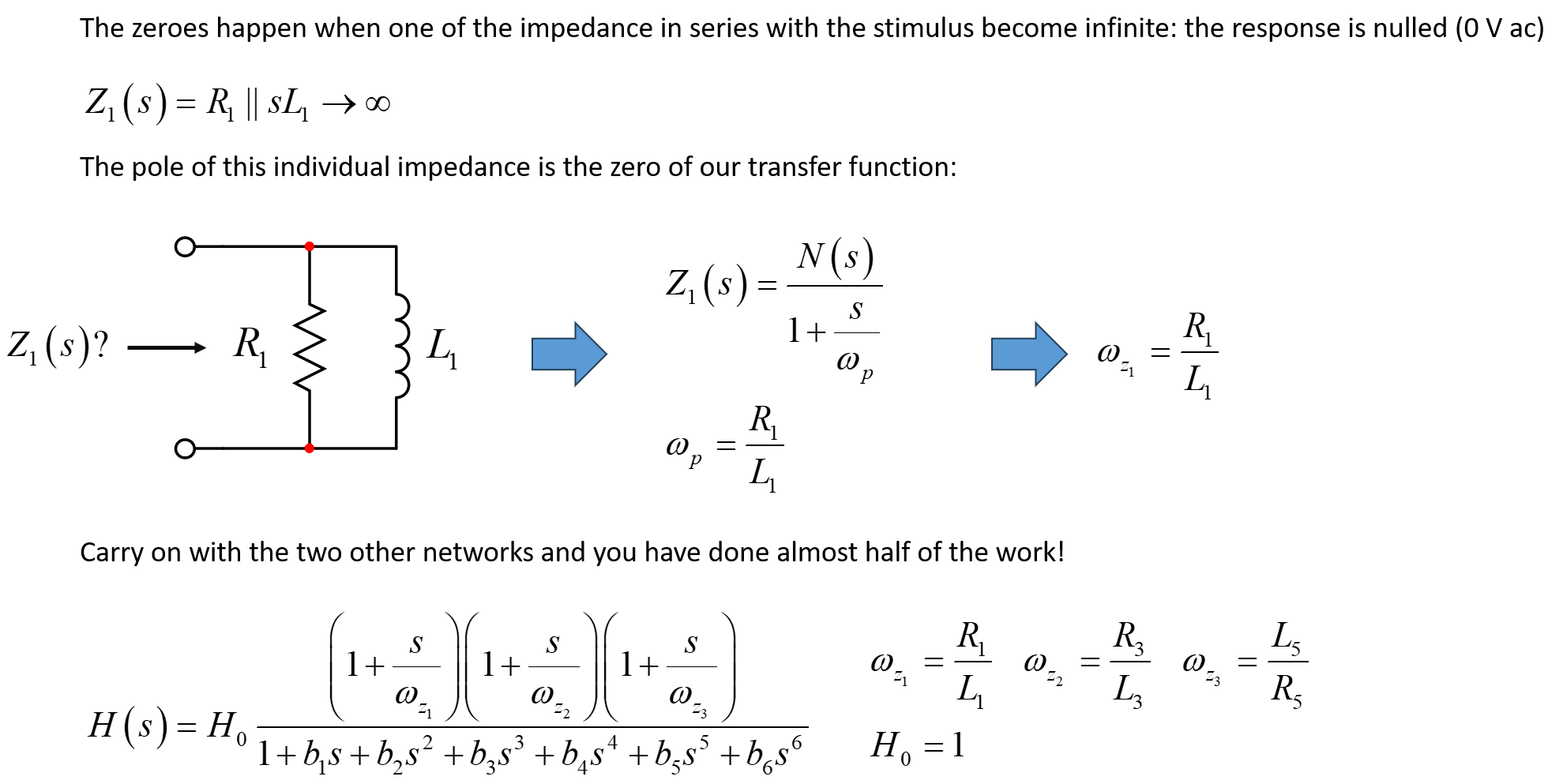
Should you add a small resistance \$r_C\$ in series with \$C_2\$ for instance, you would introduce a 4th zero.

The principle is now to determine the time constants of the circuit when each energy-storing element is set in its dc or high-frequency state (read the seminar) and the stimulus is reduced to 0 V (\$V_{in}\$ is replaced by a short circuit). You will obtain the natural time constants of this circuit which depends solely on the structure of the circuit, without its excitation. Here, we have 6 energy-storing elements with independent state variables so the denominator is of degree 6. I will show you how to determine \$b_1\$ but I'll leave the rest to you:

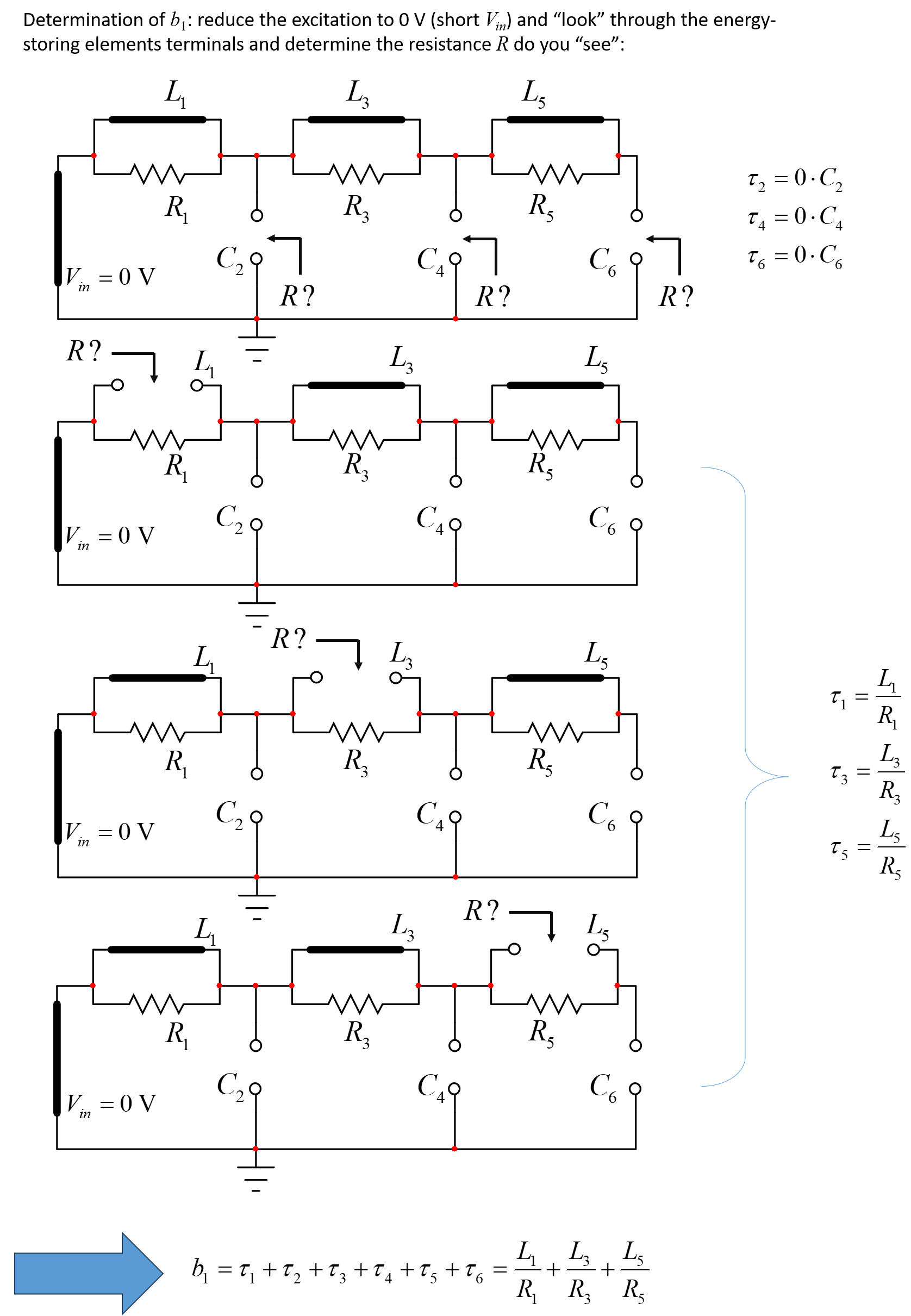
Then you can proceed with \$b_2\$ and follow the explanations I gave in this solved 6th-order example from my web page here. And if you want to adopt the FACTs - highly recommended - then you can have a look at my book on the subject.

If you go step by step or petit à petit in French and carefully craft your drawings, you will make it. The cool thing is that if you find a mistake in the end, no need to restart from scratch, just fix the guilty sketch and see all curves superimposing as in my example, just by magic! Good luck with this example.

Answer 2

Much as I admire Verbal Kint's fast analytical techniques, I find that I don't analyze enough circuits on a regular basis to keep skills like that honed so that I can confidently use them. If I NEED a symbolic analysis of a circuit like this I would either paper bash using ABCD matrices (noting that you only need one entry of the final matrix, hence you only need one row of the first matrix multiplication), or use something like Maxima (free). With Maxima I can write the nodal equations by inspection (something I find easy to remember), and solve them in a few minutes.

Labeling the nodes:

e1,e2 and e3 are the nodal equations for nodes V1, V2 and Vo respectively, which are then solved and the expression for Vo in terms of Vi extracted.

You can then mess around to try to put it into a form you like.

Answer 3

The most straightforward method I can think of using circuit analysis techniques would be to combine the impedances of the parallel parts then use nodal analysis.

It will look ugly, I would recommend using abstractions until you get to your final answer, then substitute back in. For instance, \$z_1 = \frac{R_1 L_1^2 \omega ^2 + jR_1 ^2 L \omega}{R_1 ^2 + L_1 ^2 \omega ^2}\$.

Once you've done that simplification, use nodal analysis, simplify, and substitute instances of \$j \omega\$ with \$ s \$ and rearrange to get your transfer function.