The impedance of the capacitor is just \$\frac1{2\pi\,f\,C}\approx 4.194\:\text{k}\Omega\$. Clearly, that's dominant. (Besides, the resistor is orthogonal. So won't affect this figure much, at all.) The peak current then works out to \$\frac{90\:\text{V}}{4.194\:\text{k}\Omega}\approx 21.46\:\text{mA}\$ or \$15.175\:\text{mA}_\text{RMS}\$.
By why believe that?
To work out the current in the capacitor, solve the KCL:
$$\begin{align*}
\frac{V_{_\text{C}}}{R}+C\frac{\text{d}}{\text{d}t}V_{_\text{C}}&=\frac{V_p\sin\left(\omega_{_0}\,t\right)}{R}
\\\\
\frac{V_{_\text{C}}}{R\,C}+\frac{\text{d}}{\text{d}t}V_{_\text{C}}&=\frac{V_p\sin\left(\omega_{_0}\,t\right)}{R\,C}
\\\\
\left[\frac{\text{d}}{\text{d}t}+\frac{1}{R\,C}\right]V_{_\text{C}}&=\frac{V_p}{R\,C}\cdot\sin\left(\omega_{_0}\,t\right)
\\\\
\left[\frac{\text{d}^2}{\text{d}t^2}+\omega_{_0}^2\right]\left[\frac{\text{d}}{\text{d}t}+\frac{1}{R\,C}\right]V_{_\text{C}}&=0
\end{align*}$$
The solution to the above homogeneous equation is:
$$\begin{align*}V_{_\text{C}}&=A_0\exp\left(-\frac1{R\,C}\cdot t\right)+A_1\cos\left(\omega_{_0}\,t\right)+A_2\sin\left(\omega_{_0}\,t\right)
\\\\
&=\frac{V_p\cdot \omega_{_0}\cdot R\,C}{1+\left(\omega_{_0}\cdot R\,C\right)^2}\left[\exp\left(-\frac1{R\,C}\cdot t\right)-\cos\left(\omega_{_0}\,t\right)\right]+\frac{V_p}{1+\left(\omega_{_0}\cdot R\,C\right)^2}\sin\left(\omega_{_0}\,t\right)
\end{align*}$$
(The solution for \$A_0\$, \$A_1\$, and \$A_2\$ comes from further analysis that I've skipped providing. If you sincerely care and want to see how that is also developed, I can add it.)
In your case, the term \$\omega_{_0}\cdot R\,C\$ is small -- about 0.00239. Even multiplied by \$V_p\$ it only reaches about 0.2. And when squared it doesn't even come close to 1, so \$1+\left(\omega_{_0}\cdot R\,C\right)^2=1.00000569\$.
As \$t\to\infty\$, the exponential part disappears and the voltage across the capacitor will be:
$$\begin{align*}V_{_\text{C}}
&=V_p\cdot\left[\frac{1}{1.00000569}\sin\left(\omega_{_0}\,t\right)-\frac{1}{419.383}\cos\left(\omega_{_0}\,t\right)\right]
\end{align*}$$
So the current through the capacitor will be:
$$\begin{align*}I_{_\text{C}}
&=21.460\:\text{mA}\cdot\cos\left(\omega_{_0}\,t\right)+51.171\:\mu\text{A}\cdot\sin\left(\omega_{_0}\,t\right)
\end{align*}$$
These two terms are orthogonal to each other, so the peak can be taken to be simply \$21.460\:\text{mA}\$. This peak occurs twice per cycle. But it is still going to be an RMS current of about \$15.175\:\text{mA}\$.
(All of this was much, much easier to work out by just assuming that the capacitor determined the current, ignoring the resistor, and getting to the exact same place using its impedance calculation that I used at the outset. The reason I also added the long path above is to firmly show the nuanced details in the equations, should you care to ask yourself questions about what happens when you make changes and ask different questions. You have enough here to ask any question you want, now.)
Of course, \$I_{_\text{R}}=I_{_\text{C}}\$ so the power in the resistor will be \$I_{_\text{C}}^2\cdot R\$ or, in this case, about \$2.3\:\text{mW}\$.
I've no idea where you came up with the requirement that "The energy dissipated by the resistor is the energy stored in the capacitor." It doesn't make any sense to say that because then the resistor value itself doesn't seem to matter at all. (Even assuming you limit that statement to only when your \$5\tau\$ condition was met.) So it should not even pass the sniff test.
As you can see from the above, the resistor value does in fact actually matter. If you made it \$10\times\$ larger, the RMS current would only slightly drop to about \$15.166\:\text{mA}_\text{RMS}\$ but the resistor would be \$10\times\$ larger, so the power dissipated would be close to \$10\times\$ more.
Here's an LTspice run to confirm:
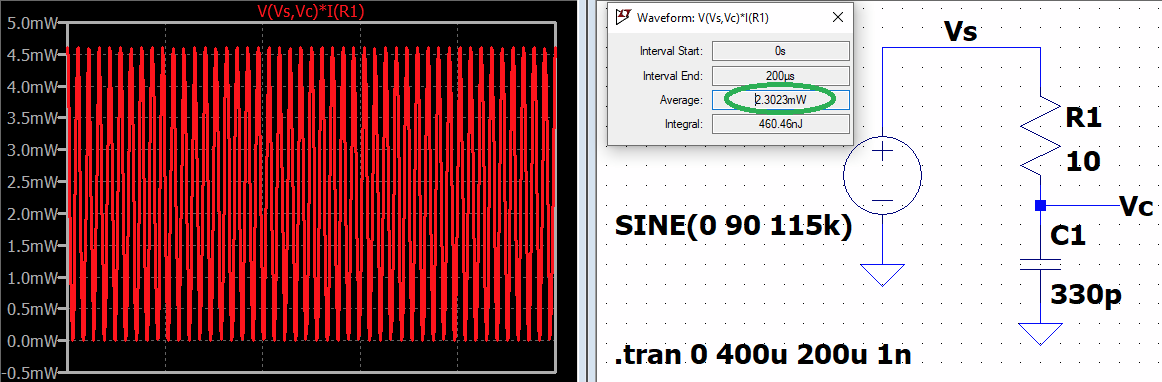
Suppose we change \$R\$ so that it is \$4.7\:\text{k}\Omega\$ and closer to the impedance of \$C\$ at this frequency. The resulting current is:
$$\begin{align*}I_{_\text{C}}
&=9.513\:\text{mA}\cdot\cos\left(\omega_{_0}\,t\right)+10.661\:\text{mA}\cdot\sin\left(\omega_{_0}\,t\right)
\end{align*}$$
These are orthogonal again. But now the actual computation is needed to work out the peak current. So \$\sqrt{\left(9.513\:\text{mA}\right)^2+\left(10.661\:\text{mA}\right)^2}\approx 14.288\:\text{mA}\$ and we should expect that as a peak value. The RMS should then be \$10.1031\:\text{mA}_\text{RMS}\$ and we'd estimate the power in the resistor to be \$479.7\:\text{mW}\$.
Here's LTspice's report:
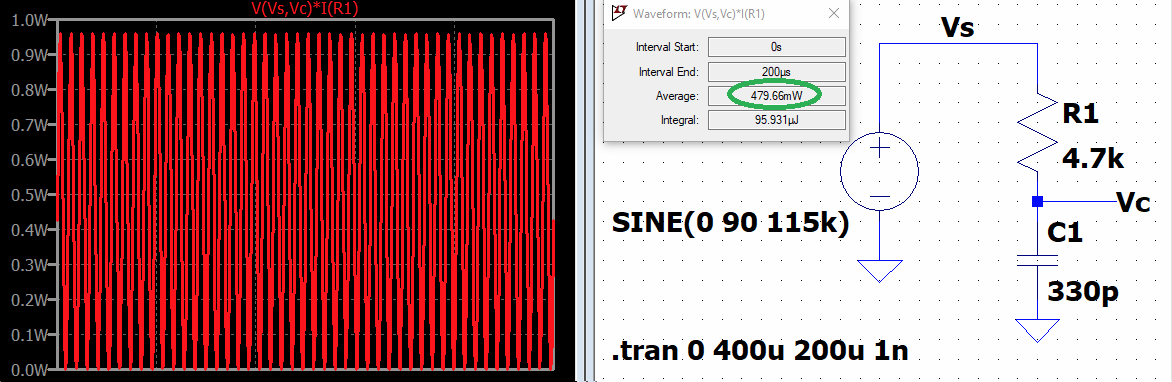
Same result!
Using the impedances, instead of all that mathy stuff, we'd compute that the total impedance is \$\sqrt{\left(4.7\:\text{k}\Omega\right)^2+\left(4.194\:\text{k}\Omega\right)^2}\approx 6.299\:\text{k}\Omega\$ and from that the fact that the current peak should be \$\frac{90\:\text{V}}{6.299\:\text{k}\Omega}\approx 14.288\:\text{mA}\$. That's the same as before.
It all works out the same, either way. One method provides the instantaneous results. The other, the average (for power.)


