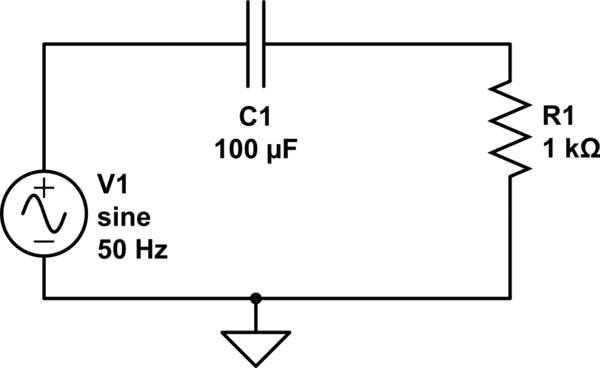
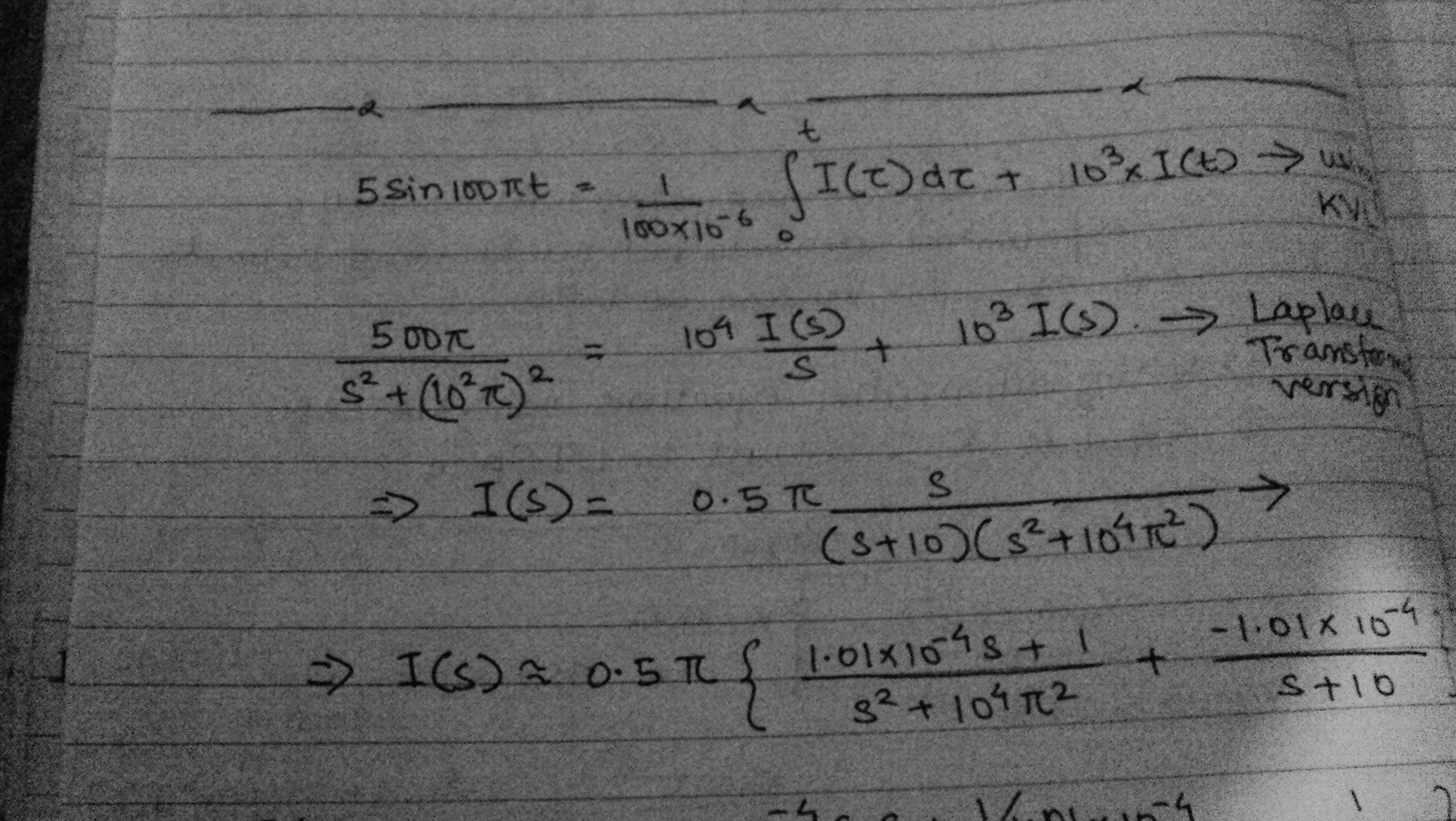The transient part is needed because before and at time 0 the capacitor voltage is 0V.
At times tn = n / omega is should also be 0 volt had you only the steady state in your description. But it is not 0 - the transient part describes the details of this. Imagine the phase fi of the sin(w t + fi) had not been 0 or pi it would have an abrupt voltage step at time 0. You need at transient portion to describe how this affects the response.
But seeing your derivation I have one even more important piece of general advice to give: Always derive your equation using symbols as long as possible!
So in your case stick with R1, C1, V1 and f labels in the equation and try to solve them analytically. Then insert numerical values into the final equation.
Now of course it is not always possible to do this due to the complexity of the equation.
But doing so:
- gives additional insight into your system having the parameters - like answer the questions - how does the different values affect the solution.
- aids in checking that the equations are reasonable as you can verify that the units match up - unit checking is a very power full tool to validate any equations with.
To give an example for point 1) from your particular derivation:
A reasonable question to ask after solving this equation is - why is the transient term x exp(-10t)? Where does the -10 come from? You can only tell by looking back through your derivations to figure that out.
But had you solved using the symbols you would find the term being
x exp(-t / (R1 * C1)) immediately giving insight into how R1 and C1 affect the transient's time response. Plus - you can see that the units are correct as the unit of RC is seconds so the argument for the exponential is sec / sec or unitless as it must be.
To illustrate point 2) from your example:
Suppose you made a mistake and ended up with an equation for transient of form x exp(-t / (R1 * R1 * C1)) you would immediately be able to spot that this cannot be correct just by looking at the units. Your unitless numbers removes this insight.
I cannot overstress how powerful this is in general for checking equations of physical properties.
So all that said - technically speaking your equation is incorrect because your numbers are unitless. Had you sticked in a proper way to derivation using only numbers, your transient term should read
x exp(-10[1 / sec] t) using units (Hz or 1 / sec). Personally I would use 1 / sec here.