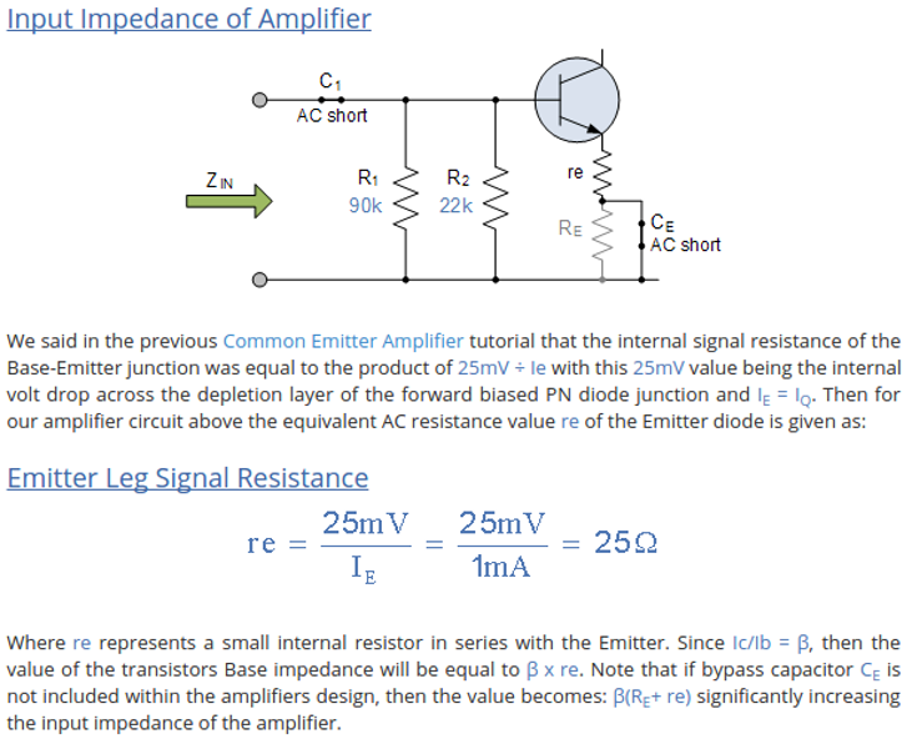
My recommendation: Forget the link which gaves you the above information, which is false resp. misleading. (By the way: This link leads you to other "explanations" which also are wrong). Hence, you should not blindly trust any information available in the internet.
The text says that the "25 mV value being the internal voltage drop across the depletion layer of the forward biased pn diode junction". That`s pure nonsense.
This value of 25 mV is the so-called "temperature voltage VT" which depends on the environment temperature and appears in the exponent of the e-function describing the relation between the controlling base-emitter voltage and the emitter current.
And what about the "resistance" re, which appears in the above figure outside the transistor?. In fact, it is NOT a resistance - it is the inverse of the transconductance gm=1/re - and some people prefer the use of re instead of gm. Note that the transconductance gm=d(Ic)/d(Vbe) is nothing else than the SLOPE of the transfer curve Ic=f(Vbe) - measured in the selected DC operating point.
More than that, it can be easily shown that the slope d(Ic)/d(Vbe) is identical to gm=Ic/Vt (VT: temp. voltage); this gives you the relation between gm=1/re and VT.
Hence, gm is the most important parameter which determines gain. It relates input voltage and output current (therefore, it is called "mutual" transconductance gm). This can be seen in the known gain formulas (common emitter):
(a) without feedback: Gain=-gm*Rc
(b) With feedback (emitter resistor Re): Gain=-gmRc/(1+gmRe)
(Sometimes you can read: (a) -Rc/re and (b)-Rc/(Re+re) ).
EDIT: Differential input resistance at the base node (without feedback resistor Re):
The input characteristic of the BJT is also exponential with the slope
1/rbe=d(Ib)/d(Vbe)=(1/beta)[d(Ic)/d(Vbe)]=gm/beta.
Hence: rbe=beta/gm (or: rbe=beta*re).
Final comment: I think, this post is a typical example for the confusion which can be caused by using such "artificial" terms like re which have no physical meaning.