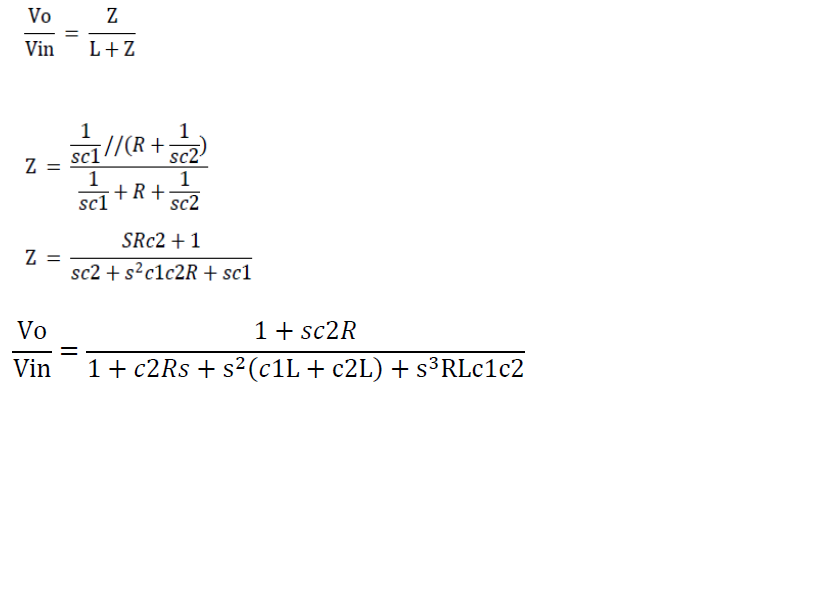 Hi
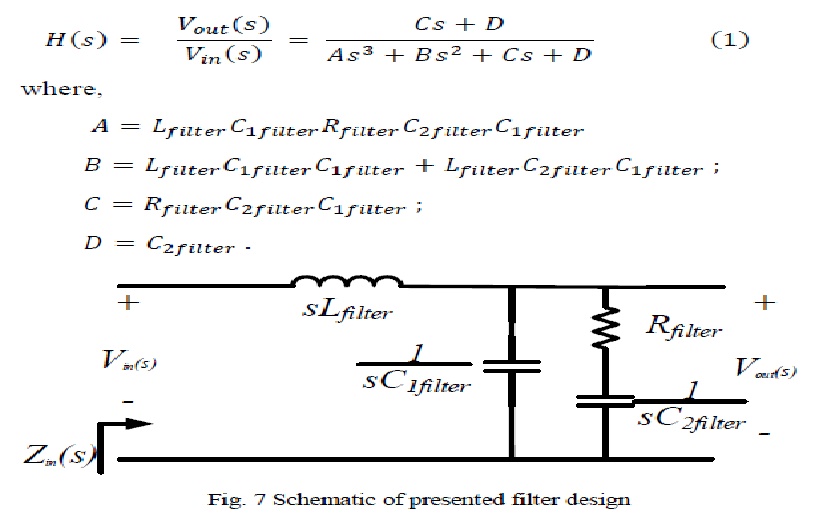
I have tried to extract the transfer function of the filter above, as you see it a high order filter.
Hi
I have tried to extract the transfer function of the filter above, as you see it a high order filter.
I have combined the \$C_1\$ in parallel with \$R\$ & \$C_2\$ then make a voltage divider in order to add the inductor. I always miss the capacitor or I don't get the same result. Where is my mistake?