1
\$\begingroup\$

I am reading about common gate amplifier on this page and there is a small signal model that I am a bit confused.

The common gate amplifier and its small signal model is shown below.

As you can see there is an open circuit resistance \$r_{oc}\$ at the output of the small signal model.

Could anyone tell me where does this resistor come from?

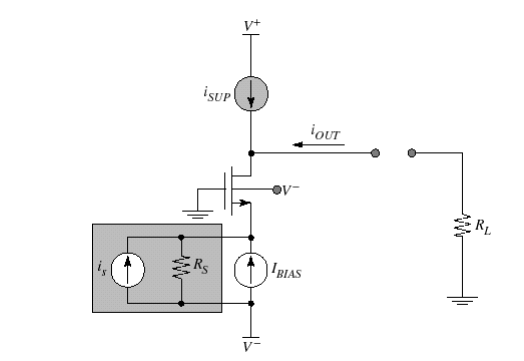
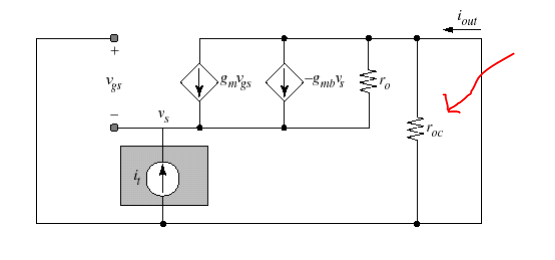
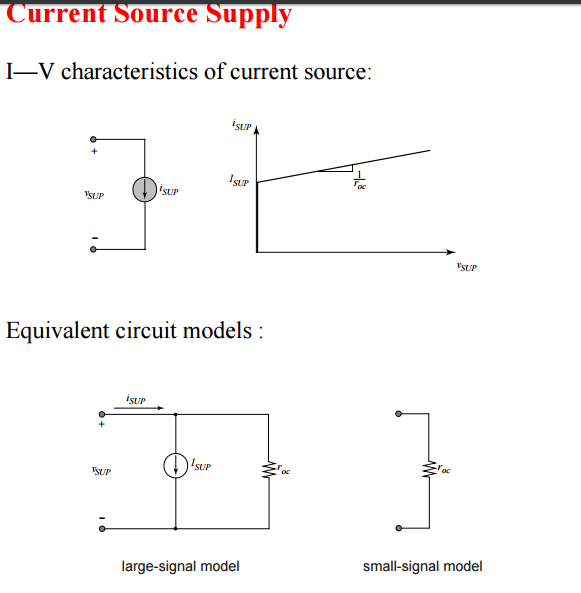
enter image description here

enter image description here

\$\endgroup\$
1
  • \$\begingroup\$ Leakage, creepage, etc. \$\endgroup\$
    – Ignacio Vazquez-Abrams
    Commented Jun 23, 2016 at 1:12

3 Answers 3

Reset to default
3
\$\begingroup\$

Here they have defined as the internal resistance of current source.

enter image description here

As in the circuit gmb and r0 is already included to capture the effect of parasitic and body-bias. So this can have a chance. But still, I am not sure whether these two documents are following the same notation.

\$\endgroup\$
2
  • \$\begingroup\$ It's the resistance of current source Isup in your question. \$\endgroup\$
    – Virange
    Commented Jun 24, 2016 at 14:57
  • \$\begingroup\$ While I submitted another answer here, I think Virange's answer is the correct one in this case - it is probably the internal resistance of Isup. \$\endgroup\$
    – Sagie
    Commented Jul 4, 2016 at 12:21
2
\$\begingroup\$

Yes it is very uncommon to have such a resistor, at least as far as I know. One intuitive reason could be because since the bulk is connected to V- in general there is a very small current flowing from the drain to the bulk instead of the the source. But this current is very small. This could be referenced by that resistor (if you notice,the resistor is between the drain and the V- which is where the bulk is also connected to). I suggest you to refer other sources such as Behzad Razavi which is also available online.

\$\endgroup\$
1
  • \$\begingroup\$ The drain->bulk current you refer to is really the leakage current of an effectively reverse-biased diode. It might have a tiny impact on the DC-equivalent circuit, but for the small-signal model it is absolutely meaningless. Therefore, r_oc can't be related to this leakage current. \$\endgroup\$
    – Sagie
    Commented Jul 4, 2016 at 12:37
1
\$\begingroup\$

Edit: as user "Virange" suggested, the \$r_{oc}\$ resistor probably represents the internal resistance of the current source marked as \$i_{SUP}\$.

While there is indeed, as user "Bhuvanesh N" indicated, a very small leakage from the body/bulk to the drain and the source terminals, \$r_{oc}\$ can't represent this leakage, as this leakage is absolutely meaningless for the small signal equivalent circuit.

The leakage current is essentially the leakage current of a reverse-biased diode - the diode's P being the bulk of the FET, and the diode's N being the FET's source, drain, and n-channel. While one might want to take this leakage current into consideration while solving the DC-equivalent circuit, its effects are completely negligible for the small-signal model.

What is relevant for the small-signal model is the body effect. The body effect means \$V_{SB}\$ changes \$V_{TH}\$, and therefore \$I_{DS}\$. In this specific circuit, the input signal changes \$V_{SB}\$, which introduces another effect on the amplified signal via body effect.

In the small-signal model circuit provided in the question, \$r_{o}\$ represents the channel length modulation (due to \$V_{DS}\$ changes), and the body effect (due to \$V_{SB}\$ changes) is repesented by the controlled current source marked as \$-g_{mb}v_s\$.

\$\endgroup\$
2
  • 1
    \$\begingroup\$ Yes what you're saying is true but this is already condered by having gmb*Vs dependent current source in the diagram. \$\endgroup\$
    – Bhuvanesh Narayanan
    Commented Jun 24, 2016 at 14:05
  • \$\begingroup\$ @BhuvaneshN you're right, I somehow failed to notice the gmb*Vs dependent current source in the small signal equivalent circuit. \$\endgroup\$
    – Sagie
    Commented Jul 4, 2016 at 12:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.