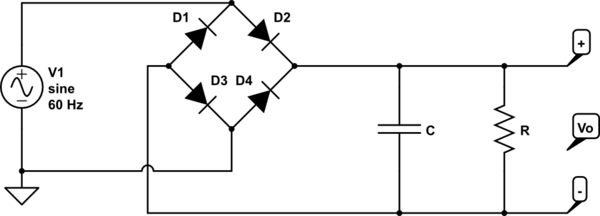
simulate this circuit – Schematic created using CircuitLab
Boylestad's book says that with the diodes conducting the effect of the resistor is "removed" so the \$RC\$ constant is so small that the capacitor charges with the value of of \$V_1\$ extremely fast. Is it a good approximation to say that it charges instantaneously regardless of the value of \$R\$ and \$C\$? Assuming ideal diodes, voltage sources, etc. This is mentioned in the section of clamper circuits with alternating constant voltage sources, would this be different with a sin wave?
If possible I would like to ask something else. Supposing a forward voltage drop of 0.6 in each diode, the peak voltage on Vo would be V1 - 0.6 - 0.6 = V1 - 1.2. If diodes are considered as ideal with 0V drop in forward bias, then Vo = V1 in this case. There is one exercise here made in my classroom where the peak voltage in Vo is different V1, assuming all diodes ideal with 0V drop, ideal sources, etc. Does it make sense? Could the capacitor not be fully charged by the end of a half-cycle? Thanks again.
