Root Locus, Gain in Feedback Loop. Need help

Question

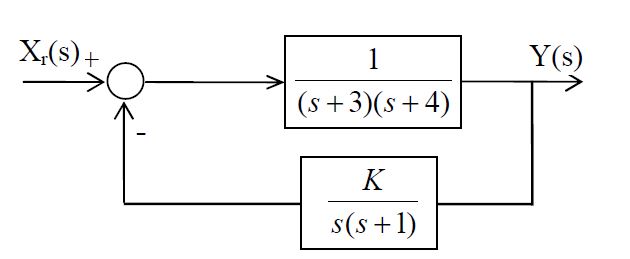
So this is the system for which I have to draw a root locus if 'K' changes from 0 to infinity. The problem for me is that root locus is drawn for open loop transfer function but 'K' is in the feedback loop. I'm guessing that there is a way to get K in open loop transfer function but I've tried everything and just cant do it. I have no problems if K is in open loop tho. I appreciate any help.

Answer 1

No - the root locus is not drawn for the open-loop function.

It is the main purpose of the root locus of a system with feedback to show if resp. for which constant gain values the CLOSED-LOOP function will have poles in the "forbidden" region (right half of the s-plane). Hence, you have to find the poles of the function

H(s)=G(s)/[1+G(s)*H(s)] with G=1/(s+3)(s+4) and H=K(s(s+1).

That means, you have to find the zeroes of the denominator which gives the characteristic equation: 1+G(s)*H(s)=0

Answer 2

When you are working with root locus, you are making a figure about your characteristic equation behavior. So, the root locus of a negative-feedback loop is going to be the same at any point of the loop. Notice: the name 'characteristic equation' is just because of that. In your case, we have: characteristic equation = \$1 + \frac{1}{(s+3)(s+4)} \frac{K}{s(s+1)}\$ if K was in the open loop this will be the same.

Answer 3

In order to draw the root locus, you need to convert the open-loop system into the closed-loop system. You can do this

$$ \begin{align} T(s) &= \frac{ \frac{1}{(s+3)(s+4)} }{ 1+ \frac{1}{(s+3)(s+4)} \frac{K}{s(s+1)} } \\ &= \frac{s^2+s}{s^4+8s^3+19s^2+12s+K} \end{align} $$ Now the gain K appears in the characteristic equation of the closed-loop \$T(s)\$ (i.e the denominator). You can easily vary K from 0 to infinity to compute the poles of the characteristic equation of the closed-loop \$T(s)\$ using any software (e.g. Matlab). If the gain K is zero, we have these poles 0,-1,-3,-4. Use this Matlab script to compute the poles at different gains

K=0; % vary it from 0 to big number
poles = roots([1 8 19 12 K])

You just need tabular data to plot the root locus. You may use Matlab as well.

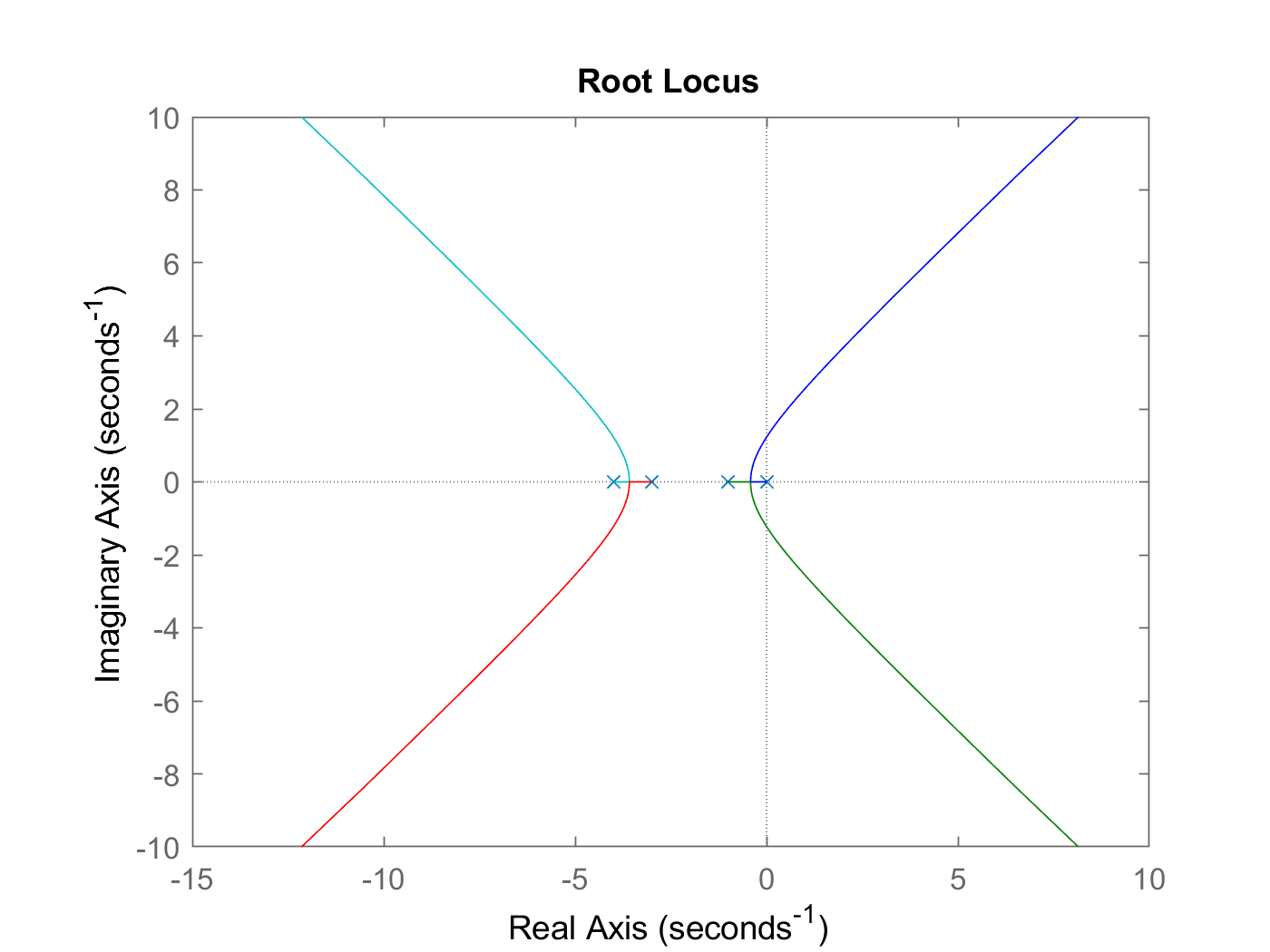
GH=zpk([],[0 -1 -3 -4],1);
sys=tf(GH);
rlocus(GH)

Or this elegant code

s=tf('s');
G = 1/((s+3)*(s+4));
H = 1/(s*(s+1));
sys = G*H;
rlocus(sys)

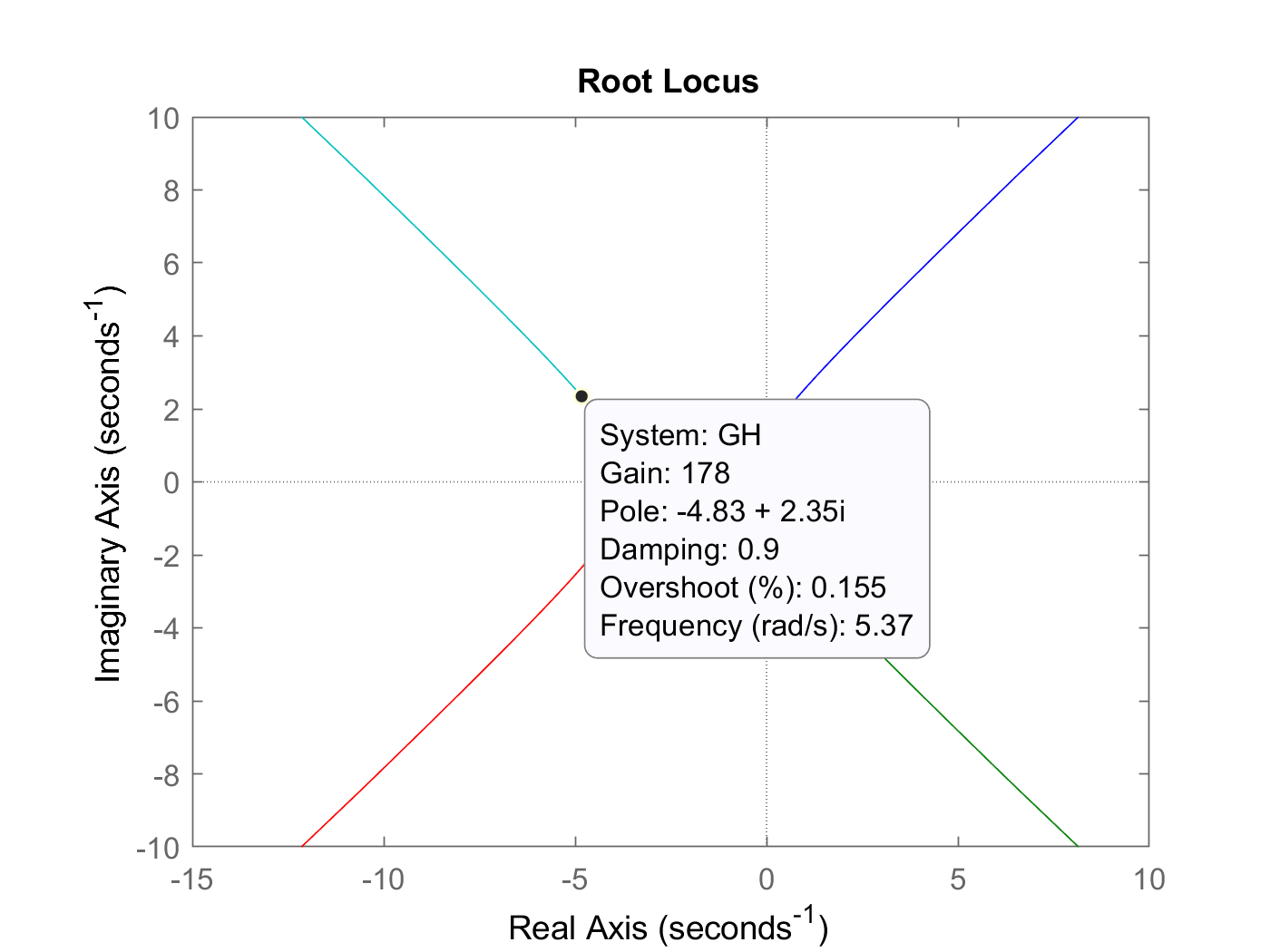
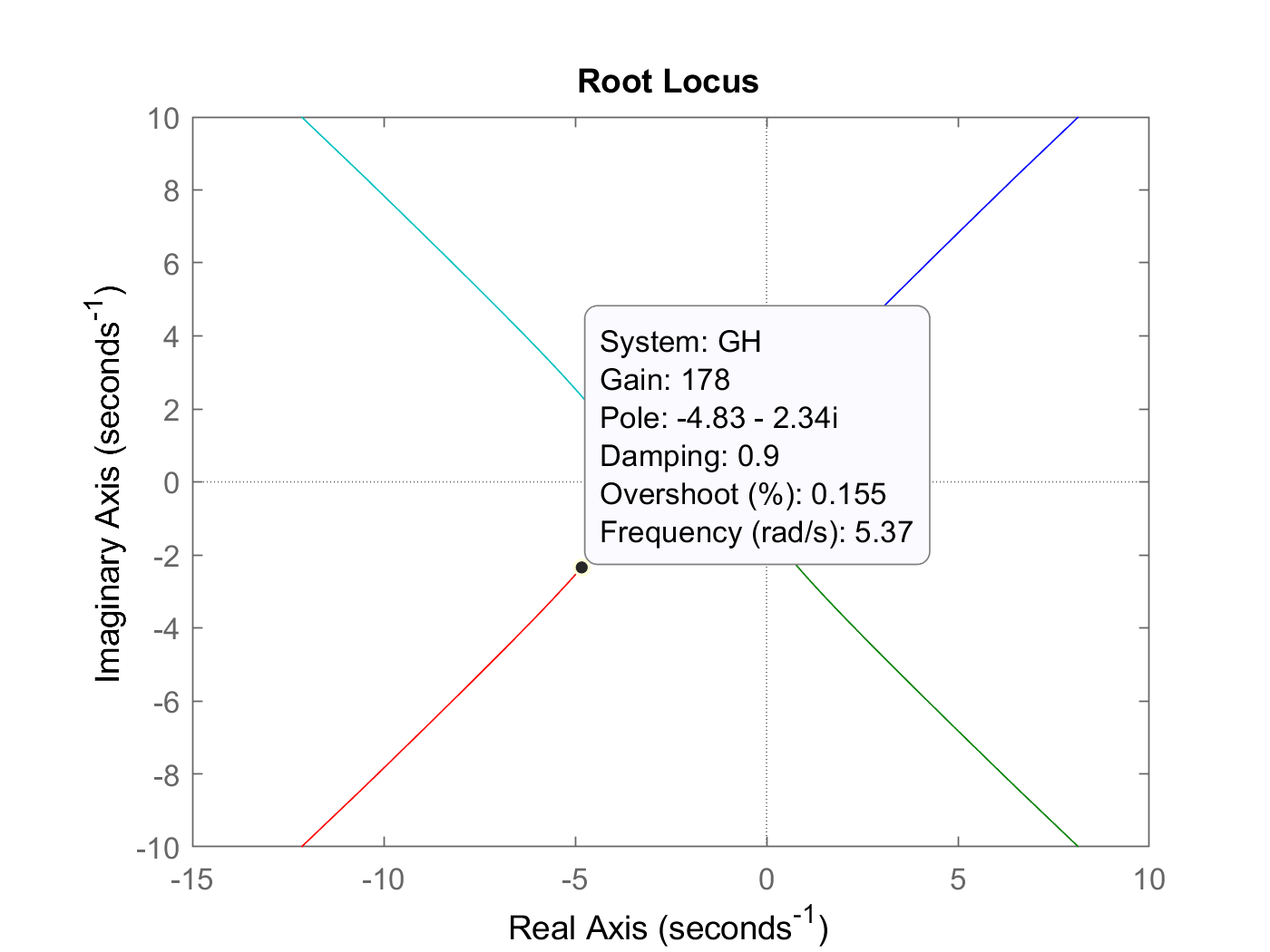
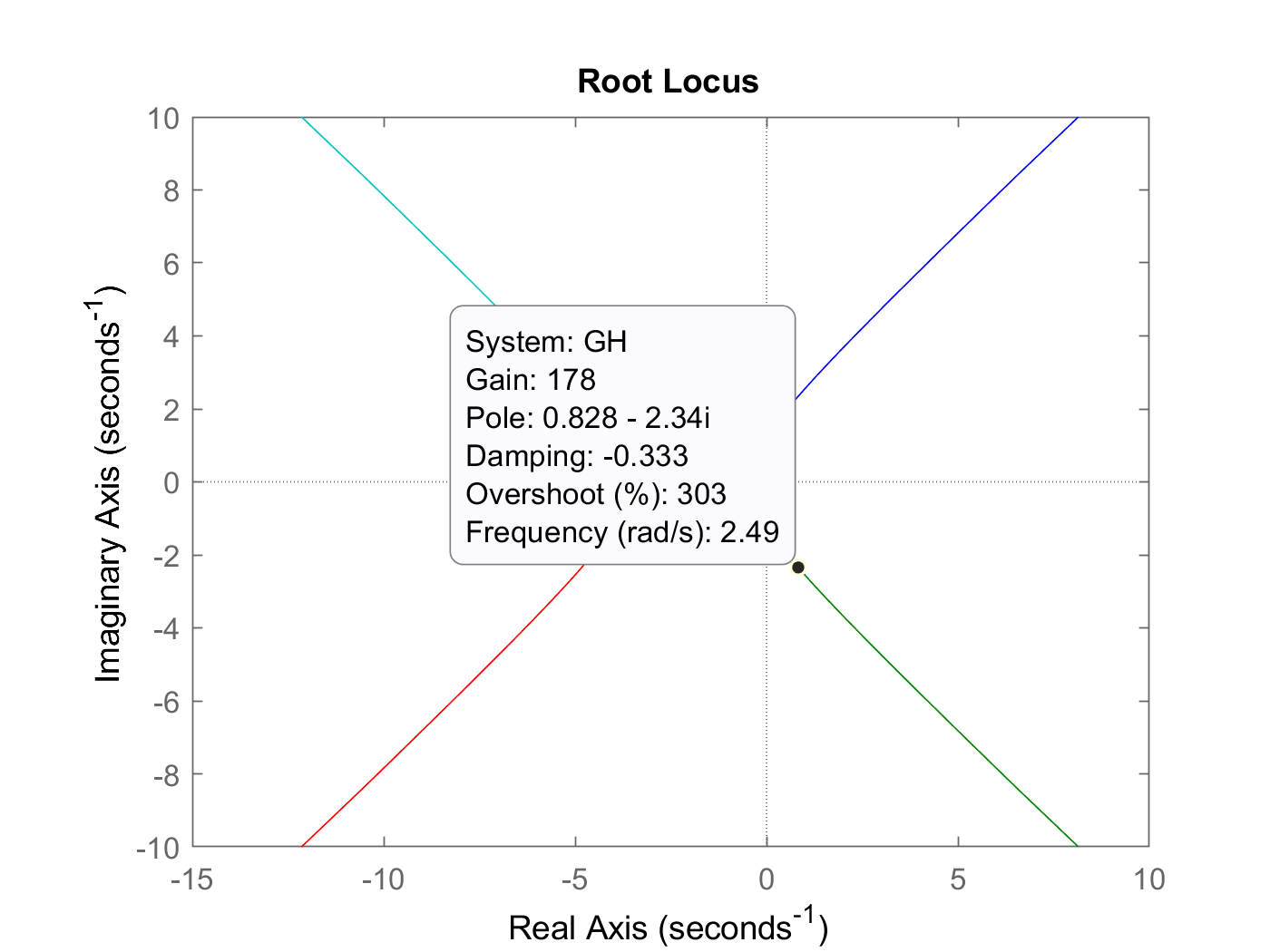
Let's go back to roots([1 8 19 12 K]) if K is 178, we have these poles

  -4.8276 + 2.3442i
  -4.8276 - 2.3442i
   0.8276 + 2.3442i
   0.8276 - 2.3442i

We can see same result in the root locus obtained by Matlab at this gain,