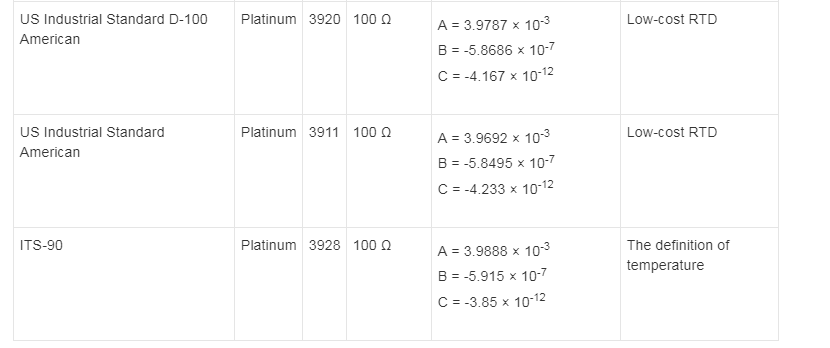
I'm currently using a cubic fit formula I found online to derive the temperature of a PT100/385 RTD using resistance. The formula is -247.29 + 2.3992*R + 0.00063962 *R^2 + 0.0000010241 *R^3 where R is Ohms. Does anyone know where I could find a similar formula for a 39250 RTD? I've tried to find one online, but I have had no luck.
I need to use this instead of the standard Linear one because I need more accurate readings at high temperatures.