1
\$\begingroup\$

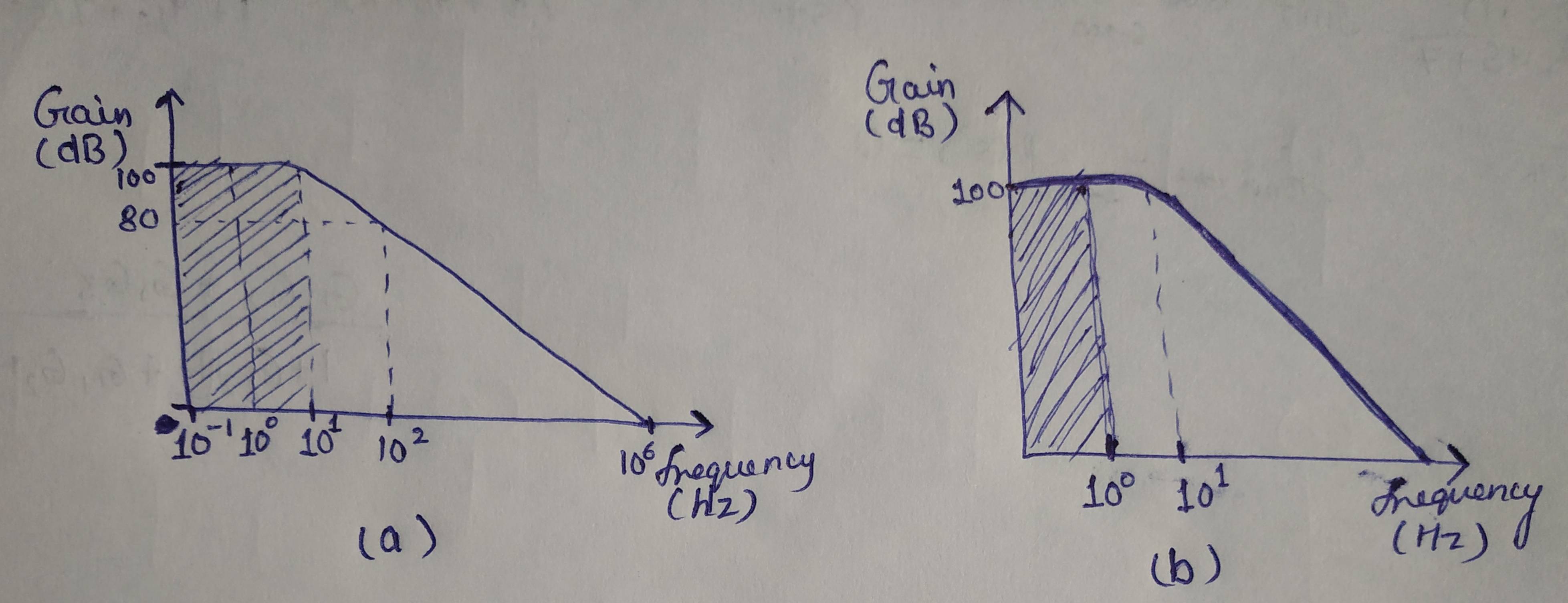
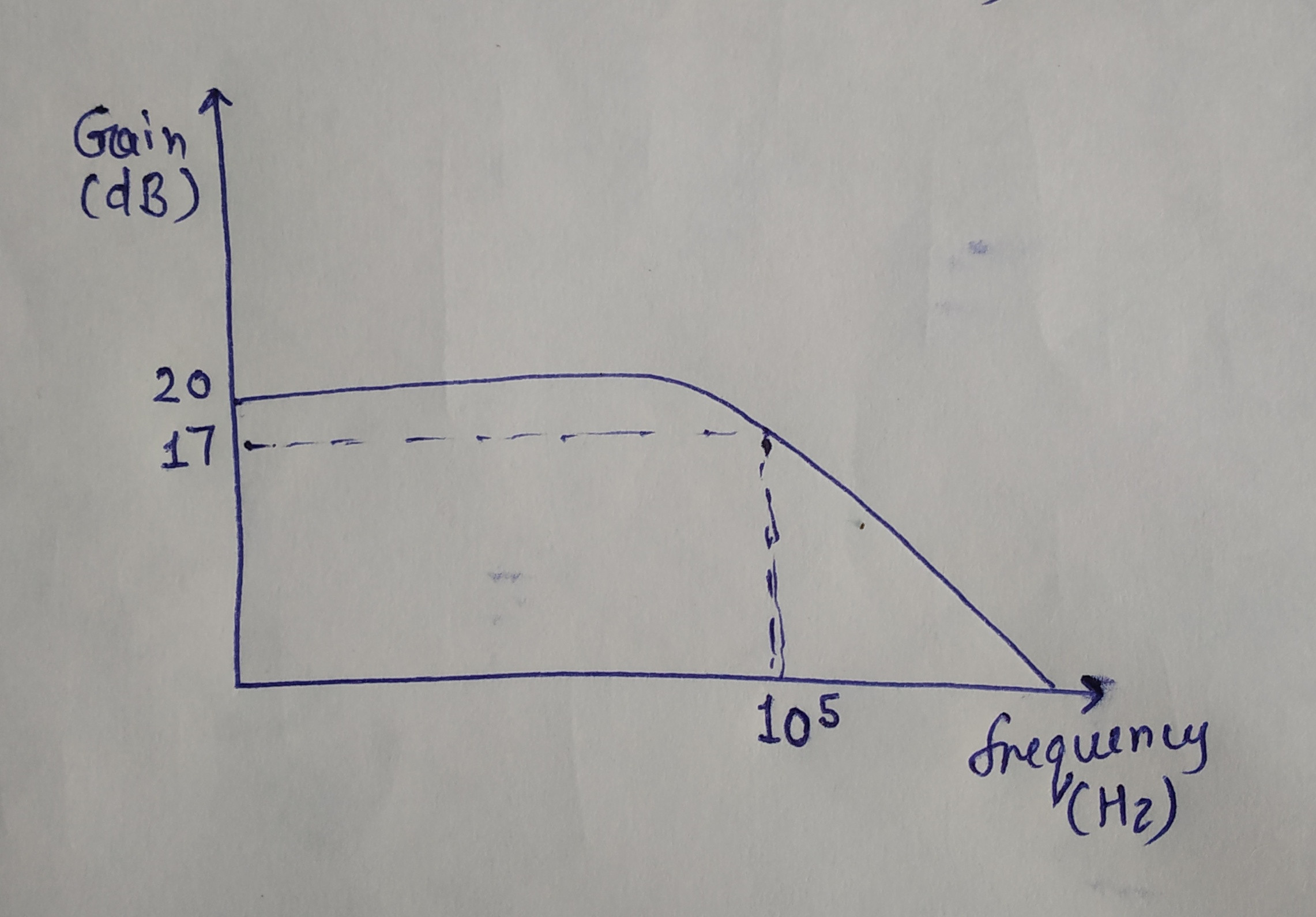
The gain and bandwidth product of an ideal op amp is always constant. This statement seems to be true in the '-20dB slope' region. But this doesn't seem to be true for the low frequency region. The two shaded regions below doesn't seem to represent the same gain bandwidth product. enter image description here

I am not confused here with plot for gain at a particular bandwidth, which may seem as below and does not satisfy the gain bandwidth product (it should not) in the constant gain region.

enter image description here

What I think (I know I am wrong) why isn't the gain bandwidth product made something like this:

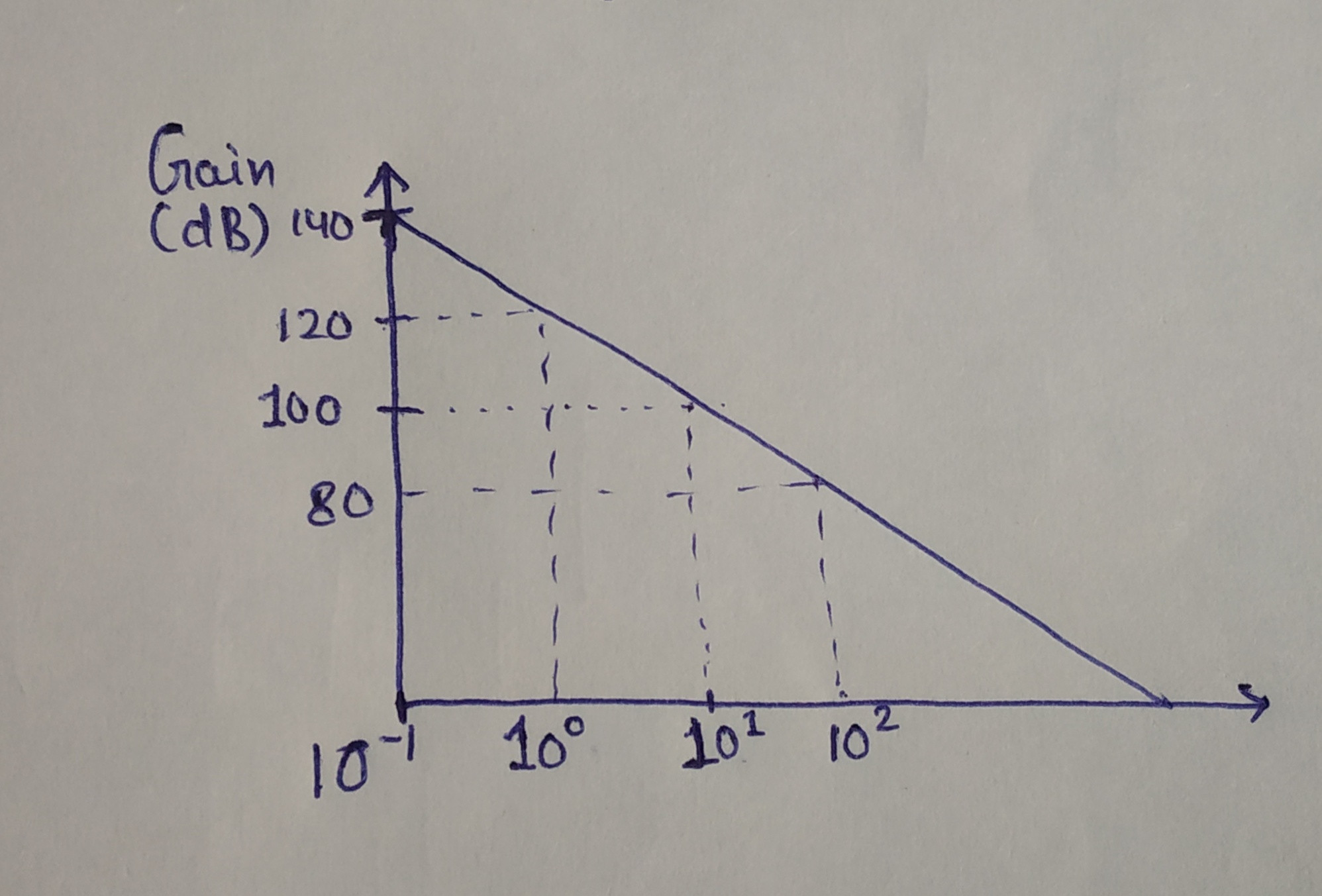
enter image description here

Can anyone please explain where am I wrong in my reasoning?

\$\endgroup\$
3
  • \$\begingroup\$ Insert the word "ideal" ahead of "op amp" in your first sentence. An ideal op amp has infinite open loop gain at DC. A real one doesn't. \$\endgroup\$
    – user16324
    Commented Oct 13, 2019 at 10:39
  • 1
    \$\begingroup\$ iirc: For an ideal opamp the gain is infinite and the bandwith is also infinite. Therefore, there is no GBWP defined. If a real opamp can be approximated by an integrator with gain, the GBWP only applies for \$ \omega > \omega_c \$ \$\endgroup\$
    – Huisman
    Commented Oct 13, 2019 at 17:56
  • \$\begingroup\$ opamps with infinite DC gain will simply exhibit massive thermal instability, as the output devices transfer heat gradients back to the input differential pair. \$\endgroup\$
    – analogsystemsrf
    Commented Oct 13, 2019 at 21:08

1 Answer 1

Reset to default
1
\$\begingroup\$

We're clearly not talking about ideal opamps here, which have infinite gain and infinite bandwidth, among other properties.

The G-BW product is a constant for any real opamp that has a dominant pole, and it only applies above the frequency associated with that pole.

Below that frequency, the opamp has some finite maximum gain dictated by the limitations of the devices used in its internal circuitry.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.