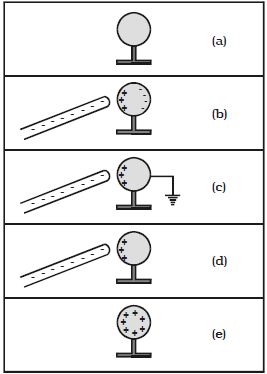
In the given figure, if we connect the positively induced side of the sphere to the earthing instead of connecting it to the negatively induced side, then will the sphere get negatively charged instead of getting positively charged?
3 Answers
(b) The diagram demonstrates that charges can move from one side of the sphere to the other. That indicates that the sphere is conductive.
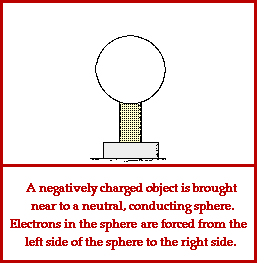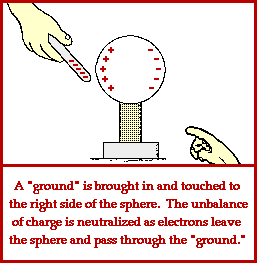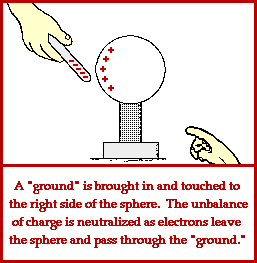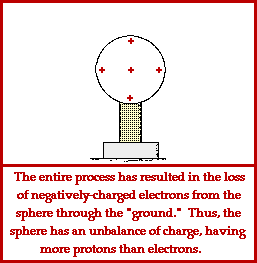
(c) When the sphere is connected to earth. Some negative charges flow to earth.
(d) If the earth connection is then removed, it is left with a positive charge.
(e) When the negatively charged rod is removed the charges re-distribute themselves evenly in the sphere, but the sphere remains charged because it has lost some negative charge to earth.
(f) If the sphere is re-connected to earth, the negative charges will redistribute themselves to include the earth. That results in negative charges flowing from earth and mostly restore the sphere to the un-charged condition, but will not make the sphere positively charged.
The fact that the sphere is conductive means that the point of earth attachment makes no difference. If there is any connection from the sphere to earth, the sphere can not be charged. Even a poor connection like humid air will tend to balance the charge of the sphere.
-
\$\begingroup\$ Moved comment ti answer \$\endgroup\$– user80875Commented Jan 25, 2022 at 16:04
If the slides represent a dynamic sequence as illustrated here:
https://www.physicsclassroom.com/mmedia/estatics/isop.cfm
Then I think the point where ground touches the sphere does not matter for the net result, that is, the sphere will take a positive charge when the ground connection is removed. The location of the ground connection is relevant for the charge distribution when ground is connected but not relevant to the net result when ground is removed.
-
\$\begingroup\$ For conductive objects, the location of the ground connection is only relevant in an extremely slow motion dramatization. In realistically measured time, redistribution of charge is instantaneous. \$\endgroup\$– user80875Commented Jan 25, 2022 at 16:35
-
1\$\begingroup\$ My understanding of the question is that the ground shown in (c) would be touching the positively induced side of the sphere. Then would the sphere become negatively charged (when ground is removed)? The answer is no. The outcome would be the same regardless of where the ground is connected to the sphere. However the distribution of charge when the ground is connected might depend on the location of the wand and ground even if the charge relaxation time is so small as to be effectively instantaneous. \$\endgroup\$ Commented Jan 25, 2022 at 17:47
-
\$\begingroup\$ How electrostatic induction is explained in terms of potential? \$\endgroup\$ Commented Feb 14, 2022 at 7:14
-
\$\begingroup\$ Potential is the measure of work required to move a positive test charge from one point to another point in the presence of an external electric field. Any net positive or negative charge generates an electric field. The zero potential is sometimes defined at infinity for net charge isolated in empty space. Ground is set to zero potential for an actual system with a large source or sink of electric charge in the surroundings of the net charge system. \$\endgroup\$ Commented Feb 14, 2022 at 16:16
No, when you connect the sphere to earth, the effective conductor now includes the whole of earth.
The induced charges in a neutral sphere (at (5.5,0,0) with radius 9.5) in a non-uniform external electric field coming from a point charge at (-15,0,0) will have the following potential on the z=0 plane:

The sphere has 0V because the net induced charge on the sphere is 0V plus the initial net charge of the sphere (0V because it is a neutral sphere) so the 0V contour coincides with the centre of the sphere.
When you now superimpose that with the potential due to the external point charge:


The sphere is equipotential but not quite equipotential in the diagram but this is only due to the error of the simulation as there are only 1000 surface charges to improve computation speed and it is also difficult to get them uniformly spaced (Thomson problem).
It can be seen though that the potential from the induced charges at any given point on the sphere exactly cancels the potential difference between a point at the centre of the sphere in the external field and the and the given point, meaning that the entire sphere becomes the potential of the point at the centre of the sphere in the external electric field, this appears to be around 3V in this case.
The sphere has a potential of 3V from the centre to infinity in the presence of the point charge but as soon as the external field from the point charge is taken away, it returns to 0V. The only way to keep |3V| on the sphere is to ground the sphere, here's why.
When you ground the sphere, it makes the conductor the size of earth plus the size of the conductor and the wire connecting them. Let's just model this as one huge sphere. We can therefore assume that the potential at the centre of this huge conductor due to the point charge is effectively zero (as opposed to 3V of before) because it is so far away. We also know that the charges induced on the huge neutral conductor (neutral sphere + neutral earth) exactly cancel out the potential so that the huge conductor is equipotential with the centre of the huge conductor, this means that the original sphere becomes 0V (so 3V less than before it was grounded), therefore the sphere itself has a net voltage of -3V but is 0V in the field. Disconnecting ground causes the sphere to remain at -3V (still 0V in the presence of the external charge and then removing the external charge reveals the -3V.