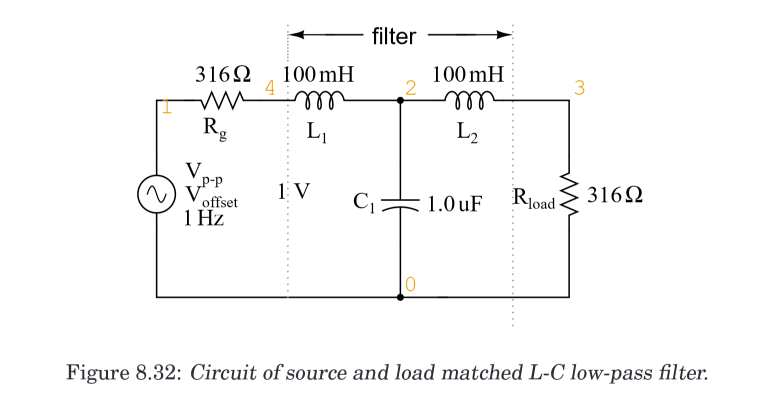
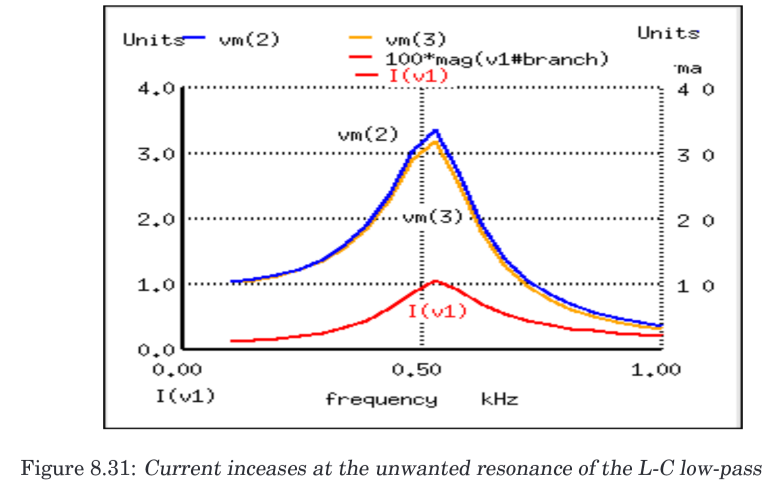
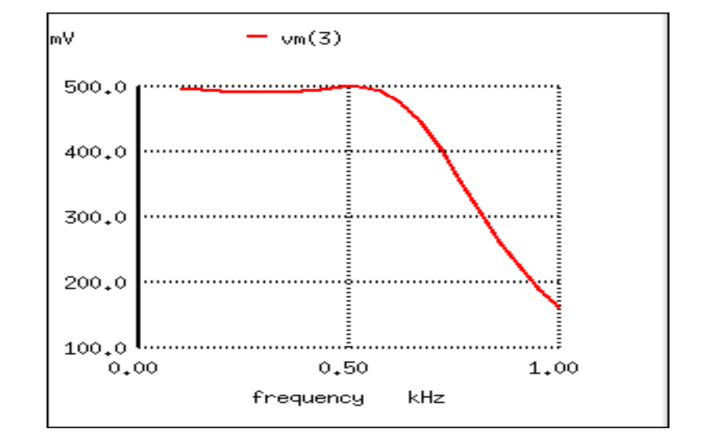
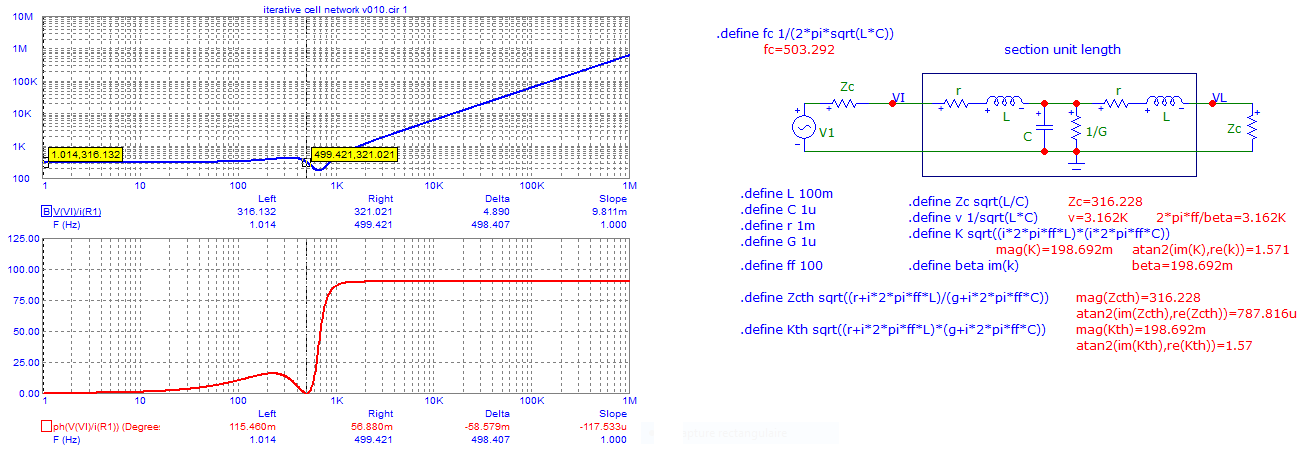
One impedance value doesn't describe the impedance of the filter at all frequencies. It's quite easy to see that at high frequencies, the input impedance of the inductor will be very high.
This filter has been designed to work between those defined impedances, and should be operated between those impedances to obtain its design performance.
This filter will present a reasonably constant input impedance over its passband, and then will deviate from that more and more into the stopband. An inductor input low pass filter as you've drawn will go high impedance at high frequency. A capacitor input lowpass filter will become a short circuit at high frequency.
That's how filters are designed with modern network theory. You define a finite resistive impedance on at least one, preferrably both ports of a filter. When only one port has a finite impedance, the other is allowed to be short or open circuit. Usually, both ports have the same impedance, but they can be different to make an impedance 'transformer' for reasonable ratios of impedance. Once you have the defined impedances, you select reactive components to work with those to produce your required response. The corollary of this is that if your filter has been designed for one impedance, and you operate it with different impedances on the ports, then it won't produce its design performance.
It is possible to make a filter keep its impedance reasonably constant at all frequencies. This is called 'diplexing'. Two filters, one highpass and one lowpass, each with a series input element, are connected inputs in parallel, with outputs one going to the required output, the other to a load. The input signal 'sees' the impedance on either filter output depending on frequency. This is often done when filtering a mixer port, mixers can misbehave if their ports are connected to loads with a poor impedance match. There's a little subtlety designing these filters, their connected input ports need to be designed into a short circuit, not the desired impedance, as the input voltage stays constant (just like a low impedance would) as the frequency is varied.