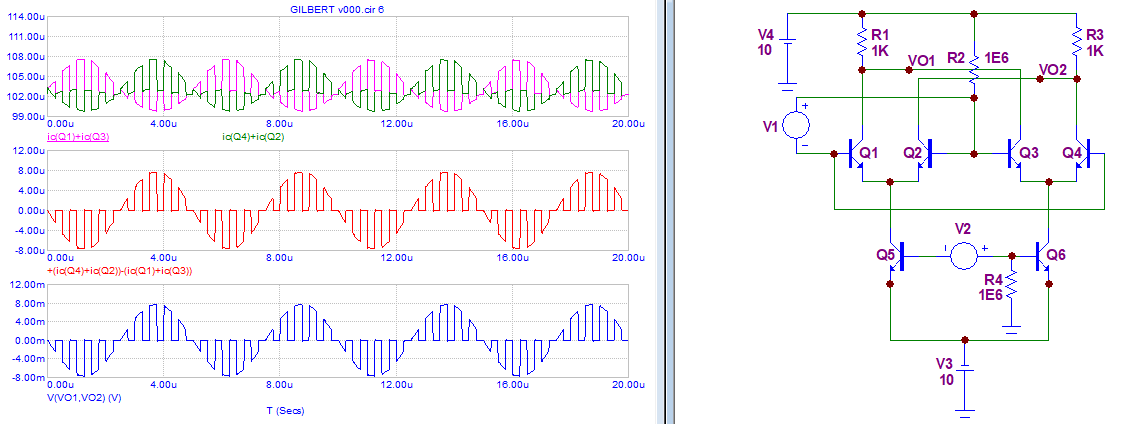
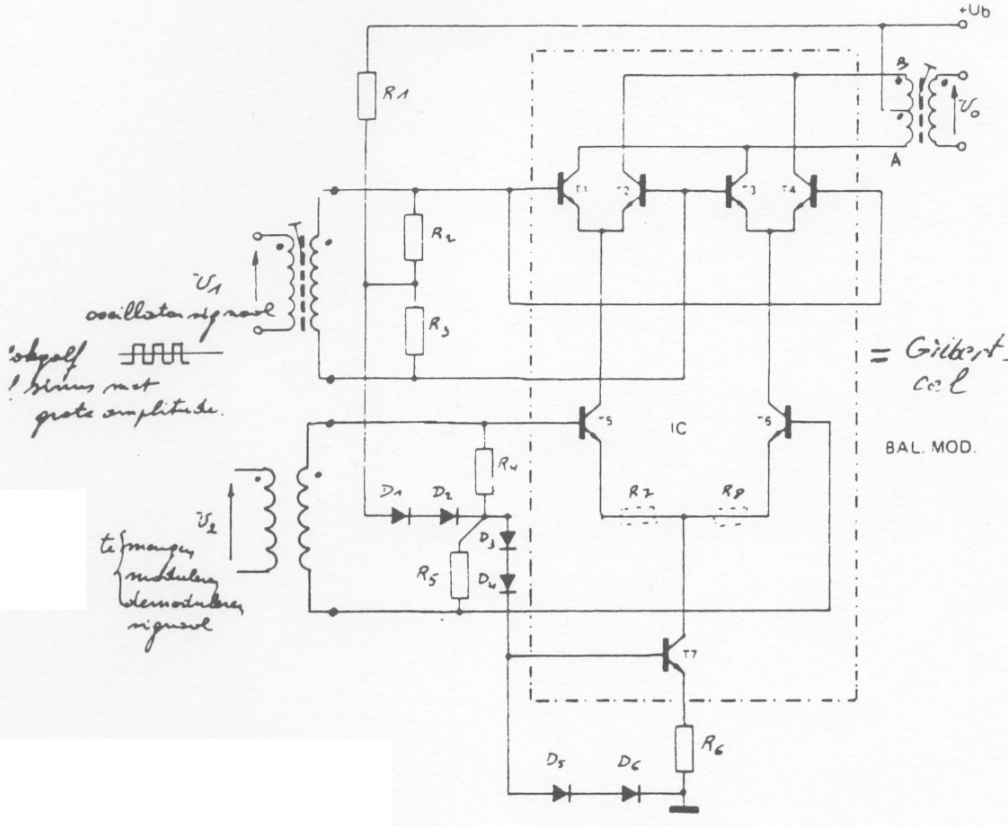
I'm currently breaking my head over this Gilbert cell:
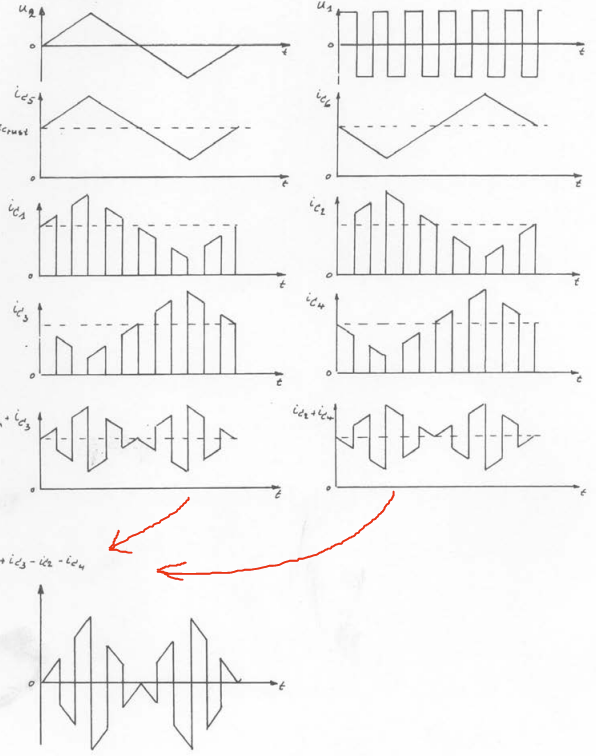
I understand the workings of this circuit, that is not the issue I'm having. What I do not understand is, when looking at the current waveforms:
How do you get this resulting output? I understand it all the way to where the red arrows are drawn, but not how you get this result from combining those 2, where is the DC current offset?
How do you get this resulting output? I understand it all the way to where the red arrows are drawn, but not how you get this result from combining those 2,
A - B is the special thing here. Signal A minus signal B.
The circuit produces an AC part of A that is \$\color{red}{\text{antiphase}}\$ (negative in polarity) to the AC part of B so, if those AC parts each have an magnitude of (say) "1", when we do "A - B", we get an AC output of "2". In other words \$A_{AC} - B_{AC} = \color{blue}{1 - (- 1)} = \color{orange}{1+1 = 2}\$.
But, the DC parts of A and B are near-enough identical hence, when we do A - B we get near-zero DC output.
where is the DC current offset?
It is now zero due to subtraction i.e. A minus B.
EE&O. For information, an interesting "specification" of the Gilbert cell to be pointed out is the fact that one can get rid of the inherent "distortion" of the "simpler" system.
Here is explained by a picture: "distortion" about current through R1 and R2.
