Using tunnel diode to cancel out armature resistance

Question

Can we use tunnel diodes to cancel out armature resistance?

A tunnel diode when it is in its negative differential region under DC shifted AC(AC with some DC offset) and if the Vp-p of the AC is very small behaves like a resistor with negative resistance.

Usually the absolute value of this negative resistance is really small ~ 1Ω so we cant use it to cancel out the resistance of lets say a lamp BUT the armature resistance of a AC motor is in the range of 1Ω so in that way:

^{simulate this circuit – Schematic created using CircuitLab}

Since \$I\$ would become very big , the motor would rotate very very fast so I suspect it would reach saturation.Am I correct?

Answer 1

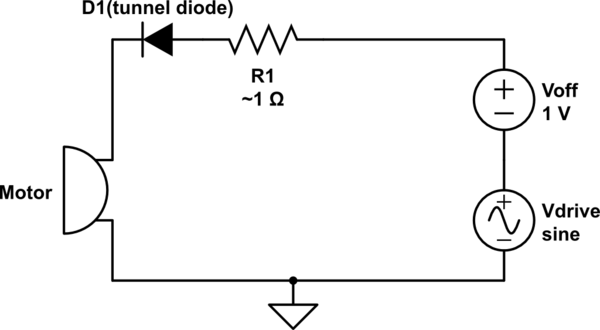
Revealing the secret of negative differential resistance by CircuitLab experiments

Experimental setup

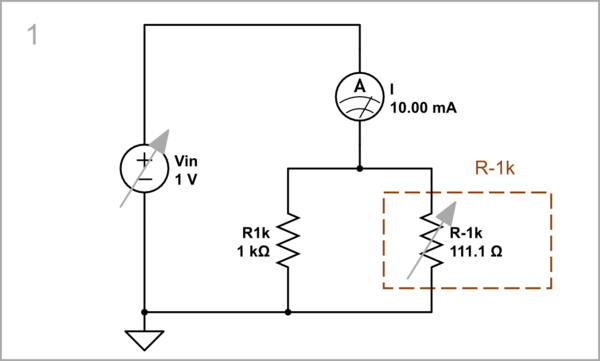
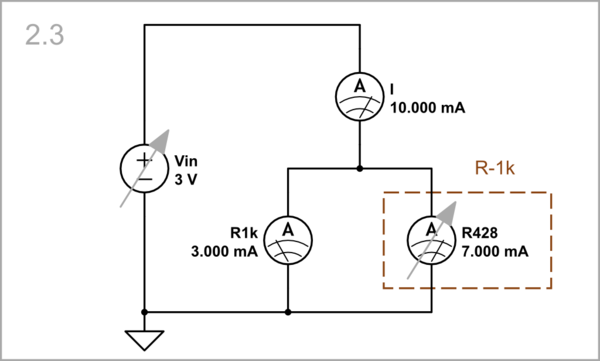
According to my comments above, two resistors are connected in parallel - R1k with a 1 kΩ "positive" resistance, and R-1k with a 1 kΩ "negative" resistance. I have put both names in square quotes because these resistances are neither negative nor positive, but the most common ohmic resistance known since the 19th century. So the negative differential resistance of -1 kΩ is implemented by a variable resistor whose resistance changes from 111 Ω to infinity when Vin varies from 1 V to 10 V.

^{simulate this circuit – Schematic created using CircuitLab}

We need to observe the currents flowing through the resistors. To simplify the schematics, we can combine the resistors with the ammeters into one device ("visualized resistor"). To do this, open the parameters window of each of the two ammeters and set the corresponding internal resistance.

Step-by-step experiments

To understand the mechanism of this so-called "N-shaped negative differential resistance", let's first examine the circuit at three successive values ​​of the input voltage - 1, 2 and 3 V.

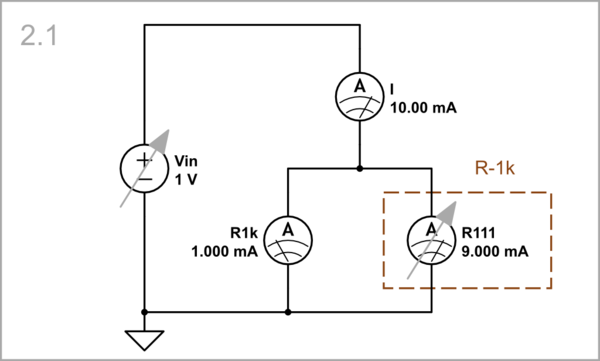
Vin = 1 V, R-1k = 100 Ω: At 1 V input voltage, the 1 k positive resistor consumes 1 mA. The negative resistor has a 111 Ω resistance, so 9 mA current flows through it, and the total current consumed is 10 mA.

^{simulate this circuit}

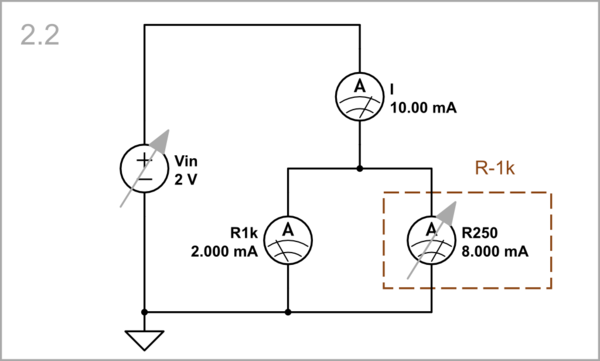
Vin = 2 V, R-1k = 222 Ω. When the input voltage increases to 2 V, the negative resistor increases its ohmic resistance to 250 Ω. Now the positive resistor consumes 2 mA but the negative 8 mA, and the total current consumed is again 10 mA (i.e., the left current increases but the right current decreases, and their sum I remains constant).

^{simulate this circuit}

Vin = 3 V, R-1k = 375 Ω: Next, the input voltage increases to 3 V, and the negative resistor increases its ohmic resistance to 428 Ω. The positive resistor consumes 3 mA but the negative 7 mA, and the total current consumed is, as usual, 10 mA... and so on...

^{simulate this circuit}

Automated experiment

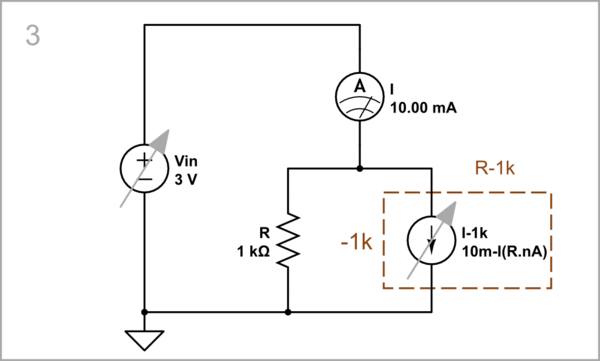
To sweep the negative resistance, we can simulate it using a behavioral current source I-1k that produces a current 10 mA - IR.

^{simulate this circuit}

Graphical representation

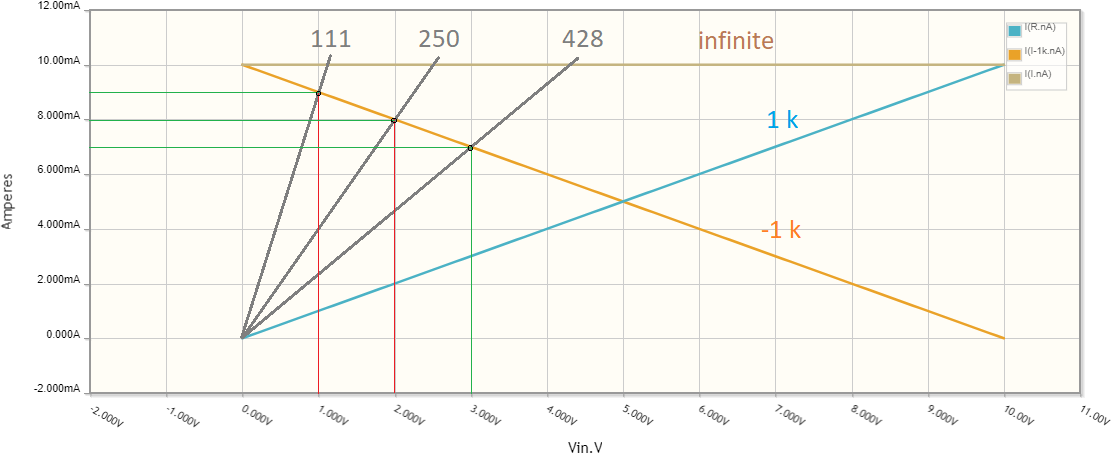
From the graphs below, we see that as the input voltage increases, the current through the positive resistor increases and through the negative resistor decreases because its resistance increases. The result is a constant common current which is seen by the input voltage source as an infinite differential resistance.

Applications

I will illustrate my conceptual explanations above by solving a specific circuit problem - eliminating the impact of the load on the output voltage of a voltage divider.

Experimental setup

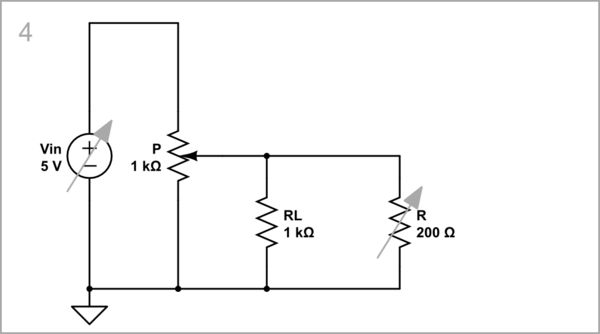
In the schematic below, a potentiometer P is loaded by a 1 kΩ "positive" resistor RL. A 1 kΩ (N-shaped) negative differential resistor R with initial resistance of 200 Ω is connected in parallel.

^{simulate this circuit}

Vin = 5 V, R = 200 Ω: As a result, a total current I = 6 mA is consimed from the potentiometer output, and its output voltage is 1 V. Note that to simplify the schematic, I have implemented the resistors with "bad" ammeters having the same internal resistance (RL1k = 1 kΩ, R = 200 Ω).

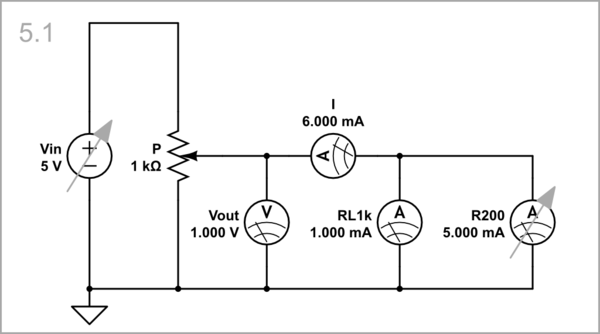
^{simulate this circuit}

Vin = 6 V, R = 333 Ω: When we increase Vin with 1 V, the potentiometer output voltage begins increasing. Simultaneously, the "negative" resistor R increases its resistance to 333 Ω thus compensating the influence of the positive load.

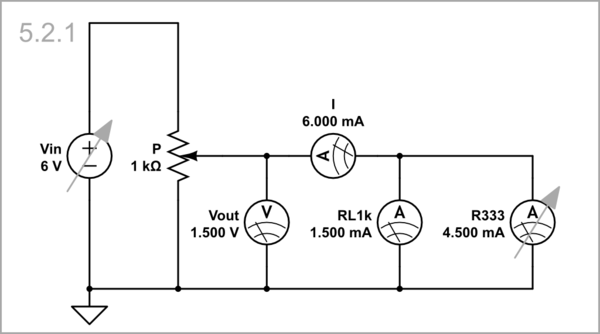
^{simulate this circuit}

As a result, the output voltage becomes 1.5 V instead of 1.2 V as it would be if R had not increased:

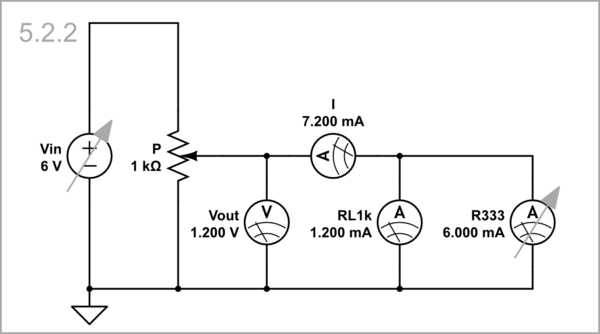
^{simulate this circuit}

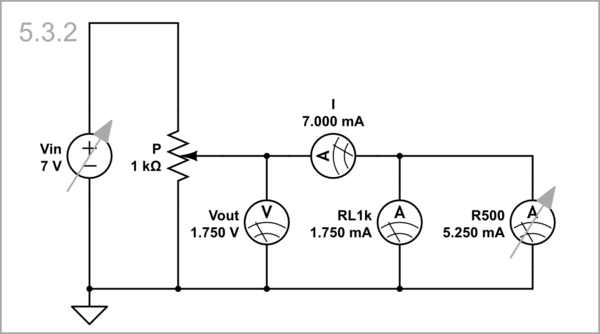
Vin = 7 V, R = 500 Ω: When we increase Vin by another 1 V, the potentiometer output voltage begins increasing even more. The "negative" resistor R increases its resistance to 500 Ω thus compensating the influence of the positive load.

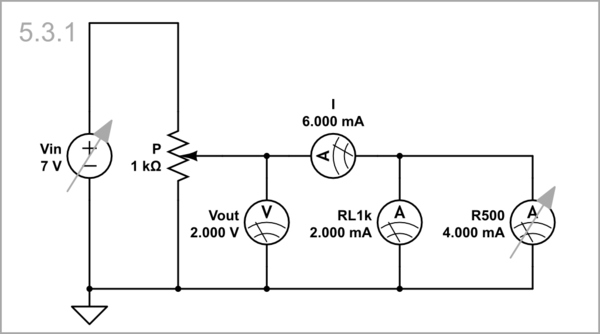
^{simulate this circuit}

The output voltage becomes 2 V instead of 1.75 V as it would be if R had not increased:

^{simulate this circuit}

Equivalent circuit

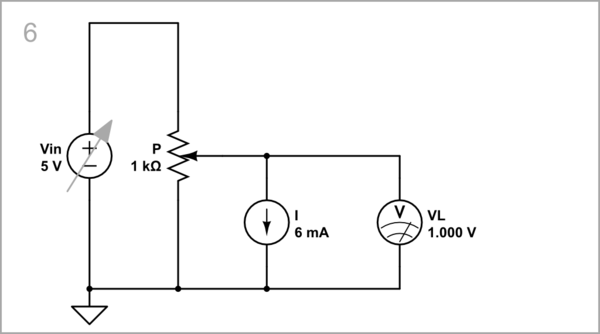
So, the voltage divider is loaded with a 6 mA constant current source I, and the output voltage variations are not influenced by this extremely high-resistance load.

^{simulate this circuit}

Conclusions

• The N-shaped negative differential resistor (e.g. a tunnel diode) is a dynamic resistor that increases its static (ohmic) resistance when the voltage across it increases.

• If connected in parallel to an equivalent "positive" resistor, it neutralizes its resistance so that the equivalent resistance is infinite.

So this is not what OP need. They need:

• a dynamic resistor that decreases its static (ohmic) resistance when the current through it increases.

• It will be connected in series to an equivalent "positive" resistor so that to neutralize its resistance, and make the equivalent resistance zero.

See also another story of mine dedicated to this circuit phenomenon.

Answer 2

No you can't do that since it does not have absolute negative resistance.

We do use IR compensation in motor speed controls to negate armature resistance though. It's done with an electronic circuit, basically to measure the armature current and increase the voltage to compensate for the I*R drop- no weird components like Esaki diodes are required.

Ideally, it means the motor will maintain a constant speed proportional to applied voltage regardless of mechanical loading. Without such "IR compensation" the motor speed will drop as the load and therefore armature current increases.