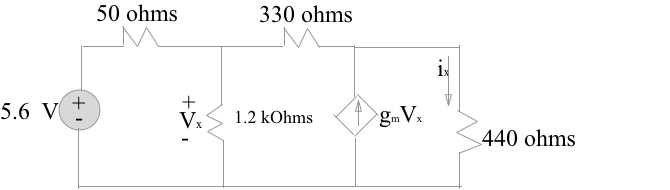
We need to find the current ix where Gm = 0.2 S. What I've done so far if created two equations using node voltage.
Eq. 1 : Vx/330 - 440ix/330 -ix +gmVx = 0
Eq. 2 : 50Vx/1200 + 50ix - 50gmVx + Vx = 5.6
Matrix (Ax=B) looks like this:
A = (1/330)+.2 (-440/330) - 1
(1/24)-50(.2)+1 50
x = Vx
ix
B = 0
5.6
Did I do this correctly? If not, what should I do?