Common collector effect of emitter resistor on output impedance

Question

I've been trying to figure out why the expression for output impedance in a common collector configuration contains a parallel Re emitter resistor. Looking at the classic circuit, it looks as though the Re resistor is not part of the seen output impedance (similar to the way it isn't in a common emitter configuration)

I'm a rather elderly person who has decided to take on myself to self-study analog electronics - so I would appreciate if someone more experienced and knowledgeable could enlighten me about this issue.

Thanks, Sefer.

Answer 1

Inside the BJT transistor (and hidden from view) is a resistor called \$r_E\$. Sometimes it is called \$r_{\pi}\$ just to confuse everybody but I was brought up with \$r_E\$ so I'll refer to it as that. I'll refer to the external emitter resistor as \$R_E\$. This is what \$r_E\$ looks like in the bigger picture: -

The value of \$r_E\$ is inversely proportional to the current flowing from the emitter (largely the current flowing into the collector) and, proportional to a value called \$V_T\$ and \$V_T\$ is 26 mV at room temperature.

So, \$r_E\$ is about 26 ohm per mA of emitter current. If you want more evidence of this see the extract I took from here regarding what \$V_T\$ is. I've put this under the main body of my answer at the bottom of the page.

In a common-collector amplifier, the "imaginery emitter" would follow the base signal but the real emitter voltage would be the imaginery emitter voltage minus the loading effect of \$R_E\$ on \$r_E\$. In other words these two resistors form a potential divider on the "imaginary emitter".

And this is why the output from a common collector (emitter follower) BJT circuit contains both resistor values in the formula. It's also why the output impedance of a common emitter is loosely referred to as \$r_E\$ but is more accurately referred to as $$r_E || R_E$$.

---

Where \$V_T\$ comes from: -

Answer 2

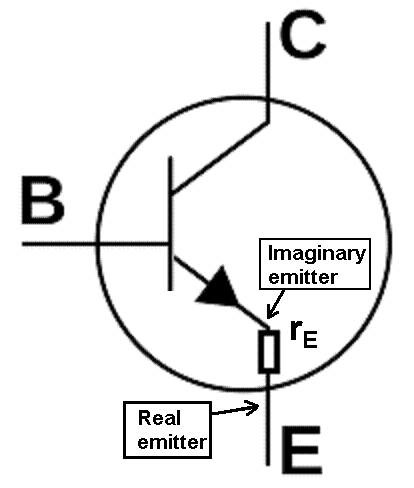
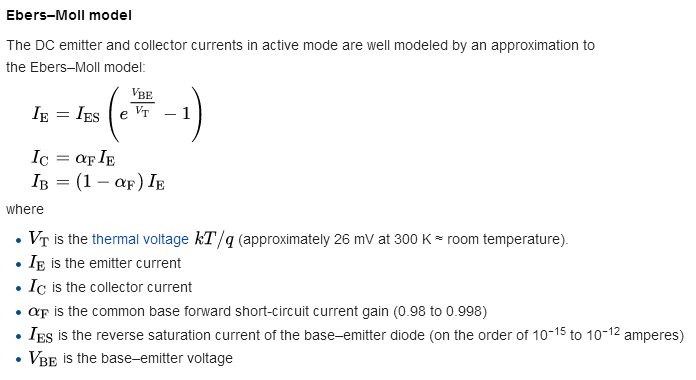
The simplest common collector amplifier looks like this:

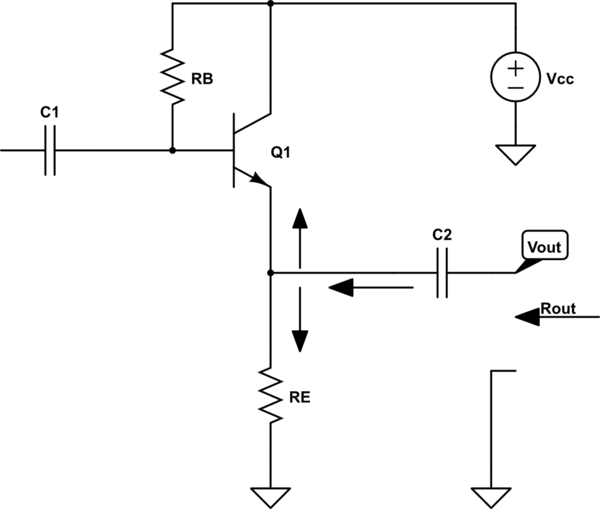
^{simulate this circuit – Schematic created using CircuitLab}

And now if we are looking form the load resistance perspective. We see two paths for a current to flow.

One path is through the \$R_E\$ resistor into GND.

And the second path is into the transistor emitter terminal.

And this is why the load is seeing two "resistances" in parallel.

\$R_{OUT} = R_E||(r_e + \frac{R_B}{\beta+1})\$

Where \$r_e = \frac{V_T}{I_E} \approx \frac{26mV}{I_E}\$

http://users.physics.harvard.edu/~horowitz/aoe/sm/smlitlre.htm

how to derive the ac emitter resistance of transistor amplifier biasing?

Or you can see it this way. The NPN transistor can only "source" the current into the load resistance. And \$R_E\$ resistor can "sink" the load current.

EDIT

For the CE amplifier with emitter degeneration resistor (\$R_E\$), we have this situation:

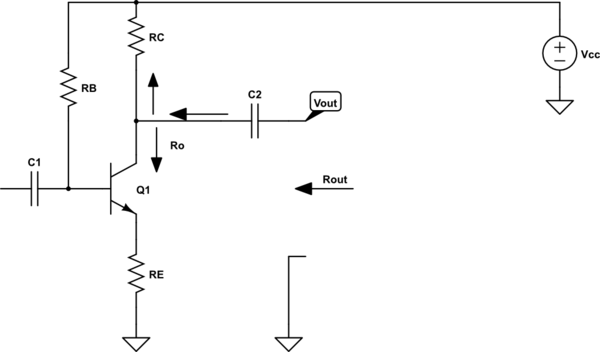
^{simulate this circuit}

And again we have two paths for a current.

But this time the resistance seen from the transistor collector into the transistor is very large and include the \$R_E\$ resistor.

\$\large R_O \approx r_o*(1+\frac{\beta*R_E}{(\beta+1)*r_e+R_B+R_E})\$

\$r_o\$ - https://en.wikipedia.org/wiki/Early_effect#Small-signal_model

So in practice, we write this like this:

\$R_{OUT} = R_C||R_O\$

And because \$R_C << R_O \$

\$ R_O = R_C||R_O \approx R_C\$