I was asked to find the Is current
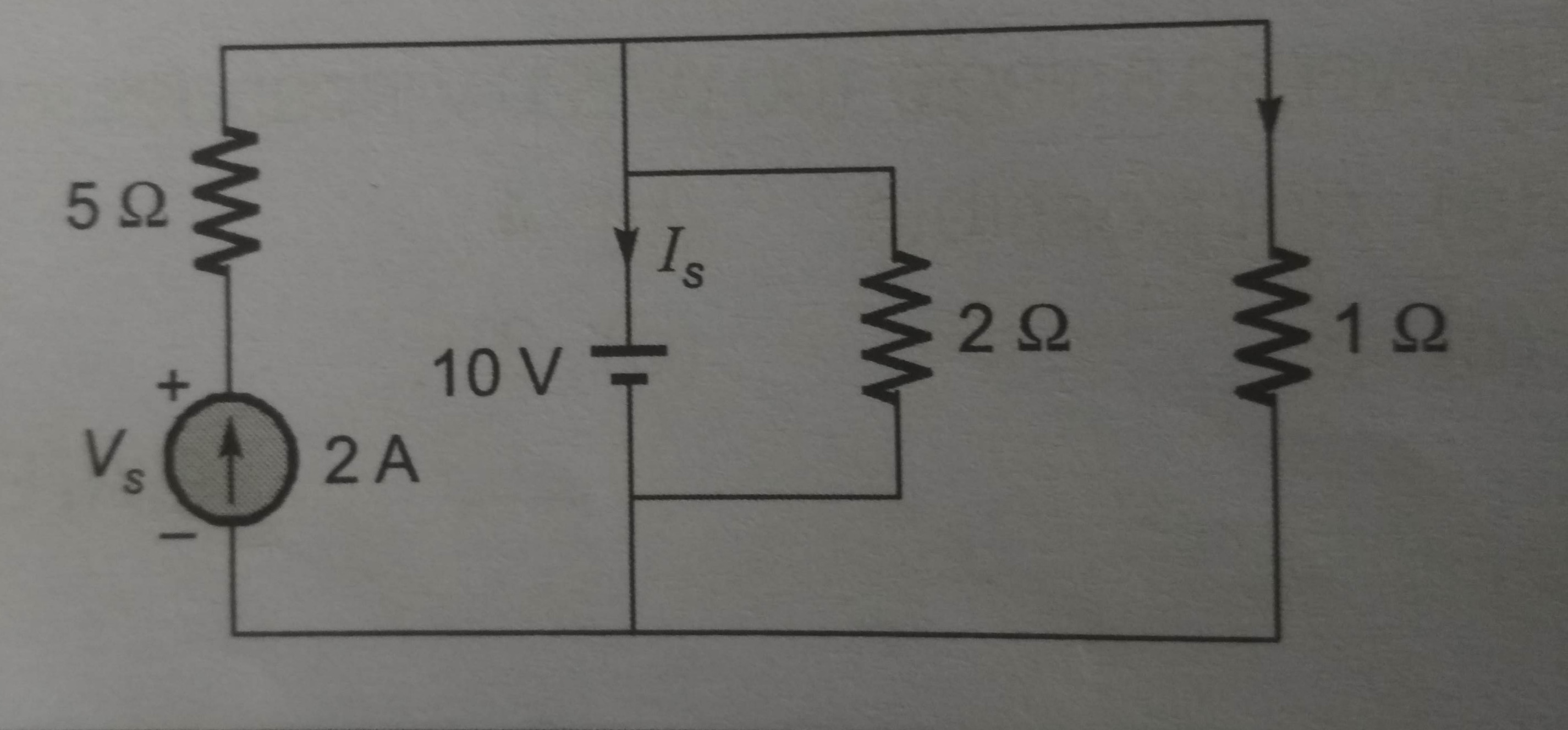
I applied KVL in that loop but my ans is wrong.
what concept is wrong on my approach?Why kvl cant be applied on that loop please explain
\$\begingroup\$
\$\endgroup\$
9
-
2\$\begingroup\$ Do you have to use KVL? I think the current law makes more sense here. You know the 2A already. There's 10V across BOTH resistors (don't be confused by how the circuit is drawn). Since they're both 10V, you can calculate the currents in them easily. Then use KCL to find "IS" \$\endgroup\$– Kyle BCommented Mar 19, 2021 at 4:01
-
3\$\begingroup\$ You did not actually apply KVL. You applied a weird and incomplete mix of KCL and KVL. You have 3 loops, yet only one loop equation. You can't just solve a loop in isolation because everything present affects everything else. You solve for it all. \$\endgroup\$– DKNguyenCommented Mar 19, 2021 at 4:02
-
3\$\begingroup\$ KVL is valid always. What you did here isn't KVL. \$\endgroup\$– HearthCommented Mar 19, 2021 at 4:03
-
3\$\begingroup\$ And there is also a hole in your logic for the KCL you tried to apply at the top center node. You wrote that the current flowing out to the right is 2-Is, but it's not, because the current flowing down is not Is. The current flowing through the battery and only the battery is Is. The current flowing down is a combination of Is and that through the 2 Ohm resistor. \$\endgroup\$– DKNguyenCommented Mar 19, 2021 at 4:08
-
2\$\begingroup\$ KVL holds. There is 10V across the 1\$\Omega\$ resistor, and 10V across the 2\$\Omega\$ resistor. \$\endgroup\$– Math Keeps Me BusyCommented Mar 19, 2021 at 4:10
|
Show 4 more comments
1 Answer
\$\begingroup\$
\$\endgroup\$
0
Because the current through \$ 1\Omega\$ is not \$2-I_s\$ as you have assumed. It is actually = \$2-(I_s+I_{2\Omega})\$, where \$I_{2\Omega} = 10/2 = 5A\$. You didn't see that there are actually 4 branches at the upper node where you split the current using KCL. Not 3 branches. \$2\Omega\$ is the branch you missed. I think \$2\Omega\$ was deliberately drawn like that to trick students.
