Why does the difference amplifier formula not work in this case?

Question

I calculated Vo for this ideal op amp circuit using the formula: \$R_2\$/\$R_1\$(\$V_2\$-\$V_1\$) = \$V_o\$
I believe the correct \$V_o\$ = -14V I did not get this result using the above formula. It seems the formula is not correct for this circuit but I don't understand why. Would someone please enlighten me?

^{simulate this circuit – Schematic created using CircuitLab}

Answer 1

It seems the formula is not correct for this circuit but I don't understand why

That's not a differential amplifier.

To solve that circuit you can proceed in at least two ways (assuming ideal op-amp). Here are a few hints (you won't get the full solution):

1. Superposition. Null the two independent sources \$V_1\$ and \$V_2\$ one at a time, and then sum the two partial responses. By nulling \$V_2\$ you're left with an inverting amplifier; by nulling \$V_1\$, with a non-inverting amplifier.
2. Impose ideal op-amp conditions. Recall that an ideal op amp, when there is a feedback path from the output to the input, adjusts its output to have $$v_\mathrm{n}=v_\mathrm{p},$$ where \$v_\mathrm{n}\$ is the potential of the inverting input and \$v_\mathrm{p}\$ that of the non-inverting one. In your circuit, this implies \$v_\mathrm{n} = V_2\$. Knowing this, you can easily apply Millman's theorem at the inverting input (or Kirchhoff's laws, if you feel more comfortable), considering the circuit containing the sources \$V_1\$ and \$v_\mathrm{o}\$. Then solve for \$v_\mathrm{o}\$.

Answer 2

^{simulate this circuit – Schematic created using CircuitLab}

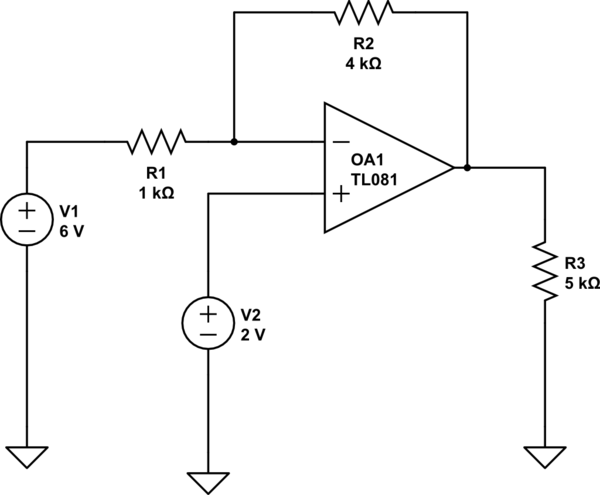
Figure 1. Inverting op-amp with offset on non-inverting input.

• The trick here is to remember that the op-amp doesn't know where your GROUND or 0 V is. This should be obvious from the schematic as there is no 0 V connection to the op-amp.
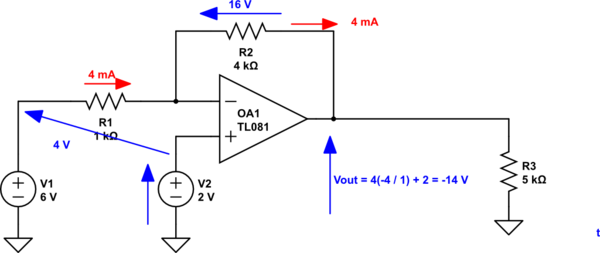
• In the inverting amplifier configuration the op-amp will try to adjust the output until the inverting input voltage is the same as the non-inverting input. This will happen when both are at +2 V above ground and your effective input is 4 V relative to the non-inverting input. -The gain of the circuit will be \$-\frac {R_F}{R_I} = -4\$ relative to the non-inverting input so

$$ V_{OUT} = -(V_1 - V_2)\frac{R_F}{R_I} + V_2 = (6-2)\frac{-4}{1} + 2 = -14 V$$

I did not get this result using the above formula.

In a practical experiment you might not get 14 V out. If this is the case you are probably driving the op-amp too close to the negative power rail. You have to either increase the negative power-rail voltage (watch the specifications) or decrease your 6 V input signal.

Answer 3

From TI/NSC:

Or...

1. because of the way opamps work, the voltage on OA1- must be equal to, and the same sign as, the voltage on OA1+
2. If OA1+ is at +2V, then OA1- must also be at +2V.
3. If OA1- is at +2V then R1 has 4 volts across it.
4. If R1 has 4V across it then the current through it must be \$ I =\frac{4V}{1000\Omega} = 0.004 A \$
5. Since R1 and R2 are in series, the current through R2 is the same current through R1.
6. Since R2 is 4000 ohms and there's 0.004 A through it, it must be dropping \$ 0/004A \times 4000 ohms = 16 \text{ volts.}\$
7. Since OA1 is an inverting amplifier and OA1- is positive, OA1 OUT must be negative.
8. Since the voltage on the left-hand side of R1 is +2 volts, the total drop across R2 is 16 volts, and OA1 OUT must be negative, OA1 OUT must be at -14 volts.
9. R3 doesn't matter since it's not in the loop.